


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 3-9-2020
Date: 11-11-2020
Date: 16-10-2019
|
A rational number is a number that can be expressed as a fraction  where
where 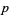 and
and 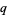 are integers and
are integers and  . A rational number
. A rational number  is said to have numerator
is said to have numerator 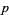 and denominator
and denominator 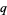 . Numbers that are not rational are called irrational numbers. The real line consists of the union of the rational and irrational numbers. The set of rational numbers is of measure zero on the real line, so it is "small" compared to the irrationals and the continuum.
. Numbers that are not rational are called irrational numbers. The real line consists of the union of the rational and irrational numbers. The set of rational numbers is of measure zero on the real line, so it is "small" compared to the irrationals and the continuum.
The set of all rational numbers is referred to as the "rationals," and forms a field that is denoted 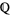 . Here, the symbol
. Here, the symbol  derives from the German word Quotient, which can be translated as "ratio," and first appeared in Bourbaki's Algèbre (reprinted as Bourbaki 1998, p. 671).
derives from the German word Quotient, which can be translated as "ratio," and first appeared in Bourbaki's Algèbre (reprinted as Bourbaki 1998, p. 671).
Any rational number is trivially also an algebraic number.
Examples of rational numbers include 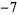 , 0, 1, 1/2, 22/7, 12345/67, and so on. Farey sequences provide a way of systematically enumerating all rational numbers.
, 0, 1, 1/2, 22/7, 12345/67, and so on. Farey sequences provide a way of systematically enumerating all rational numbers.
The set of rational numbers is denoted Rationals in the Wolfram Language, and a number  can be tested to see if it is rational using the command Element[x, Rationals].
can be tested to see if it is rational using the command Element[x, Rationals].
The elementary algebraic operations for combining rational numbers are exactly the same as for combining fractions.
It is always possible to find another rational number between any two members of the set of rationals. Therefore, rather counterintuitively, the rational numbers are a continuous set, but at the same time countable.
For 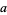 ,
, 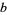 , and
, and 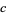 any different rational numbers, then
any different rational numbers, then
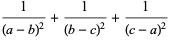 |
is the square of the rational number
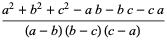 |
(Honsberger 1991).
The probability that a random rational number has an even denominator is 1/3 (Salamin and Gosper 1972).
It is conjectured that if there exists a real number  for which both
for which both 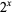 and
and 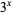 are integers, then
are integers, then  is rational. This result would follow from the four exponentials conjecture (Finch 2003).
is rational. This result would follow from the four exponentials conjecture (Finch 2003).
REFERENCES:
Bourbaki, N. Éléments de mathématique: Algèbre. Reprinted as Elements of Mathematics: Algebra I, Chapters 1-3. Berlin: Springer-Verlag, 1998.
Courant, R. and Robbins, H. "The Rational Numbers." §2.1 in What Is Mathematics?: An Elementary Approach to Ideas and Methods, 2nd ed. Oxford, England: Oxford University Press, pp. 52-58, 1996.
Finch, S. R. "Powers of 3/2 Modulo One." §2.30.1 in Mathematical Constants. Cambridge, England: Cambridge University Press, pp. 194-199, 2003.
Honsberger, R. More Mathematical Morsels. Washington, DC: Math. Assoc. Amer., pp. 52-53, 1991.
Salamin, E. and Gosper, R. W. Item 54 in Beeler, M.; Gosper, R. W.; and Schroeppel, R. HAKMEM. Cambridge, MA: MIT Artificial Intelligence Laboratory, Memo AIM-239, p. 18, Feb. 1972. https://www.inwap.com/pdp10/hbaker/hakmem/number.html#item54.
Wolfram, S. A New Kind of Science. Champaign, IL: Wolfram Media, p. 1168, 2002.



|
|
|
|
تفوقت في الاختبار على الجميع.. فاكهة "خارقة" في عالم التغذية
|
|
|
|
|
|
|
أمين عام أوبك: النفط الخام والغاز الطبيعي "هبة من الله"
|
|
|
|
|
|
|
قسم شؤون المعارف ينظم دورة عن آليات عمل الفهارس الفنية للموسوعات والكتب لملاكاته
|
|
|