


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 23-5-2021
Date: 29-5-2021
Date: 31-5-2021
|
A codimension one foliation 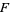 of a 3-manifold
of a 3-manifold 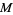 is said to be taut if for every leaf
is said to be taut if for every leaf 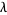 in the leaf space
in the leaf space  of
of 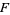 , there is a circle
, there is a circle 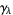 transverse to
transverse to 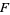 (i.e., a closed loop transverse to the tangent field of
(i.e., a closed loop transverse to the tangent field of 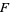 ) which intersects
) which intersects 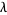 .
.
Taut foliations play a significant role in various aspects of topology and are credited as being one of two major tools (along with incompressible surfaces) responsible for revealing significant topological and geometric information about 3-manifolds (Gabai and Oertel 1989). As such, a considerable amount of research has gone into the study of taut foliations on 3-manifolds. One well-known result is that every taut foliation is necessarily Reebless and that, for any non-taut Reebless foliation, the leaves which don't admit a closed transversal are necessarily tori. Additionally, the closed leaves of a taut foliation are homologically nontrivial.
Some classification results for taut foliations are also known. One such result, attributed by Eliashberg and Thurston to Novikov and Sullivan, says that a foliation 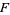 on a closed 3-manifold
on a closed 3-manifold 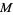 is taut if it is different from the foliation
is taut if it is different from the foliation 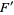 on
on  and satisfies any one of the following:
and satisfies any one of the following:
1. Each leaf 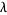 of
of 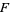 is intersected by a transversal closed curve.
is intersected by a transversal closed curve.
2. There exists a vector field 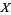 on
on  which is transversal to
which is transversal to 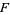 and preserves a volume form
and preserves a volume form 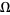 on
on  .
.
3. 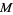 admits a Riemannian metric for which all leaves are minimal surfaces.
admits a Riemannian metric for which all leaves are minimal surfaces.
Moreover, a necessary and sufficient condition for tautness of a foliation 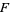 is that
is that 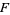 contain no generalized Reeb components (Goodman 1975).
contain no generalized Reeb components (Goodman 1975).
REFERENCES:
Calegari, D. Foliations and the Geometry of 3-Manifolds. Oxford, England: Clarendon Press, 2007.
Eliashberg, Y. M. and Thurston, W. P. Confoliations. Providence, RI: Amer. Math. Soc., 1998.
Gabai, D. and Oertel, U. "Essential Laminations in 3-Manifolds." Ann. Math. 130, 41-73, 1989.
Goodman, S. "Closed Leaves in Foliations of Codimension One." Comm. Math. Helv. 50, 383-388, 1975.



|
|
|
|
علامات بسيطة في جسدك قد تنذر بمرض "قاتل"
|
|
|
|
|
|
|
أول صور ثلاثية الأبعاد للغدة الزعترية البشرية
|
|
|
|
|
|
|
مستشفى العتبة العباسية الميداني في سوريا يقدّم خدماته لنحو 1500 نازح لبناني يوميًا
|
|
|