


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 3-11-2020
Date: 11-11-2020
Date: 18-1-2021
|
An abundant number, sometimes also called an excessive number, is a positive integer 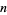 for which
for which
 |
(1) |
where 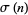 is the divisor function and
is the divisor function and 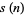 is the restricted divisor function. The quantity
is the restricted divisor function. The quantity  is sometimes called the abundance.
is sometimes called the abundance.
A number which is abundant but for which all its proper divisors are deficient is called a primitive abundant number (Guy 1994, p. 46).
The first few abundant numbers are 12, 18, 20, 24, 30, 36, ... (OEIS A005101).
Every positive integer 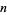 with
with 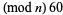 is abundant. Any multiple of a perfect number or an abundant number is also abundant. Prime numbers are not abundant. Every number greater than 20161 can be expressed as a sum of two abundant numbers.
is abundant. Any multiple of a perfect number or an abundant number is also abundant. Prime numbers are not abundant. Every number greater than 20161 can be expressed as a sum of two abundant numbers.
There are only 21 abundant numbers less than 100, and they are all even. The first odd abundant number is
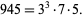 |
(2) |
That 945 is abundant can be seen by computing
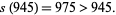 |
(3) |
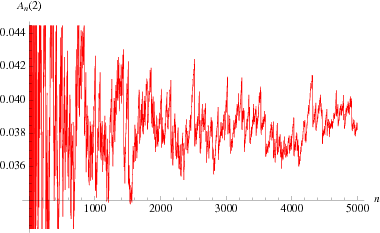
Define the density function
 |
(4) |
(correcting the expression in Finch 2003, p. 126) for a positive real number  where
where  gives the cardinal number of the set
gives the cardinal number of the set  , then Davenport (1933) proved that
, then Davenport (1933) proved that 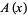 exists and is continuous for all
exists and is continuous for all  , and Erdős (1934) gave a simplified proof (Finch 2003). The special case
, and Erdős (1934) gave a simplified proof (Finch 2003). The special case 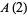 then gives the asymptotic density of abundant numbers,
then gives the asymptotic density of abundant numbers,
 |
(5) |
The following table summarizes improvements in bounds on the constant over time.
| value | reference |
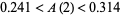 |
Behrend (1933) |
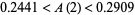 |
Wall (1971) and Wall et al. (1977) |
 |
Deléglise (1998) |
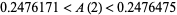 |
Kobayashi (2010, p. 12) |
REFERENCES:
Behrend, F. "Über numeri abundantes." Sitzungsber. Preuss. Akad. Wiss., Phys.-Math. Kl., No. 21/23, 322-328, 1932.
Behrend, F. "Über numeri abundantes. II." Sitzungsber. Preuss. Akad. Wiss., Phys.-Math. Kl., No. 6, 280-293, 1933.
Davenport, H. "Über numeri abundantes." Sitzungsber. Preuss. Akad. Wiss., Phys.-Math. Kl., No. 6, 830-837, 1933.
Deléglise, M. "Bounds for the Density of Abundant Integers." Exp. Math. 7, 137-143, 1998.
Dickson, L. E. History of the Theory of Numbers, Vol. 1: Divisibility and Primality. New York: Dover, pp. 3-33, 2005.
Erdős, P. "On the Density of the Abundant Numbers." J. London Math. Soc. 9, 278-282, 1934.
Finch, S. R. "Abundant Numbers Density Constant." §2.11 in Mathematical Constants. Cambridge, England: Cambridge University Press, pp. 126-127, 2003.
Guy, R. K. Unsolved Problems in Number Theory, 2nd ed. New York: Springer-Verlag, pp. 45-46, 1994.
Kobayashi, M. "On the Density of Abundant Numbers." Ph.D. thesis. Hanover, NH: Dartmouth College, 2010.
Singh, S. Fermat's Enigma: The Epic Quest to Solve the World's Greatest Mathematical Problem. New York: Walker, pp. 11 and 13, 1997.
Sloane, N. J. A. Sequence A005101/M4825 in "The On-Line Encyclopedia of Integer Sequences."
Souissi, M. Un Texte Manuscrit d'Ibn Al-Bannā' Al-Marrakusi sur les Nombres Parfaits, Abondants, Deficients, et Amiables. Karachi, Pakistan: Hamdard Nat. Found., 1975.
Wall, C. R. "Density Bounds for the Sum of Divisors Function." In The Theory of Arithmetic Functions: Proceedings of the Conference at Western Michigan University, April 29-May 1, 1971. (Ed. A. A. Gioia and D. L. Goldsmith). New York: Springer-Verlag, pp. 283-287, 1971.
Wall, C. R.; Crews, P. L.; and Johnson, D. B. "Density Bounds for the Sum of Divisors Function." Math. Comput. 26, 773-777, 1972.
Wall, C. R.; Crews, P. L.; and Johnson, D. B. "Density Bounds for the Sum of Divisors Function." Math. Comput. 31, 616, 1977.



|
|
|
|
4 أسباب تجعلك تضيف الزنجبيل إلى طعامك.. تعرف عليها
|
|
|
|
|
|
|
أكبر محطة للطاقة الكهرومائية في بريطانيا تستعد للانطلاق
|
|
|
|
|
|
|
أصواتٌ قرآنية واعدة .. أكثر من 80 برعماً يشارك في المحفل القرآني الرمضاني بالصحن الحيدري الشريف
|
|
|