


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 11-1-2021
Date: 2-9-2020
Date: 25-12-2019
|
Call a number of the form 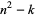 a "near-square number."
a "near-square number."
Numbers of the form  for
for 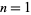 , 2, ... are
, 2, ... are 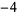 ,
, 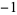 , 4, 11, 20, 31, 44, 59, 76, 95, ... (OEIS A028875). These are prime for indices
, 4, 11, 20, 31, 44, 59, 76, 95, ... (OEIS A028875). These are prime for indices 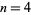 , 6, 8, 12, 14, 16, ... (OEIS A028876), corresponding to the primes 11, 31, 59, 139, 191, 251, 479, ... (OEIS A028877).
, 6, 8, 12, 14, 16, ... (OEIS A028876), corresponding to the primes 11, 31, 59, 139, 191, 251, 479, ... (OEIS A028877).
Numbers of the form 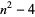 for
for 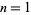 , 2, ... are
, 2, ... are 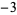 , 0, 5, 12, 21, 32, 45, 60, ... (OEIS A028347). Since they can always be factored as
, 0, 5, 12, 21, 32, 45, 60, ... (OEIS A028347). Since they can always be factored as 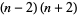 for
for 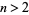 , the only (positive) prime of this form is 5.
, the only (positive) prime of this form is 5.
Numbers of the form 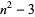 for
for 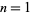 , 2, ... are
, 2, ... are 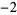 , 1, 6, 13, 22, 33, 46, 61, ... (OEIS A028872). These are prime for indices
, 1, 6, 13, 22, 33, 46, 61, ... (OEIS A028872). These are prime for indices 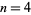 , 8, 10, 14, 20, 26, 32, ... (OEIS A028873), corresponding to the primes 13, 61, 97, 193, 397, 673, ... (OEIS A028874).
, 8, 10, 14, 20, 26, 32, ... (OEIS A028873), corresponding to the primes 13, 61, 97, 193, 397, 673, ... (OEIS A028874).
Numbers of the form 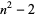 for
for 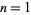 , 2, ... are
, 2, ... are 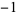 , 2, 7, 14, 23, 34, 47, 62, ... (OEIS A008865). These are prime for indices
, 2, 7, 14, 23, 34, 47, 62, ... (OEIS A008865). These are prime for indices 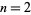 , 3, 5, 7, 9, 13, 15, 19, 21, 27, ... (OEIS A028870), corresponding to the primes 2, 7, 23, 47, 79, 167, 223, 359, 439, ... (OEIS A028871). The "Kynea primes" (see below) are special cases of this form.
, 3, 5, 7, 9, 13, 15, 19, 21, 27, ... (OEIS A028870), corresponding to the primes 2, 7, 23, 47, 79, 167, 223, 359, 439, ... (OEIS A028871). The "Kynea primes" (see below) are special cases of this form.
Numbers of the form 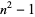 for
for  , 2, ... are 0, 3, 8, 15, 24, 35, 48, 63, ... (OEIS A005563). Since they can always be factored as
, 2, ... are 0, 3, 8, 15, 24, 35, 48, 63, ... (OEIS A005563). Since they can always be factored as 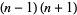 for
for 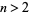 , the only prime of this form is 3.
, the only prime of this form is 3.
Numbers of the form 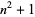 for
for 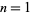 , 2, ... are 2, 5, 10, 17, 26, 37, 50, ... (OEIS A002522). These are prime for indices
, 2, ... are 2, 5, 10, 17, 26, 37, 50, ... (OEIS A002522). These are prime for indices 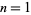 , 2, 4, 6, 10, 14, 16, 20, 24, 26, 36, ... (OEIS A005574), corresponding to the primes 2, 5, 17, 37, 101, 197, 257, 401, 577, 677, ... (OEIS A002496). Fermat primes and generalized Fermat primes (with
, 2, 4, 6, 10, 14, 16, 20, 24, 26, 36, ... (OEIS A005574), corresponding to the primes 2, 5, 17, 37, 101, 197, 257, 401, 577, 677, ... (OEIS A002496). Fermat primes and generalized Fermat primes (with 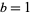 ) are of this form.
) are of this form.
Numbers of the form 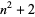 for
for 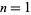 , 2, ... are 3, 6, 11, 18, 27, 38, 51, 66, 83, 102, ... (OEIS A059100). These are prime for indices
, 2, ... are 3, 6, 11, 18, 27, 38, 51, 66, 83, 102, ... (OEIS A059100). These are prime for indices 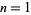 , 3, 9, 15, 21, 33, 39, 45, 57, 81, 99, ... (OEIS A067201), corresponding to the primes 3, 11, 83, 227, 443, 1091, 1523, 2027, ... (OEIS A056899).
, 3, 9, 15, 21, 33, 39, 45, 57, 81, 99, ... (OEIS A067201), corresponding to the primes 3, 11, 83, 227, 443, 1091, 1523, 2027, ... (OEIS A056899).
Numbers of the form 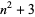 for
for 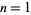 , 2, ... are 4, 7, 12, 19, 28, 39, 52, 67, ... (OEIS A117950). These are prime for indices
, 2, ... are 4, 7, 12, 19, 28, 39, 52, 67, ... (OEIS A117950). These are prime for indices 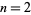 , 4, 8, 10, 14, 22, 28, 38, 50, 52, 62, ... (OEIS A049422), corresponding to the primes 7, 19, 67, 103, 199, 487, 787, 1447, ... (OEIS A049423).
, 4, 8, 10, 14, 22, 28, 38, 50, 52, 62, ... (OEIS A049422), corresponding to the primes 7, 19, 67, 103, 199, 487, 787, 1447, ... (OEIS A049423).
Numbers of the form 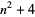 for
for  , 2, ... are 5, 8, 13, 20, 29, 40, 53, 68, 85, ... (OEIS A087475). These are prime for indices
, 2, ... are 5, 8, 13, 20, 29, 40, 53, 68, 85, ... (OEIS A087475). These are prime for indices 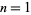 , 3, 5, 7, 13, 15, 17, 27, ... (OEIS A007591), corresponding to the primes 5, 13, 29, 53, 173, 229, 293, 733, ... (OEIS A005473).
, 3, 5, 7, 13, 15, 17, 27, ... (OEIS A007591), corresponding to the primes 5, 13, 29, 53, 173, 229, 293, 733, ... (OEIS A005473).
Numbers of the form 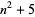 for
for 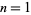 , 2, ... are 6, 9, 14, 21, 30, 41, 54, 69, ... (OEIS A117951). These are prime for indices
, 2, ... are 6, 9, 14, 21, 30, 41, 54, 69, ... (OEIS A117951). These are prime for indices 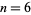 , 12, 36, 48, 72, 78, 96, ... (OEIS A078402), corresponding to the primes 41, 149, 1301, 2309, 5189, ... (OEIS A056905).
, 12, 36, 48, 72, 78, 96, ... (OEIS A078402), corresponding to the primes 41, 149, 1301, 2309, 5189, ... (OEIS A056905).
For 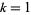 , 2, ..., the first few near-square numbers of the form
, 2, ..., the first few near-square numbers of the form 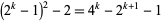 are
are  , 7, 47, 223, 959, 3967, ... (OEIS A093112). As of Jun. 2016, a total of 42 primes of this form (arbitrarily dubbed Carol primes by their original investigator in reference to a personal acquaintance) are known. The first few have indices 2, 3, 4, 6, 7, 10, 12, 15, 18, 19, 21, 25, 27, 55, 129, ... (OEIS A091515), corresponding to the primes 7, 47, 223, 3967, 16127, 1046527, ... (OEIS A091516). Some large primes of this form are summarized in the following table.
, 7, 47, 223, 959, 3967, ... (OEIS A093112). As of Jun. 2016, a total of 42 primes of this form (arbitrarily dubbed Carol primes by their original investigator in reference to a personal acquaintance) are known. The first few have indices 2, 3, 4, 6, 7, 10, 12, 15, 18, 19, 21, 25, 27, 55, 129, ... (OEIS A091515), corresponding to the primes 7, 47, 223, 3967, 16127, 1046527, ... (OEIS A091516). Some large primes of this form are summarized in the following table.
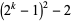 |
decimal digits | discoverer |
 |
152916 | C. Emmanuel (May 7, 2007; Harvey; https://primes.utm.edu/primes/page.php?id=80384) |
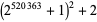 |
313290 | M. Rodenkirch (Apr. 14, 2016) |
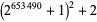 |
393441 | M. Rodenkirch (Jun. 15, 2016) |
For 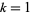 , 2, ..., the first few near-square numbers of the form
, 2, ..., the first few near-square numbers of the form 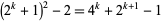 are 2, 7, 23, 79, 287, 1087, 4223, 16639, ... (OEIS A093069). As of Jun. 2016, a total of 50 primes of this form (arbitrarily dubbed Kynea primes by their original investigator in reference to a personal acquaintance) are known. The first few have indices 0, 1, 2, 3, 5, 8, 9, 12, 15, 17, 18, 21, ... (OEIS A091513) and are given by 2, 7, 23, 79, 1087, 66047, 263167, 16785407, ... (OEIS A091514). Some large primes of this form are summarized in the following table.
are 2, 7, 23, 79, 287, 1087, 4223, 16639, ... (OEIS A093069). As of Jun. 2016, a total of 50 primes of this form (arbitrarily dubbed Kynea primes by their original investigator in reference to a personal acquaintance) are known. The first few have indices 0, 1, 2, 3, 5, 8, 9, 12, 15, 17, 18, 21, ... (OEIS A091513) and are given by 2, 7, 23, 79, 1087, 66047, 263167, 16785407, ... (OEIS A091514). Some large primes of this form are summarized in the following table.
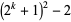 |
decimal digits | discoverer |
 |
169553 | C. Emmanuel (October 2005); https://primes.utm.edu/primes/page.php?id=75878 |
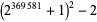 |
222510 | M. Rodenkirch (Feb. 2016) |
 |
226405 | M. Rodenkirch (Feb. 7, 2016) |
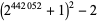 |
266142 | M. Rodenkirch (Feb. 28, 2016) |
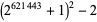 |
374146 | M. Rodenkirch (May 30, 2016) |
 |
398250 | M. Rodenkirch (Jun. 19, 2016) |
REFERENCES:
Harvey, S. https://harvey563.tripod.com/Carol_Kynea.txt.
Minovic, P. "Carol/Kynea New Records." primenumbers discussion group. Feb. 20, 2004a. https://groups.yahoo.com/group/primenumbers/message/14574.
Minovic, P. "Re: Carol/Kynea New Records." primenumbers discussion group. Feb. 24, 2004b. https://groups.yahoo.com/group/primenumbers/message/14586.
Rodenkirch, M. "Carol and Kynea Prime Search." https://www.mersenneforum.org/rogue/ckps.html.
Sloane, N. J. A. Sequences A002496/M1506, A002522, A005563/M2720, A005574/M1010, A005473/M005473, A007591/M2416, A008865, A028347, A028870, A028871, A028872, A028873, A028874, A028875, A028876, A028877, A049422, A049423, A056899, A056905, A059100, A060867, A067201, A078402, A087475, A091513, A091514, A091515, A091516, A093069, A093112, A117950, and A117951 in "The On-Line Encyclopedia of Integer Sequences."



|
|
|
|
علامات بسيطة في جسدك قد تنذر بمرض "قاتل"
|
|
|
|
|
|
|
أول صور ثلاثية الأبعاد للغدة الزعترية البشرية
|
|
|
|
|
|
|
مدرسة دار العلم.. صرح علميّ متميز في كربلاء لنشر علوم أهل البيت (عليهم السلام)
|
|
|