


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 12-10-2018
Date: 23-4-2019
Date: 8-8-2019
|
Find the shape of the curve down which a bead sliding from rest and accelerated by gravity will slip (without friction) from one point to another in the least time. The term derives from the Greek 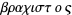 (brachistos) "the shortest" and
(brachistos) "the shortest" and  (chronos) "time, delay."
(chronos) "time, delay."
The brachistochrone problem was one of the earliest problems posed in the calculus of variations. Newton was challenged to solve the problem in 1696, and did so the very next day (Boyer and Merzbach 1991, p. 405). In fact, the solution, which is a segment of a cycloid, was found by Leibniz, L'Hospital, Newton, and the two Bernoullis. Johann Bernoulli solved the problem using the analogous one of considering the path of light refracted by transparent layers of varying density (Mach 1893, Gardner 1984, Courant and Robbins 1996). Actually, Johann Bernoulli had originally found an incorrect proof that the curve is a cycloid, and challenged his brother Jakob to find the required curve. When Jakob correctly did so, Johann tried to substitute the proof for his own (Boyer and Merzbach 1991, p. 417).
In the solution, the bead may actually travel uphill along the cycloid for a distance, but the path is nonetheless faster than a straight line (or any other line).
The time to travel from a point 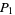 to another point
to another point 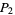 is given by the integral
is given by the integral
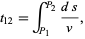 |
(1) |
where 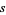 is the arc length and
is the arc length and 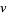 is the speed. The speed at any point is given by a simple application of conservation of energy equating kinetic energy to gravitational potential energy,
is the speed. The speed at any point is given by a simple application of conservation of energy equating kinetic energy to gravitational potential energy,
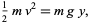 |
(2) |
giving
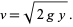 |
(3) |
Plugging this into (◇) together with the identity
 |
(4) |
then gives
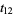 |
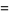 |
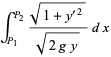 |
(5) |
 |
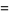 |
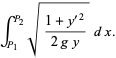 |
(6) |
The function to be varied is thus
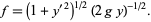 |
(7) |
To proceed, one would normally have to apply the full-blown Euler-Lagrange differential equation
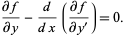 |
(8) |
However, the function 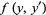 is particularly nice since
is particularly nice since  does not appear explicitly. Therefore,
does not appear explicitly. Therefore, 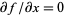 , and we can immediately use the Beltrami identity
, and we can immediately use the Beltrami identity
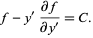 |
(9) |
Computing
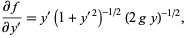 |
(10) |
subtracting 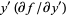 from
from 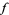 , and simplifying then gives
, and simplifying then gives
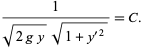 |
(11) |
Squaring both sides and rearranging slightly results in
![[1+((dy)/(dx))^2]y](http://mathworld.wolfram.com/images/equations/BrachistochroneProblem/Inline18.gif) |
 |
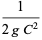 |
(12) |
 |
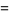 |
 |
(13) |
where the square of the old constant  has been expressed in terms of a new (positive) constant
has been expressed in terms of a new (positive) constant  . This equation is solved by the parametric equations
. This equation is solved by the parametric equations
 |
 |
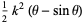 |
(14) |
 |
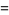 |
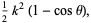 |
(15) |
which are--lo and behold--the equations of a cycloid.
If kinetic friction is included, the problem can also be solved analytically, although the solution is significantly messier. In that case, terms corresponding to the normal component of weight and the normal component of the acceleration(present because of path curvature) must be included. Including both terms requires a constrained variational technique (Ashby et al. 1975), but including the normal component of weight only gives an approximate solution. The tangent and normal vectors are
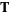 |
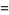 |
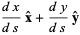 |
(16) |
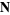 |
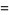 |
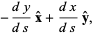 |
(17) |
gravity and friction are then
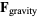 |
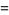 |
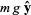 |
(18) |
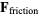 |
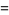 |
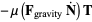 |
(19) |
 |
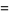 |
 |
(20) |
and the components along the curve are
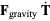 |
 |
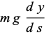 |
(21) |
 |
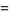 |
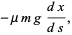 |
(22) |
so Newton's Second Law gives
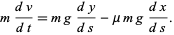 |
(23) |
But
 |
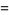 |
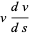 |
(24) |
 |
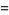 |
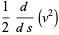 |
(25) |
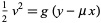 |
(26) |
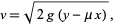 |
(27) |
so
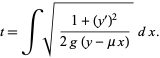 |
(28) |
Using the Euler-Lagrange differential equation gives
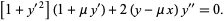 |
(29) |
This can be reduced to
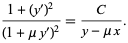 |
(30) |
Now letting
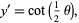 |
(31) |
the solution is
 |
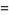 |
![1/2k^2[(theta-sintheta)+mu(1-costheta)]](http://mathworld.wolfram.com/images/equations/BrachistochroneProblem/Inline61.gif) |
(32) |
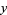 |
 |
![1/2k^2[(1-costheta)+mu(theta+sintheta)].](http://mathworld.wolfram.com/images/equations/BrachistochroneProblem/Inline64.gif) |
(33) |
REFERENCES:
Ashby, N.; Brittin, W. E.; Love, W. F.; and Wyss, W. "Brachistochrone with Coulomb Friction." Amer. J. Phys. 43, 902-905, 1975.
Boyer, C. B. and Merzbach, U. C. A History of Mathematics, 2nd ed. New York: Wiley, 1991.
Courant, R. and Robbins, H. What Is Mathematics?: An Elementary Approach to Ideas and Methods, 2nd ed. Oxford, England: Oxford University Press, 1996.
Gardner, M. The Sixth Book of Mathematical Games from Scientific American. Chicago, IL: University of Chicago Press, pp. 130-131, 1984.
Haws, L. and Kiser, T. "Exploring the Brachistochrone Problem." Amer. Math. Monthly 102, 328-336, 1995.
Hayen, J. C. "Brachistochrone with Coulomb Friction." Int. J. Non-Linear Mech. 40, 1057-1075, 2005.
Lipp, S. C. "Brachistochrone with Coulomb Friction." SIAM J. Control Optim. 35, 562-584, 1997.
Mach, E. The Science of Mechanics. Chicago, IL: Open Court, 1893.
Phillips, J. P. "Brachistochrone, Tautochrone, Cycloid--Apple of Discord." Math. Teacher 60, 506-508, 1967.
Smith, D. E. History of Mathematics, Vol. 2: Special Topics of Elementary Mathematics. New York: Dover, p. 326, 1958.
Steinhaus, H. Mathematical Snapshots, 3rd ed. New York: Dover, pp. 148-149, 1999.
Wagon, S. Mathematica in Action. New York: W. H. Freeman, pp. 60-66 and 385-389, 1991.
Wells, D. The Penguin Dictionary of Curious and Interesting Geometry. London: Penguin, p. 46, 1991.



|
|
|
|
دخلت غرفة فنسيت ماذا تريد من داخلها.. خبير يفسر الحالة
|
|
|
|
|
|
|
ثورة طبية.. ابتكار أصغر جهاز لتنظيم ضربات القلب في العالم
|
|
|
|
|
|
|
العتبة العباسية المقدسة تقدم دعوة إلى كلية مزايا الجامعة للمشاركة في حفل التخرج المركزي الخامس
|
|
|