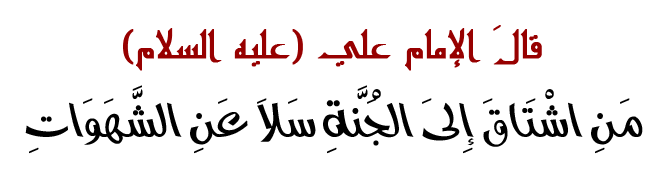
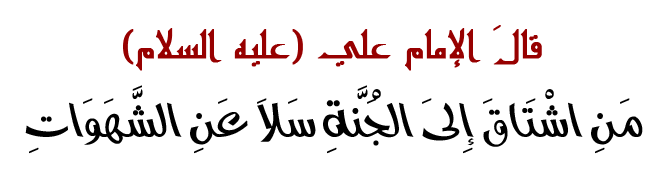

 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 13-11-2016
Date: 13-11-2016
Date: 13-11-2016
|
Died: 27 March 1888 in Turin, Italy

Francesco Faà di Bruno's parents were Carolina Sappa and Luigi Faà di Bruno. Francesco was the youngest of his parents' twelve children and he had seven sisters and four brothers. His father was a wealthy landowner with several titles such as Marquis of Bruno, Count of Carentino, Lord of Fontanile, and Patrizio of Alessandria. The village of Bruno is about 18 km south west of Alessandria, a major city roughly equidistant from Turin, Milan and Genoa. Francesco's mother, Carolina, was from a noble family from Milan. Patrizia Solari writes [11] that Luigi and Carolina had:-
... a happy marriage, one of the most wealthy of the Piedmontese nobility and most generous to the needy people.
Although he is always known as Francesco, the full name of the subject of this biography was Francesco da Paola Virgilio Secondo Maria Faà di Bruno. One of Francesco's brothers, Giuseppe Maria Faà di Bruno, built the church of St Peter in Hatton Garden, London, the most important church for Italian immigrants. He was a leader of the Pious Society of Missions and wrote "Catholic Belief", an exposition of Catholic doctrine aimed at non-Catholics. Over one million copies of this book were sold. Another of Francesco's brothers, Emilio, served in the army and was killed in the Battle of Lissa in 1866. Two of Francesco's sisters became nuns.
When Francesco was nine years old, his mother died. His early education had been at home and he began his school career in 1836 when he entered the college of the Novi Ligure dei Padri Somaschi. After studying for four years at the Novi Ligure he entered the Royal Military Academy of Turin in 1840 with the aim of making a career in the army. It was a six-year course and after graduating in 1846 he was commissioned as a lieutenant in the army. The advanced courses he took included topography and foreign languages. The revolutions which broke out across Europe in 1848 led to the First Italian War of Independence in which Faà di Bruno participated in the Piedmontese Brigata Guardie commanded by Vittorio Emanuele. For six weeks the Brigata Guardie attacked the Austrians at Peschiera del Garda and were victorious on 30 May 1848. After this military campaign there was an uneasy truce was lasted around seven months. Faà di Bruno's main task during these months was to draw up maps and he produced his major map of the Mincio area. He undertook his duties with great enthusiasm, realising that the generals commanding the Piedmontese armies did not have recent accurate maps of the Lombardo-Veneto region. In 1849 he was promoted to captain and shortly after this, in March 1849, the Austrians broke the true and the resulting battle between the Austrian and Piedmontese armies at Novara on 22 March was a humiliating defeat for the Piedmontese. Faà di Bruno's horse was killed by Austrian rifle fire, so he mounted another horse only to have that killed too. He was wounded in the leg and seeing many of his comrades killed, he realised that an army career was not for him.
Charles Albert, the King of Sardinia, resigned following the defeat at Novara and his son Vittorio Emanuele became King of Sardinia. He had two young sons and, realising Faà di Bruno was both an extraordinary person and an exceptional scholar, he asked him to tutor his two young sons. However, Faà di Bruno was known as a devout Catholic and the King immediately came under pressure to appoint a secular tutor for his sons. Vittorio Emanuele decided that, for political reasons, he had to withdraw his offer to Faà di Bruno which he did. Then Faà di Bruno asked permission to leave the army and take up the study of mathematics. He travelled to Paris in 1850 where he studied at the Sorbonne under Augustin-Louis Cauchy who [2]:-
... he admired, not only for his genius, but also for his religious fervour and his philanthropy.
At the Sorbonne Faà di Bruno was in the same classes as Charles Hermite and the two became close friends. However, his time in Paris was not all spent on academic studies for he also assisted in the parish of Saint Sulpice and visited the homes of the poor. He greatly enjoyed visiting the bookshops of Paris and shops where scientific instruments were sold. While in Paris he began publishing mathematical papers: Note sur un nouveau procédé pour reconnaître immédiatment, dans certains cas, l'existence de racines imaginaires dans une équation numérique (1850); Démonstration d'un théorème de M Sylvester, relatif à la décomposition d'un produit de deux déterminants (1851); and Démonstration d'un théorème relatif à la réduction des fonctions homogènes à deux lettres à leur forme canonique (1852). After graduating from Paris with his Licence in Science in 1851, he returned to Turin but this was a difficult time for anyone who was a devout Catholic [3]:-
Among the greatest tragedies of his life, there will always be the contrast between his aspirations, as a sincere patriot desiring Italian unity, but rejection, as a Catholic loyal to the pope, of the methods and unacceptable manner in which that unity was pursued with persecution and oppression of the Church.
The Church of San Massimo, in Borgo Nuovo, was completed in June 1853 and, in the following year, Faà di Bruno set up a choral school for women in the church where they were trained every Sunday by Faà di Bruno who played the organ. He now published papers in Italian such as Sullo stabilimento di un osservatorio magnetico e meteorologico in Torino (1853), and Theorema di geometria (1853), and also continued to publish in French, for example Note sur un théorème de M Brioschi (1854). In May 1855 Faà di Bruno returned to Paris to undergo further training at the astronomical observatory at Brera. The authorities in Turin promised him that, after he returned from Paris, he would be employed at the Observatory in Turin - a promise they did not keep. However, in Paris Faà di Bruno studied astronomy under Urbain Le Verrier and also undertook research with Cauchy on mathematics. He graduated in 1856 having presented two theses, one in mathematics Théorie générale de l'élimination on elimination theory, and the other in astronomy on celestial mechanics. While in Paris he had also invented a mechanical device to allow blind people to write. He had personal reasons to invent such an apparatus, for his sister Maria Luigia was becoming blind.
He continued to publish papers in both French and in Italian: Sullo sviluppo delle Funzioni (1855), Sulle Funzioni Isobariche (1856) and Note sur une nouvelle formule de calcul différentiel (1857) were all written while he was undertaking research in Paris. Both the first and the third of these papers contain the result for which he is best known, namely Faà di Bruno's formula. This formula gives the nth derivative of the composition of two functions f (g(x)). Warren Johnson writes [7]:-
Once Faà di Bruno's formula was considered a real analysis result: it is in the 'Cours d'Analyse' of Goursat and of de la Vallée Poussin. Riordan and Comtet saw it as part of combinatorial analysis, a term that seems to be going out of fashion; the subject subsumed in algebraic combinatorics, the books of Riordan and Comtet largely superseded by Stanley's monumental 'Enumerative combinatorics' (1997, 1999) where Faà di Bruno's formula is mentioned, but not stated. It can also be found in books on partitions [George E Andrews, 'The theory of partitions' (1976)], mathematical statistics, matrix theory, calculus of finite differences, computer science [Donald E Knuth, 'The Art of Computer Programming' (1968)], symmetric functions, and miscellaneous mathematical techniques.
Several authors have pointed out that Faà di Bruno was not the first to either state or prove this result. The papers [4] and [7] give fascinating accounts of earlier work. However, Faà di Bruno did give a form of the formula using determinants which nobody had found earlier. After his time in Paris, he was appointed as a lecturer in higher analysis at the University of Turin, and he also gave popular astronomy courses. In 1859 he published an important book in French, Théorie générale de l'élimination. By this stage in his career he had 20 works published. From 1859 Angelo Genocchi held the Chair of Algebra and Complementary Geometry at Turin, then in the following year he moved to the Chair of Higher Analysis and Faà di Bruno was appointed as his deputy. He obtained his doctorate, essentially a D.Sc., in 1861.
After his time studying in the Sorbonne, Faà di Bruno did much charity work on his return to Turin. He had seen food being prepared and distributed to the poor while in Paris and, back in Turin, he began to organise a similar scheme during the winter months. At this time Faà di Bruno came in contact with Giovanni Bosco. Bosco had been ordained a Roman Catholic priest in 1841 in Turin and began to work there to help boys who came to look for work in the city. Bosco provided boys with education, religious instruction, and recreation. Eventually he headed a large establishment containing a grammar school, a technical school, and a church, all built through his efforts. In Turin Bosco and others founded the Society of St Francis de Sales in 1859. Faà di Bruno, following Bosco's example, founded the Pia opera di Santa Zita in the San Donato area of Turin on 2 February 1859. He used his own money together with funds he had collected standing at the doors of churches. He chose the site because this was an area of Turin which was inhabited by the poorest people. Also in 1859 Faà di Bruno founded the Opera per la santificazione delle feste, a Society to promote Sunday observance and to protect workers who were being forced to work on Sundays. He was president of the Society and Bosco accepted the role of vice-president.
Faà di Bruno was incredibly energetic in his work with the poor. In 1860 he founded the Infermeria di San Giuseppe, an infirmary for poor women and the sick where people had an opportunity to convalesce after an illness. In 1862 he founded a boarding-house for the elderly and disabled women. In fact one has to realise that there was much illness in Turin at this time. Frequent epidemics, especially of typhus and cholera, were the result of poor hygiene and Faà di Bruno was able to set up wash rooms in the San Donato area. But his charity work was not restricted to caring for the sick, for he was also passionate about providing educational opportunities for the young people from poor families. He organised a mobile library in 1863 which provided books on many topics, particularly religion and science. In 1864 he set up classes providing training in home economics and, in 1866, he organised courses to train people to become elementary school teachers.
We mentioned above that Emilio Faà di Bruno, one of Francesco's brothers, served in the army and was killed in the Battle of Lissa in 1866. Francesco began the building of the church of Nostra Signora del Suffragio in 1866, following the death of his brother. Building the church took around three years and much of the architectural design was due to Faà di Bruno himself. His experience as a soldier, and his brother's death, had greatly affected him and he held daily prayers in the church of Nostra Signora del Suffragio for the souls of all soldiers killed in wars. Despite the many long hours he spent undertaking charity work, Faà di Bruno did not ignore his mathematical research. He continued to publish articles and gained international fame as a mathematician. This fame, however, did not lead to rapid promotion within the University of Turin. The reason for this was the secular nature of the Italian Independence movement with discrimination against those active in the Church. In 1871 he was put in charge of teaching calculus and analytic geometry and he was appointed as an extraordinary professor of higher analysis in 1876. His colleagues thought very highly of him and seven times they put him forward for a chair. Although he is usually remembered today because of "Faà di Bruno's formula", his most influential mathematical work was his book Théorie des formes binaires on binary forms which he published in 1876. The book was based on lectures that Faà di Bruno had given at the University of Turin. The book became better known in 1881 when Max Noether published a German edition. Paul Gordan wrote to Faà di Bruno from Erlangen on 29 September 1875 and his letter is reproduced, both in the original German and in a French translation, in the Preface to Théorie des formes binaires:-
I have had the opportunity to read your book on binary forms, and I was happy because I found it well adapted to introduce the reader to the theory of invariants. The subject is thoroughly and brilliantly set out, the exposition is simple, clear and, in several places, elegant. ... You have with this work delivered a service to science for which it will be grateful, since you have filled an important gap.
Not only did Faà di Bruno have difficulties in obtaining promotion within the university, but he also had difficulty in becoming ordained. Although he had undertaken the necessary training, his archbishop was opposed to ordaining men later in their lives. Faà di Bruno had to make a special plea to pope Pius IX to overrule the archbishop before he was ordained a Roman Catholic priest in Rome on 22 October 1876. The religious order he had founded, the Suore Minime di Nostra Signora del Suffragio, supported girls in a house called the Conservatorio del Suffragio. In order to provide work for the girls, Faà di Bruno had the idea that they could train as typesetters. He purchased a printing press and set up the Tipographia Suffragio. There a number of mathematics books were published including one by Faà di Bruno himself on elliptic functions. In 1898, ten years after Faà di Bruno's death, the printing press was purchased by Giuseppe Peano for 407 lire and he printed the Rivista di Matematica on it for several years.
In [2] Faà di Bruno is described as follows:-
Faà di Bruno was tall and not always well dressed, but he was simple and good natured. He was of a solitary disposition and spoke seldom (and not always successfully in the classroom). He cultivated music and was said to be a good pianist.
In fact, to give a little more information on his musical talents, he composed scared melodies which were highly thought of by Franz Liszt. We have mentioned above his interest in scientific instruments, but let us add that he invented, among other things, a differential barometer, described in a publication of 1870, and an electric alarm clock. He set up a Foucault pendulum in his church, the Chiesa del Suffragio, to demonstrate the rotation of the earth.
He died suddenly, two days short of his 63rd birthday, from an intestinal infection. Bosco, who had been an inspiration to Faà di Bruno and had died less than two months before him, was made a Saint on 1 April 1934. Already by this time there was a movement to canonise Faà di Bruno and in 1955 the Sacred Congregation of Rites officially accepted the claim for Faà di Bruno to be canonised. Faà di Bruno was declared a Saint by pope John Paul II in St Peter's Square in Rome on 25 September 1988.
Books:
Articles:



|
|
|
|
لخفض ضغط الدم.. دراسة تحدد "تمارين مهمة"
|
|
|
|
|
|
|
طال انتظارها.. ميزة جديدة من "واتساب" تعزز الخصوصية
|
|
|
|
|
|
|
مشاتل الكفيل تزيّن مجمّع أبي الفضل العبّاس (عليه السلام) بالورد استعدادًا لحفل التخرج المركزي
|
|
|