


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 18-8-2020
Date: 6-9-2020
Date: 9-8-2020
|
Let  be a positive nonsquare integer. Then Artin conjectured that the set
be a positive nonsquare integer. Then Artin conjectured that the set  of all primes for which
of all primes for which  is a primitive root is infinite. Under the assumption of the generalized Riemann hypothesis, Artin's conjecture was solved by Hooley (1967; Finch 2003, p. 105).
is a primitive root is infinite. Under the assumption of the generalized Riemann hypothesis, Artin's conjecture was solved by Hooley (1967; Finch 2003, p. 105).
Let 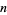 be not an
be not an  th power for any
th power for any 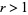 such the squarefree part
such the squarefree part 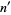 of
of 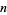 satisfies
satisfies 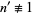 (mod 4). Let
(mod 4). Let 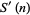 be the set of all primes for which such an
be the set of all primes for which such an 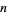 is a primitive root. Then Artin also conjectured that the density of
is a primitive root. Then Artin also conjectured that the density of 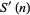 relative to the primes is given independently of the choice of
relative to the primes is given independently of the choice of 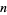 by
by 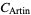 , where
, where
![C_(Artin)=product_(k=1)^infty[1-1/(p_k(p_k-1))]=0.3739558136...](https://mathworld.wolfram.com/images/equations/ArtinsConstant/NumberedEquation1.gif) |
(1) |
(OEIS A005596), and 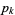 is the
is the 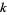 th prime.
th prime.
The significance of Artin's constant is more easily seen by describing it as the fraction of primes 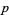 for which
for which 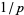 has a maximal period repeating decimal, i.e.,
has a maximal period repeating decimal, i.e., 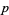 is a full reptend prime (Conway and Guy 1996) corresponding to a cyclic number.
is a full reptend prime (Conway and Guy 1996) corresponding to a cyclic number.
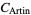 is connected with the prime zeta function
is connected with the prime zeta function 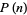 by
by
 |
(2) |
where 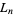 is a Lucas number (Ribenboim 1998, Gourdon and Sebah). Wrench (1961) gave 45 digits of
is a Lucas number (Ribenboim 1998, Gourdon and Sebah). Wrench (1961) gave 45 digits of 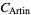 , and Gourdon and Sebah give 60.
, and Gourdon and Sebah give 60.
If  and
and  is still restricted not to be an
is still restricted not to be an 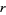 th power, then the density is not
th power, then the density is not 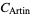 itself, but a rational multiple thereof. The explicit formula for computing the density in this case is conjectured to be
itself, but a rational multiple thereof. The explicit formula for computing the density in this case is conjectured to be
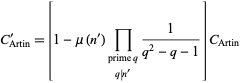 |
(3) |
(Matthews 1976, Finch 2003), where 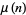 is the Möbius function. Special cases can be written down explicitly for
is the Möbius function. Special cases can be written down explicitly for  a prime,
a prime,
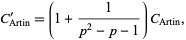 |
(4) |
or 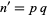 , where
, where 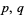 are both primes with
are both primes with 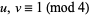 ,
,
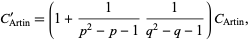 |
(5) |
If  is a perfect cube (which is not a perfect square), a perfect fifth power (which is not a perfect square or perfect cube), etc., other formulas apply (Hooley 1967, Western and Miller 1968).
is a perfect cube (which is not a perfect square), a perfect fifth power (which is not a perfect square or perfect cube), etc., other formulas apply (Hooley 1967, Western and Miller 1968).
REFERENCES:
Artin, E. Collected Papers (Ed. S. Lang and J. T. Tate). New York: Springer-Verlag, pp. viii-ix, 1965.
Conway, J. H. and Guy, R. K. The Book of Numbers. New York: Springer-Verlag, p. 169, 1996.
Finch, S. R. "Artin's Constant." §2.4 in Mathematical Constants. Cambridge, England: Cambridge University Press, pp. 104-110, 2003.
Gourdon, X. and Sebah, P. "Some Constants from Number Theory." https://numbers.computation.free.fr/Constants/Miscellaneous/constantsNumTheory.html.
Hooley, C. "On Artin's Conjecture." J. reine angew. Math. 225, 209-220, 1967.
Hooley, C. Applications of Sieve Methods to the Theory of Numbers. Cambridge, England: Cambridge University Press, 1976.
Ireland, K. and Rosen, M. A Classical Introduction to Modern Number Theory, 2nd ed. New York: Springer-Verlag, 1990.
Lehmer, D. H. and Lehmer, E. "Heuristics Anyone?" In Studies in Mathematical Analysis and Related Topics: Essays in Honor of George Pólya (Ed. G. Szegö, C. Loewner, S. Bergman, M. M. Schiffer, J. Neyman, D. Gilbarg, and H. Solomon). Stanford, CA: Stanford University Press, pp. 202-210, 1962.
Lenstra, H. W. Jr. "On Artin's Conjecture and Euclid's Algorithm in Global Fields." Invent. Math. 42, 201-224, 1977.
Matthews, K. R. "A Generalization of Artin's Conjecture for Primitive Roots." Acta Arith. 29, 113-146, 1976.
Ram Murty, M. "Artin's Conjecture for Primitive Roots." Math. Intell. 10, 59-67, 1988.
Ribenboim, P. The New Book of Prime Number Records. New York: Springer-Verlag, 1996.
Shanks, D. Solved and Unsolved Problems in Number Theory, 4th ed. New York: Chelsea, pp. 80-83, 1993.
Sloane, N. J. A. Sequence A005596/M2608 in "The On-Line Encyclopedia of Integer Sequences."
Western, A. E. and Miller, J. C. P. Tables of Indices and Primitive Roots. Cambridge, England: Cambridge University Press, pp. xxxvii-xlii, 1968.
Wrench, J. W. "Evaluation of Artin's Constant and the Twin Prime Constant." Math. Comput. 15, 396-398, 1961.



|
|
|
|
دراسة يابانية لتقليل مخاطر أمراض المواليد منخفضي الوزن
|
|
|
|
|
|
|
اكتشاف أكبر مرجان في العالم قبالة سواحل جزر سليمان
|
|
|
|
|
|
|
المجمع العلمي ينظّم ندوة حوارية حول مفهوم العولمة الرقمية في بابل
|
|
|