


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 23-8-2019
Date: 17-9-2018
Date: 12-8-2018
|
A formal extension of the hypergeometric function to two variables, resulting in four kinds of functions (Appell 1925; Picard 1880ab, 1881; Goursat 1882; Whittaker and Watson 1990, Ex. 22, p. 300),
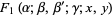 |
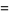 |
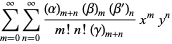 |
(1) |
 |
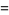 |
 |
(2) |
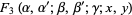 |
 |
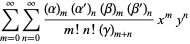 |
(3) |
 |
 |
 |
(4) |
These double series are absolutely convergent for
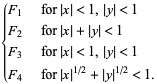 |
(5) |
Appell defined the functions in 1880 and they were subsequently studied by Picard in 1881. The functions 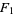 ,
, 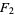 , and
, and 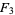 can be expressed in terms of double integrals as
can be expressed in terms of double integrals as
 |
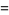 |
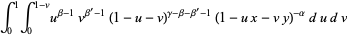 |
(6) |
 |
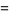 |
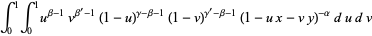 |
(7) |
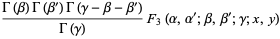 |
 |
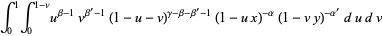 |
(8) |
(Bailey 1934, pp. 76-77). There appears to be no simple integral representation of this type for the function 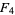 (Bailey 1934, p. 77).
(Bailey 1934, p. 77).
The function 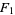 can also be expressed by the simple integral
can also be expressed by the simple integral
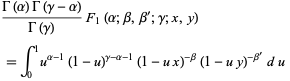 |
(9) |
(Bailey 1934, p. 77), for ![R[alpha]>0](http://mathworld.wolfram.com/images/equations/AppellHypergeometricFunction/Inline27.gif) and
and ![R[gamma-alpha]>0](http://mathworld.wolfram.com/images/equations/AppellHypergeometricFunction/Inline28.gif) .
.
The Appell functions are special cases of the Kampé de Fériet function, and are the first four in the set of Horn functions. The 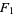 function is implemented in the Wolfram Language as AppellF1[a, b1, b2, c, x, y].
function is implemented in the Wolfram Language as AppellF1[a, b1, b2, c, x, y].
For general complex parameters, the 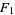 function can be written as the contour integral
function can be written as the contour integral
 |
(10) |
for 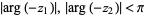 , where
, where 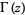 is a gamma function and
is a gamma function and 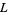 and
and 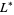 are complicated contours related to those used in the definition of theMeijer G-function. In fact, the four functions can also be expressed as double contour integrals taken along contours of the Barnes type (Bailey 1934).
are complicated contours related to those used in the definition of theMeijer G-function. In fact, the four functions can also be expressed as double contour integrals taken along contours of the Barnes type (Bailey 1934).
In particular, the general integral
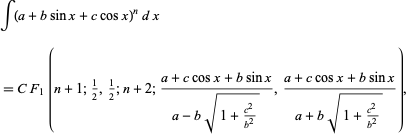 |
(11) |
where
 |
(12) |
has a closed form in terms of  .
.
Integrals that result in particularly nice closed forms involving the 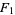 function include
function include
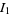 |
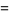 |
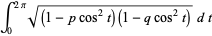 |
(13) |
 |
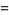 |
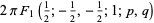 |
(14) |
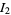 |
 |
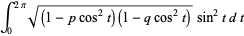 |
(15) |
 |
 |
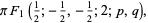 |
(16) |
which arise in computing area and geometric centroid of the interior of the cranioid curve.
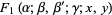 reduces to the hypergeometric function in the cases
reduces to the hypergeometric function in the cases
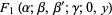 |
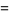 |
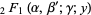 |
(17) |
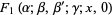 |
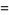 |
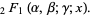 |
(18) |
In addition,
 |
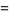 |
 |
(19) |
 |
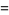 |
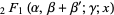 |
(20) |
 |
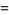 |
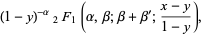 |
(21) |
where  is a hypergeometric function.
is a hypergeometric function.
REFERENCES:
Appell, P. "Sur les fonctions hypergéométriques de plusieurs variables." In Mémoir. Sci. Math. Paris: Gauthier-Villars, 1925.
Appell, P. and Kampé de Fériet, J. Fonctions hypergéométriques et hypersphériques: polynomes d'Hermite. Paris: Gauthier-Villars, 1926.
Bailey, W. N. "A Reducible Case of the Fourth Type of Appell's Hypergeometric Functions of Two Variables." Quart. J. Math. (Oxford) 4, 305-308, 1933.
Bailey, W. N. "On the Reducibility of Appell's Function  ." Quart. J. Math. (Oxford) 5, 291-292, 1934.
." Quart. J. Math. (Oxford) 5, 291-292, 1934.
Bailey, W. N. "Appell's Hypergeometric Functions of Two Variables." Ch. 9 in Generalised Hypergeometric Series. Cambridge, England: Cambridge University Press, pp. 73-83 and 99-101, 1935.
Erdélyi, A.; Magnus, W.; Oberhettinger, F.; and Tricomi, F. G. Higher Transcendental Functions, Vol. 1. New York: Krieger, pp. 222 and 224, 1981.
Exton, H. Handbook of Hypergeometric Integrals: Theory, Applications, Tables, Computer Programs. Chichester, England: Ellis Horwood, p. 27, 1978.
Goursat, E. "Extension du problème de Riemann à des fonctions hypergéométriques de deux variables." Comptes Rendus Acad. Sci. Paris 95, 903 and 1044, 1882.
Iyanaga, S. and Kawada, Y. (Eds.). Encyclopedic Dictionary of Mathematics. Cambridge, MA: MIT Press, p. 1461, 1980.
Picard, E. "Sur une classe de fonctions de deux variables indépendantes." Comptes Rendus Acad. Sci. Paris 90, 1119-1121, 1880a.
Picard, E. "Sur une extension aux fonctions de deux variables du problème de Riemann relatif aux fonctions hypergéométriques." Comptes Rendus Acad. Sci. Paris 90, 1267-1269, 1880b.
Picard, E. "Sur une extension aux fonctions de deux variables du problème de Riemann relatif aux fonctions hypergéométriques." Ann. Ecole Norm. Sup. (2) 10, 305-322, 1881.
Watson, G. N. "The Product of Two Hypergeometric Functions." Proc. London Math. Soc. 20, 189-195, 1922.
Whittaker, E. T. and Watson, G. N. A Course in Modern Analysis, 4th ed. Cambridge, England: Cambridge University Press, 1990.



|
|
|
|
دخلت غرفة فنسيت ماذا تريد من داخلها.. خبير يفسر الحالة
|
|
|
|
|
|
|
ثورة طبية.. ابتكار أصغر جهاز لتنظيم ضربات القلب في العالم
|
|
|
|
|
|
|
العتبة العباسية المقدسة تقدم دعوة لجامعة الحلة للمشاركة في الحفل المركزي لتخرج طلبة الجامعات
|
|
|