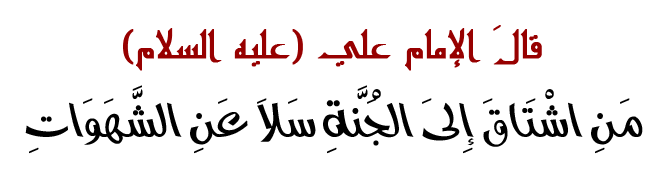
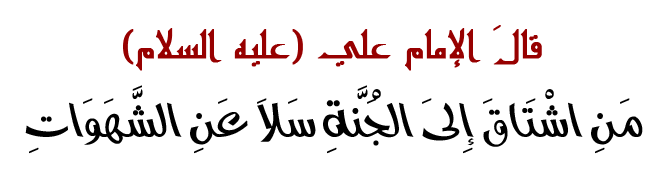

 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 27-4-2017
Date: 26-4-2017
Date: 1-5-2017
|
Died: 17 June 1956 in Victoria, Australia
David Picken's father was David Picken (born in Kilmarnock, Ayrshire about 1847) who was a schoolmaster. His mother was Margaret Picken (born in Edinburgh about 1847). He had four older siblings: Gusella (born about 1871), Robert (born about 1874), Charles (born about 1876), and Annie (born about 1877). He also had a younger brother Ralph (born about 1885).
David Picken attended school at Allan Glen's, Glasgow and then studied at Glasgow University being awarded an M.A. He then went to Cambridge to study the Mathematical Tripos and matriculated at Jesus College, Cambridge in October 1899. He was sixth wrangler in 1902. Following his graduation Picken was appointed Lecturer and Assistant Professor in the University of Glasgow. From there he moved to become Professor of Mathematics at Victoria College, Wellington, New Zealand in 1907. In 1915 he was appointed Master of Ormond College, University of Melbourne, Victoria, Australia. The College was a Presbyterian one named after its principal benefactor Francis Ormond. In fact Picken was the second master of Ormond College, the first being John MacFarland who had become Vice-Chancellor of the University in 1914.
David Kennedy Picken's obituary appeared in The Mathematical Gazette, Vol. 41, No. 337. (Oct., 1957), pp. xxvi-xxvii. We present a version of this obituary at THIS LINK.
Let us give here a few details which are not in the Gazette obituary. First let us list a few of the papers that Picken published in The Mathematical Gazette: Ratio and proportion (January 1920); The complete angle and geometrical generality (December 1922); Some general principles of analytical geometry (July 1923); The complete angle (October 1923); The notation of the calculus (October 1923); Parallelism and similarity (October 1924); The approach to the logarithmic and exponential functions (December 1926); and The approach to the calculus (October 1927). He also published in The Australian Math. Teacher,for example The Arithmetic and Algebra of the Natural Numbers (1946). Picken's book The Number System of Arithmetic and Algebra was published by Melbourne University Press in 1923.
A nice account of Picken as Master of Ormond College is given by Sir Zelman Cowen who wrote:-
My own experience is I think instructive. When I came to the University of Melbourne in 1936 my ambition, transmitted to me by my mother, was to become a barrister. She stipulated, "You're to be a boy or a barrister." Accordingly, I wished to take a straight law course and embark on the practice of the law as soon as possible. It happened that I'd won a non-resident scholarship to Ormond College in the University, and I was interviewed by the Master of that College, D K Picken, a doughty, Scots Cambridge mathematician. He threw cold water on my hopes of completing my law course as quickly as possible. He told me bluntly that this was misconceived and that I would benefit both educationally and in my personal development if I undertook a combined Arts and Law course. At the time I felt frustrated and vexed that my entry into the legal profession would be delayed for a year. But ever since then I have been deeply grateful for Picken's firm guidance. His insistence that I should broaden my university studies opened up a learning and cultural experience for me in areas in which the university was at its best. I was exposed to its outstanding teachers. It was a truly broad and liberal education and as such, it was to be of inestimable benefit to me.
Picken was a member of the Edinburgh Mathematical Society, joining in May 1903 and remaining a member during his career in New Zealand and Australia. He served on the Committee of the Society from session 1904-5, and served as editor of the Proceedings from session 1906-07. He read papers to the Society such as A Proof of the Addition Theorem in Trigonometry to the meeting on Friday 9 December 1904, On a Direct Method of Obtaining the Foci and Directrices from the General Equation of the Second Degree to the meeting on 9 June 1905, On Simson Line and Related Theory: and An Exercise in Geometric Generality (communicated by A W Young) on 8 May 1914.
He also joined the Wellington Philosophical Society when working in Wellington, New Zealand. He lectured on Spherical Geometry and Trigonometry to the Society on 11 April 1911. He was on the Council of the Philosophical Society from 1912.



|
|
|
|
تفوقت في الاختبار على الجميع.. فاكهة "خارقة" في عالم التغذية
|
|
|
|
|
|
|
أمين عام أوبك: النفط الخام والغاز الطبيعي "هبة من الله"
|
|
|
|
|
|
|
قسم شؤون المعارف ينظم دورة عن آليات عمل الفهارس الفنية للموسوعات والكتب لملاكاته
|
|
|