Nonrelativistic Electron Gas
المؤلف:
Sidney B. Cahn, Gerald D. Mahan And Boris E. Nadgorny
المصدر:
A GUIDE TO PHYSICS PROBLEMS
الجزء والصفحة:
part 2 , p 32
30-8-2016
1526
Nonrelativistic Electron Gas
a) Derive the relation between pressure and volume of a free nonrelativistic electron gas at zero temperature.
b) The formula obtained in (a) is approximately correct for sufficiently low temperatures (the so-called strongly degenerate gas). Discuss the applicability of this formula to common metals.
SOLUTION
a) As τ → 0, the Fermi-Dirac distribution function
(1)
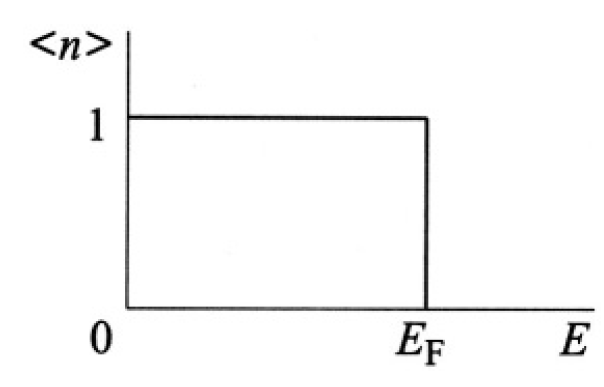
Figure 1.1
becomes a step function. All the states above a certain energy, ε > μ, are empty, and the states below, ε < μ, are filled (see Figure 1.1). This energy for an electron gas is called the Fermi energy. Physically, this results from the simple fact that the total energy of the gas should be a minimum. However, we have to reconcile this with the Pauli principle, which prohibits more than one electron per quantum state (i.e., same momentum and spin). This means that the states are filled gradually from zero energy to the limiting energy, εF. The number of states accessible to a free particle with absolute value of momentum between p and p + dp is
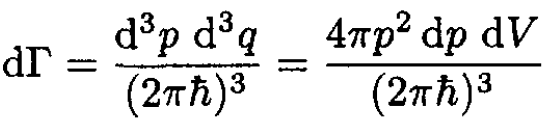(2)
In each of these states, we can put two electrons with opposite spin (up and down), so if we consider the total number of electrons, N, contained in a box of volume V, then N is given by
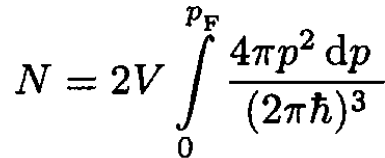(3)
Substituting p2/2m = ε, we obtain
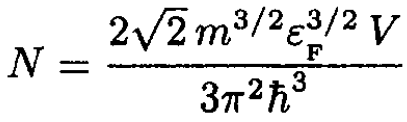(4)
and therefore
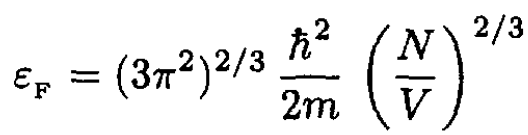(5)
To calculate the total energy of the gas, we can write
where again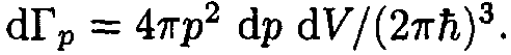 
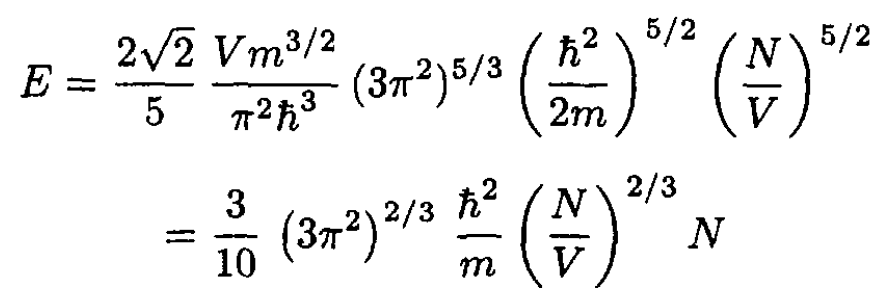(6)
and therefore
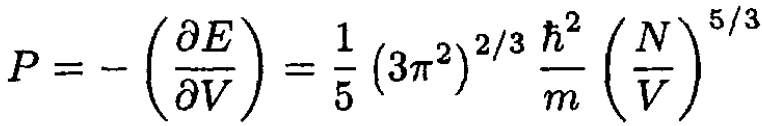(7)
and we can check that
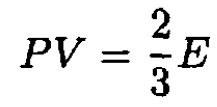(8)
b) The condition for strong degeneracy is that the temperature τ should be much smaller than the Fermi energy:
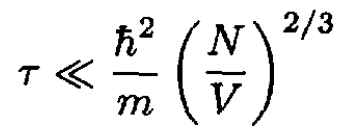(9)
For typical metals, if we assume that there is one free electron per atom and a typical interatomic distance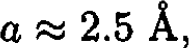 
we obtain an electron density N/V 
which indicates a Fermi energy of the order of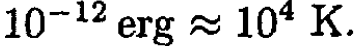 
So, most of the metals are strongly degenerate, even at room temperature.
الاكثر قراءة في مواضيع اخرى
اخر الاخبار
اخبار العتبة العباسية المقدسة
