
Electrons and Holes
 المؤلف:
Sidney B. Cahn, Gerald D. Mahan And Boris E. Nadgorny
المؤلف:
Sidney B. Cahn, Gerald D. Mahan And Boris E. Nadgorny
 المصدر:
A GUIDE TO PHYSICS PROBLEMS
المصدر:
A GUIDE TO PHYSICS PROBLEMS
 الجزء والصفحة:
part 2 , p 47
الجزء والصفحة:
part 2 , p 47
 26-8-2016
26-8-2016
 1566
1566
Electrons and Holes
a) Derive a formula for the concentration of electrons in the conduction band of a semiconductor with a fixed chemical potential (Fermi level) μ, assuming that in the conduction band ε – μ >> τ (nondegenerate electrons).
b) What is the relationship between hole and electron concentrations in a semiconductor with arbitrary impurity concentration and band gap Eg?
c) Find the concentration of electrons and holes for an intrinsic semiconductor (no impurities), and calculate the chemical potential if the electron mass is equal to the mass of the hole: me = mh.
SOLUTION
a) Let the zero of energy be the bottom of the conduction band, so μ ≤ 0 (see Figure 1.1). The number of electrons may be found from
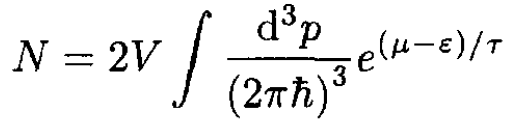 (1)
(1)
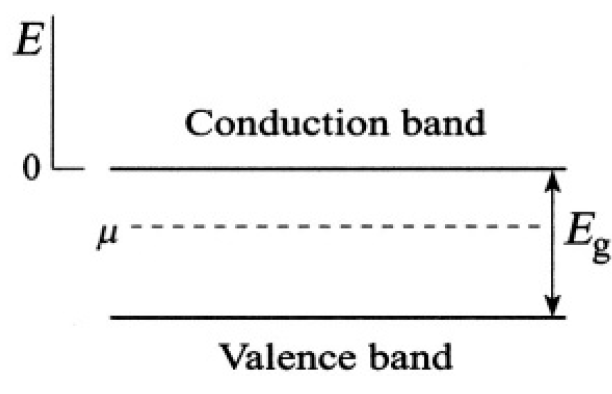
Figure 1.1
where 2 = 2s + 1 for electrons, and the Fermi distribution formula has been approximated by
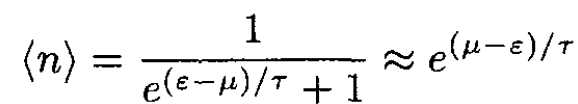 (2)
(2)
The concentration of electrons is then
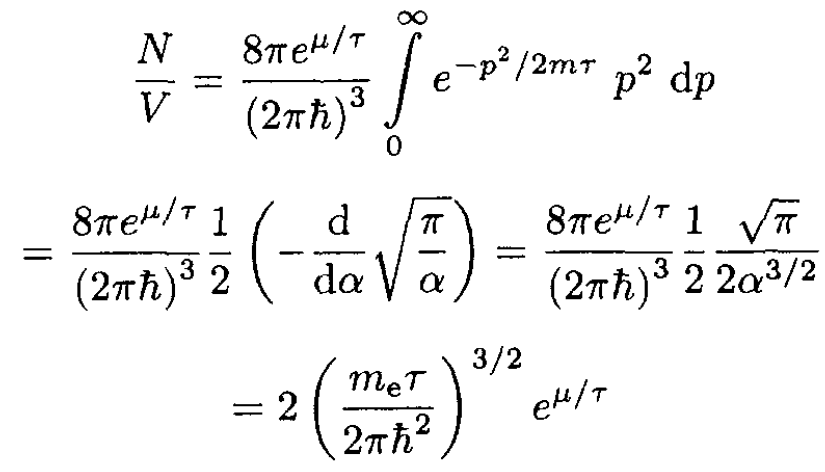 (3)
(3)
where 1/α ≡ 2mτ
b) In an intrinsic semiconductor
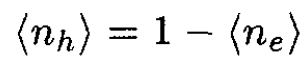 (4)
(4)
since a hole is defined as the absence of an electron. We may then write
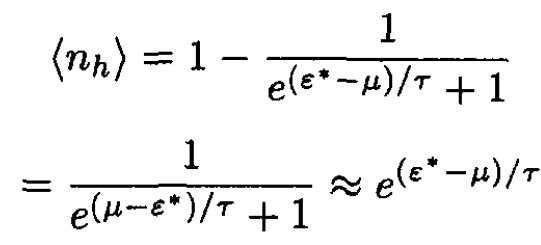 (5)
(5)
where ε* is the energy of a hole and we have used the non-degeneracy condition for holes μ – ε* >> τ. The number of holes is
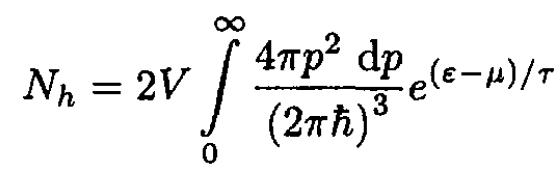 (6)
(6)
The energy of a hole (from the bottom of the conduction band) is
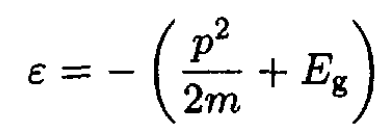 (7)
(7)
Therefore, similar to (a):
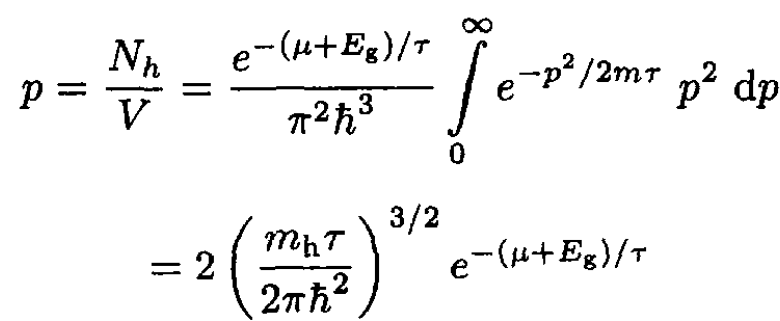 (8)
(8)
The product of the concentrations of electrons and holes does not depend on the chemical potential μ, as we see by multiplying (3) and (8):
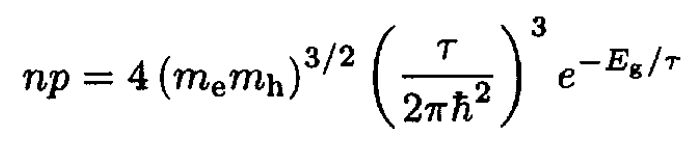 (9)
(9)
We did not use the fact that there are no impurities. The only important assumption is that μ – ε >> τ, which implies that the chemical potential μ is not too close to either the conduction or valence bands.
c) Since, in the case of an intrinsic semiconductor ni = pi (every electron in the conduction band leaves behind a hole in the valence band), we can write, using (9),
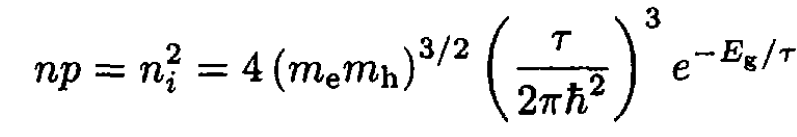 (10)
(10)
Therefore,
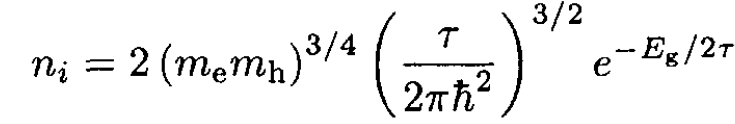 (11)
(11)
Equating (3) and (11), we can find the chemical potential for an intrinsic semiconductor:
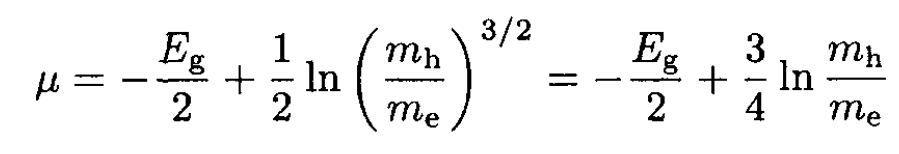 (12)
(12)
If me = mh, then the chemical potential is in the middle of the band gap:
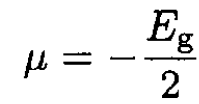
 الاكثر قراءة في مواضيع اخرى
الاكثر قراءة في مواضيع اخرى
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة


