Nonlinear Oscillator
المؤلف:
Sidney B. Cahn And Boris E. Nadgorny
المصدر:
A GUIDE TO PHYSICS PROBLEMS
الجزء والصفحة:
part 1 , p 22
28-7-2016
1596
Nonlinear Oscillator
a) A nonlinear oscillator has a potential V(x) given by
with λ a small parameter. Find the solution of the equations of motion to first order in λ, assuming x = 0 at t = 0.
b) Comment on the temperature dependence of the thermal expansion coefficient, if the interaction of the atoms in a solid is described by V(x) from (a).
SOLUTION
a) The Lagrangian for the potential V(x) is
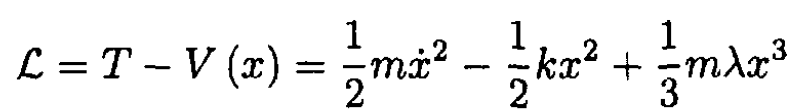
Therefore, the equation of motion for the nonlinear harmonic oscillator is
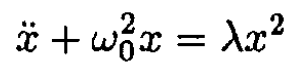(1)
where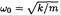 
is the principal frequency of a harmonic oscillator. We will look for a solution of the form
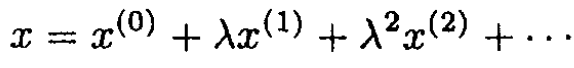(2)
Where x(0) is a solution of a harmonic oscillator equation
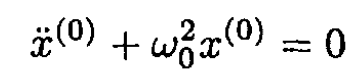(3)
Since we are looking only for the first order corrections, we do not have to consider a frequency shift in the principal frequency ω0. The solution of equation (3) with initial condition x(0) = 0 is x(0) = A sin ω0t. Substituting this into (1) and using (2), we obtain an equation for x(1) (leaving only the terms which are first order in λ).
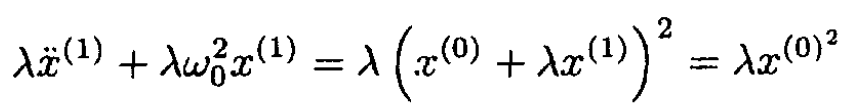(4)
or
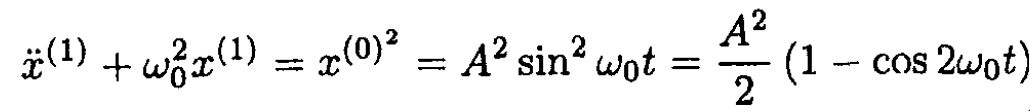(5)
The solution for x(1) is a sum of the solutions of the linear homogeneous and the linear inhomogeneous equations:
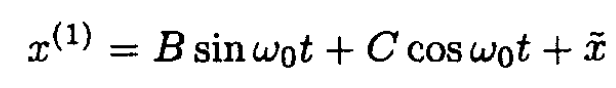(6)
where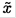
is the inhomogeneous solution of the form
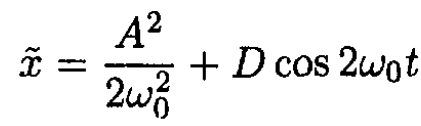(7)
Substituting (6) and (7) into (5), we obtain 
So
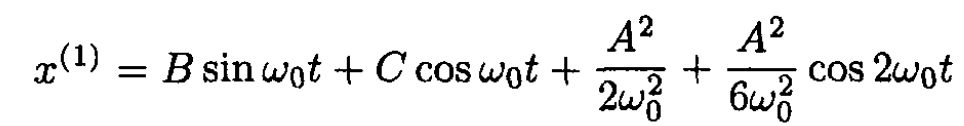(8)
Using the initial condition x(1)(0) = 0 we obtain C = -2A2/3ω20. The solution of the equation of motion (1) will be
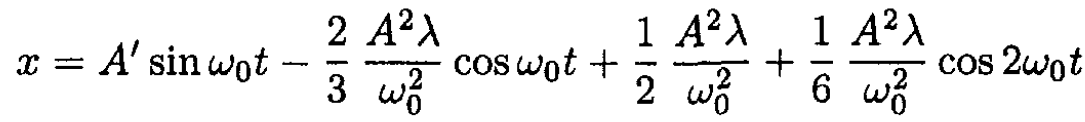(9)
where is A' defined from initial conditions.
b) The average of <x> over a period T = 2π/ω0 is certainly nonzero for a given amplitude of oscillation A. Inspection of (9) reveals that
(10)
To take into account the energy distribution of the amplitude, we have to calculate the thermodynamical average of <x> as a function of temperature
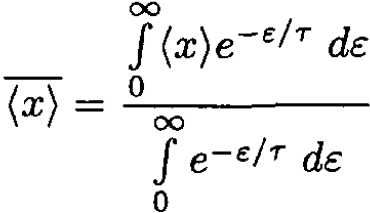(11)
where τ is the temperature in energy units and is Boltzmann’s constant.
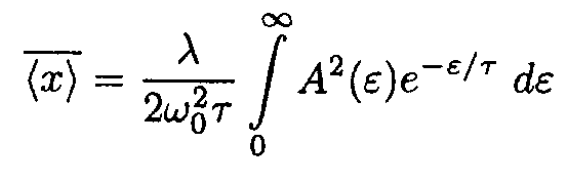(12)
The amplitude of the oscillator as a function of energy is given by
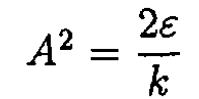(13)
Substituting (13) into (12) gives
(14)
This result can explain the nonzero thermal expansion coefficient of solids.
الاكثر قراءة في مواضيع اخرى
اخر الاخبار
اخبار العتبة العباسية المقدسة
