

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Quotient-Difference Table
المؤلف:
Conway, J. H. and Guy, R. K.
المصدر:
In The Book of Numbers. New York:Springer-Verlag
الجزء والصفحة:
...
29-11-2021
2570
Quotient-Difference Table
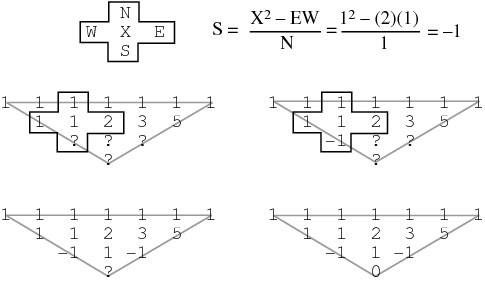
A quotient-difference table is a triangular array of numbers constructed by drawing a sequence of 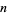 numbers in a horizontal row and placing a 1 above each. An additional "1" is then placed at the beginning and end of the row of 1s, and the value of rows underneath the original row is then determined by looking at groups of adjacent numbers
numbers in a horizontal row and placing a 1 above each. An additional "1" is then placed at the beginning and end of the row of 1s, and the value of rows underneath the original row is then determined by looking at groups of adjacent numbers
 |
(1) |
and computing
 |
(2) |
for the elements falling within a triangle formed by the diagonals extended from the first and last "1," as illustrated above.
0s in quotient-difference tables form square "windows" which are bordered by geometric sequences. Quotient-difference tables eventually yield a row of 0s iff the starting sequence is defined by a linear recurrence relation. For example, continuing the above example generated by the Fibonacci numbers
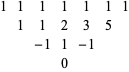 |
(3) |
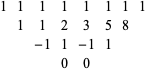 |
(4) |
 |
(5) |
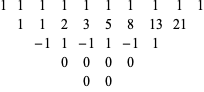 |
(6) |
and it can be seen that a row of 0s emerges (and furthermore that an attempt to extend the table will result in division by zero). This verifies that the Fibonacci numbers satisfy a linear recurrence, which is in fact given by the well-known formula
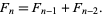 |
(7) |
However, construction of a quotient-difference table for the Catalan numbers, Motzkin numbers, etc., does not lead to a row of zeros, suggesting that these numbers cannot be generated using a linear recurrence.
REFERENCES:
Conway, J. H. and Guy, R. K. In The Book of Numbers. New York:Springer-Verlag, pp. 85-89, 1996.
Getu, S.; Shapiro, L. W.; Woan, W. J.; and Woodson, L. C. "How to Guess a Generating Function." SIAM J. Disc. Math. 5, 497-499, 1992.
Gragg, W. B. "The Padé Table and Its Relation to Certain Algorithms of Numerical Analysis." SIAM Rev. 14, 1-16, 1972.
Henrici, P. "Quotient-Difference Algorithms." In Mathematical Methods for Digital Computers, Vol. 2 (Ed. A. Ralston and H. S. Wilf). New York: Wiley, pp. 35-62, 1967.
Jones, W. B. and Thron, W. J. Continued Fractions: Analytical Theory and Applications. Reading, MA: Addison-Wesley, 1980.
Lidl, R. and Niederreiter, H. §6.6 in Introduction to Finite Fields and Their Applications, rev. ed. Cambridge, England: Cambridge University Press, 1994.
Sloane, N. J. A. and Plouffe, S. The Encyclopedia of Integer Sequences. San Diego, CA: Academic Press, pp. 15-17, 1995.
 الاكثر قراءة في الرياضيات التطبيقية
الاكثر قراءة في الرياضيات التطبيقية
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة
قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)


















