A cubic spline is a spline constructed of piecewise third-order polynomials which pass through a set of 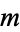 control points. The second derivative of each polynomial is commonly set to zero at the endpoints, since this provides a boundary condition that completes the system of  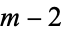equations. This produces a so-called "natural" cubic spline and leads to a simple tridiagonal system which can be solved easily to give the coefficients of the polynomials. However, this choice is not the only one possible, and other boundary conditions can be used instead.

Cubic splines are implemented in the Wolfram Language as BSplineCurve[pts, SplineDegree -> 3].

Consider 1-dimensional spline for a set of 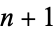 points 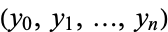. Following Bartels et al. (1998, pp. 10-13), let the 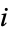th piece of the spline be represented by

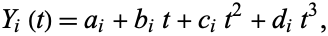(1)

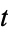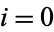where  is a parameter  and , ..., . Then

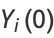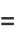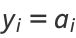			(2)
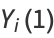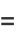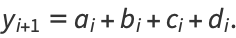			(3)

Taking the derivative of 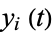 in each interval then gives

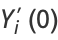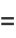			(4)
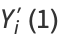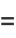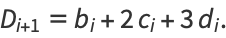			(5)

Solving (2)-(5) for 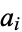, , 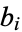, 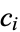and 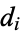 then gives

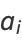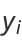			(6)
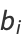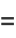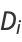			(7)
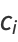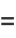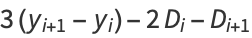			(8)
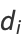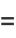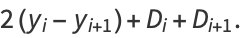			(9)

Now require that the second derivatives also match at the points, so

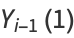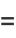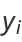			(10)
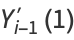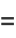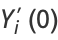			(11)
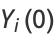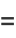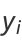			(12)
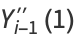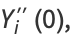			(13)

for interior points, as well as that the endpoints satisfy

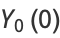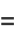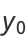			(14)
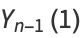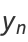			(15)

This gives a total of  equations for the 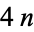 unknowns. To obtain two more conditions, require that the second derivatives at the endpoints be zero, so

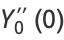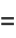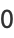			(16)
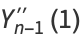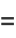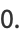			(17)

Rearranging all these equations (Bartels et al. 1998, pp. 12-13) leads to the following beautifully symmetric tridiagonal system

(18)

If the curve is instead closed, the system becomes

(19)

REFERENCES:

Bartels, R. H.; Beatty, J. C.; and Barsky, B. A. "Hermite and Cubic Spline Interpolation." Ch. 3 in An Introduction to Splines for Use in Computer Graphics and Geometric Modelling. San Francisco, CA: Morgan Kaufmann, pp. 9-17, 1998.

Burden, R. L.; Faires, J. D.; and Reynolds, A. C. Numerical Analysis, 6th ed. Boston, MA: Brooks/Cole, pp. 120-121, 1997.

Press, W. H.; Flannery, B. P.; Teukolsky, S. A.; and Vetterling, W. T. "Cubic Spline Interpolation." §3.3 in Numerical Recipes in FORTRAN: The Art of Scientific Computing, 2nd ed. Cambridge, England: Cambridge University Press, pp. 107-110, 1992.

اخر الاخبار

اخبار العتبة العباسية المقدسة

قسم الشؤون الفكرية يطلق باقة من النشاطات الرقمية الخاصة بشهر المحرم

العتبة العباسية المقدسة تختتم السلسلة الأولى لمشروع المحاضرات القرآنية العاشورائية في بابل

العتبة العباسية المقدسة تسلّم راية العزاء إلى تجمّع مواكب محافظة الديوانية

قسم الشؤون الفكرية يواصل إقامة المجالس الحسينية ضمن سلسلة (بيوت النعيم)

المزيد

الآخبار الصحية

تناول الجبن.. مفيد للجسم أم خطر يهدد صحتك؟

طبيبة أسنان تحذر.. لا تتجاهل هذه العلامة بعد التنظيف

يجب أن تأكل المزيد من الشمام هذا الصيف.. إليك السبب ؟

تأثير صحي كبير.. كم بيضة يجب أن تتناول يوميا؟

المزيد

الآخبار التكنلوجية

أداء خلايا البيروفسكايت الشمسية تحت الماء قد يزيد كفاءة تحويل الكهرباء

مسافة الأمان: أساس القيادة الآمنة وتجنب الحوادث

هل القيادة السريعة تعني استهلاك اكثر للوقود؟

لماذا يعتبر كوكب المريخ غير صالح للحياة؟

المزيد