
Hénon Map
 المؤلف:
Dickau, R. M
المؤلف:
Dickau, R. M
 المصدر:
"The Hénon Attractor." http://mathforum.org/advanced/robertd/henon.html.
المصدر:
"The Hénon Attractor." http://mathforum.org/advanced/robertd/henon.html.
 الجزء والصفحة:
...
الجزء والصفحة:
...
 19-9-2021
19-9-2021
 3039
3039
Hénon Map
There are at least two maps known as the Hénon map.
The first is the two-dimensional dissipative quadratic map given by the coupled equations
(Hénon 1976).
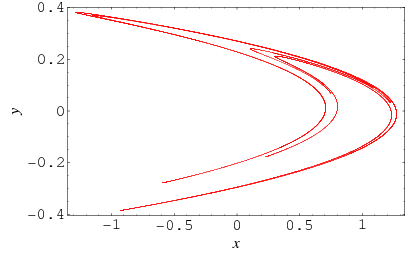
The strange attractor illustrated above is obtained for 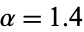 and
and 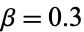 .
.

The illustration above shows two regions of space for the map with 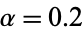 and
and 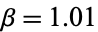 colored according to the number of iterations required to escape (Michelitsch and Rössler 1989).
colored according to the number of iterations required to escape (Michelitsch and Rössler 1989).
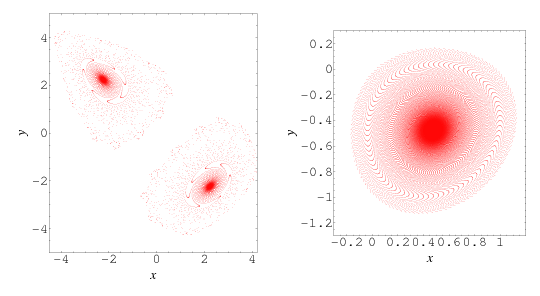
The plots above show evolution of the point 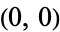 for parameters
for parameters 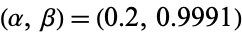 (left) and
(left) and 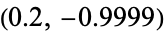 (right).
(right).
The Hénon map has correlation exponent 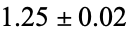 (Grassberger and Procaccia 1983) and capacity dimension
(Grassberger and Procaccia 1983) and capacity dimension  (Russell et al. 1980). Hitzl and Zele (1985) give conditions for the existence of periods 1 to 6.
(Russell et al. 1980). Hitzl and Zele (1985) give conditions for the existence of periods 1 to 6.
A second Hénon map is the quadratic area-preserving map
(Hénon 1969), which is one of the simplest two-dimensional invertible maps.
REFERENCES:
Dickau, R. M. "The Hénon Attractor." http://mathforum.org/advanced/robertd/henon.html.
Gleick, J. Chaos: Making a New Science. New York: Penguin Books, pp. 144-153, 1988.
Grassberger, P. and Procaccia, I. "Measuring the Strangeness of Strange Attractors." Physica D 9, 189-208, 1983.
Hénon, M. "Numerical Study of Quadratic Area-Preserving Mappings." Quart. Appl. Math. 27, 291-312, 1969.
Hénon, M. "A Two-Dimensional Mapping with a Strange Attractor." Comm. Math. Phys. 50, 69-77, 1976.
Hitzl, D. H. and Zele, F. "An Exploration of the Hénon Quadratic Map." Physica D 14, 305-326, 1985.
Lauwerier, H. Fractals: Endlessly Repeated Geometric Figures. Princeton, NJ: Princeton University Press, pp. 128-133, 1991.
Michelitsch, M. and Rössler, O. E. "A New Feature in Hénon's Map." Comput. & Graphics 13, 263-275, 1989. Reprinted in Chaos and Fractals, A Computer Graphical Journey: Ten Year Compilation of Advanced Research (Ed. C. A. Pickover). Amsterdam, Netherlands: Elsevier, pp. 69-71, 1998.
Morosawa, S.; Nishimura, Y.; Taniguchi, M.; and Ueda, T. "Dynamics of Generalized Hénon Maps." Ch. 7 in Holomorphic Dynamics. Cambridge, England: Cambridge University Press, pp. 225-262, 2000.
Peitgen, H.-O. and Richter, D. H. The Beauty of Fractals: Images of Complex Dynamical Systems. New York: Springer-Verlag, 1986.
Peitgen, H.-O. and Saupe, D. (Eds.). "A Chaotic Set in the Plane." §3.2.2 in The Science of Fractal Images. New York: Springer-Verlag, pp. 146-148, 1988.
Russell, D. A.; Hanson, J. D.; and Ott, E. "Dimension of Strange Attractors." Phys. Rev. Let. 45, 1175-1178, 1980.
Wells, D. The Penguin Dictionary of Curious and Interesting Geometry. London: Penguin, pp. 95-97, 1991.
 الاكثر قراءة في الرياضيات التطبيقية
الاكثر قراءة في الرياضيات التطبيقية
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة


