Hofstadter's Q-Sequence

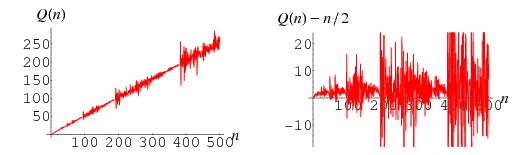
The recursive sequence generated by the recurrence equation

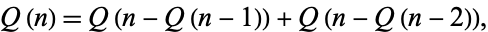with 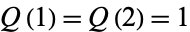. The first few values are 1, 1, 2, 3, 3, 4, 5, 5, 6, 6, ... (OEIS A005185; Wolfram 2002, pp. 129-130, sequence (e)). These numbers are sometimes called 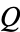-numbers. The Hofstadter 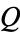-sequence can be implemented in the Wolfram Language as

Hofstadter[1] = Hofstadter[2] = 1;
Hofstadter[n_Integer?Positive] := Hofstadter[n] = Block[
   {$RecursionLimit = Infinity},
   Hofstadter[n - Hofstadter[n - 1]] +
    Hofstadter[n - Hofstadter[n - 2]]
   ]

There are currently no rigorous analyses or detailed predictions of the rather erratic behavior of 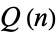 (Guy 1994). It has, however, been demonstrated that the chaotic behavior of the 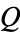-numbers shows some signs of order, namely that they exhibit approximate period doubling, self-similarity and scaling (Pinn 1999, 2000). These properties are shared with the related sequence

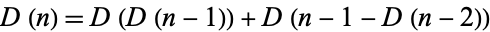with 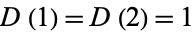, which exhibits exact period doubling (Pinn 1999, 2000). The chaotic regions of  are separated by predictable smooth behavior.

REFERENCES:

Conolly, B. W. "Fibonacci and Meta-Fibonacci Sequences." In Fibonacci and Lucas Numbers, and the Golden Section (Ed. S. Vajda). New York: Halstead Press, pp. 127-138, 1989.

Dawson, R.; Gabor, G.; Nowakowski, R.; and Weins, D. "Random Fibonacci-Type Sequences." Fib. Quart. 23, 169-176, 1985.

Guy, R. "Some Suspiciously Simple Sequences." Amer. Math. Monthly 93, 186-191, 1986.

Guy, R. K. "Three Sequences of Hofstadter." §E31 in Unsolved Problems in Number Theory, 2nd ed. New York: Springer-Verlag, pp. 231-232, 1994.

Hofstadter, D. R. Gödel, Escher Bach: An Eternal Golden Braid. New York: Vintage Books, pp. 137-138, 1980.

Kubo, T. and Vakil, R. "On Conway's Recursive Sequence." Disc. Math. 152, 225-252, 1996.

Mallows, C. L. "Conway's Challenge Sequence." Amer. Math. Monthly 98, 5-20, 1991.

Pickover, C. A. "The Crying of Fractal Batrachion ." Comput. & Graphics 19, 611-615, 1995. Reprinted in Chaos and Fractals, A Computer Graphical Journey: Ten Year Compilation of Advanced Research (Ed. C. A. Pickover). Amsterdam, Netherlands: Elsevier, pp. 127-131, 1998.

Pickover, C. A. "The Crying of Fractal Batrachion ." Ch. 25 in Keys to Infinity. New York: W. H. Freeman, pp. 183-191, 1995.

Pinn, K. "Order and Chaos is Hofstadter's 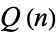 Sequence." Complexity 4, 41-46, 1999.

Pinn, K. "A Chaotic Cousin of Conway's Recursive Sequence." Exper. Math. 9, 55-66, 2000.

Sloane, N. J. A. Sequence A005185/M0438 in "The On-Line Encyclopedia of Integer Sequences."

Tanny, S. M. "A Well-Behaved Cousin of the Hofstadter Sequence." Disc. Math. 105, 227-239, 1992.

Wolfram, S. A New Kind of Science. Champaign, IL: Wolfram Media, pp. 129-130, 2002.