


 الفيزياء الكلاسيكية
الفيزياء الكلاسيكية
 الكهربائية والمغناطيسية
الكهربائية والمغناطيسية
 علم البصريات
علم البصريات
 الفيزياء الحديثة
الفيزياء الحديثة
 النظرية النسبية
النظرية النسبية
 الفيزياء النووية
الفيزياء النووية
 فيزياء الحالة الصلبة
فيزياء الحالة الصلبة
 الليزر
الليزر
 علم الفلك
علم الفلك
 المجموعة الشمسية
المجموعة الشمسية
 الطاقة البديلة
الطاقة البديلة
 الفيزياء والعلوم الأخرى
الفيزياء والعلوم الأخرى
 مواضيع عامة في الفيزياء
مواضيع عامة في الفيزياء|
Read More
Date: 18-5-2017
Date: 20-5-2017
Date: 18-9-2020
|
Mathematical Derivation
To determine the density of allowed quantum states as a function of energy, we need to consider an appropriate mathematical model. Electrons are allowed to move relatively freely in the conduction band of a semiconductor, but are confined to the crystal. As a first step, we will consider a free electron confined to a three-dimensional infinite potential well, where the potential well represents the crystal. The potential of the infinite potential well is defined as
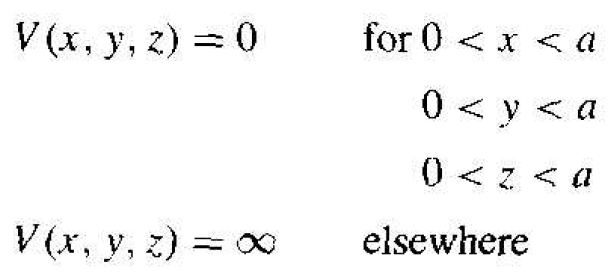 (1)
(1)
where the crystal is assumed to be a cube with length a. Schrodinger's wave equation in three dimensions can be solved using the separation of variables technique. Extrapolating the results from the one-dimensional infinite potential well, we can show that
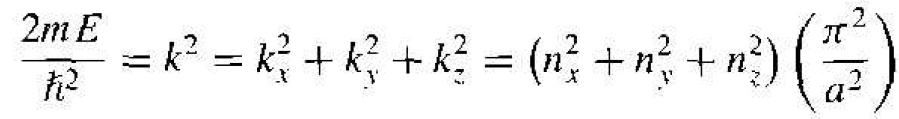 (2)
(2)
where nx, ny, and nz are positive integers. (Negative values of nx, ny, and nz yield the same wave function, except for the sign, as the positive integer values, resulting in the same probability function and energy, so the negative integers do not represent a different quantum state.)
We can schematically plot the allowed quantum states in k space. Figure 1.1a shows a two-dimensional plot as a function of kx and ky. Each point represents an allowed quantum state corresponding to various integral values of nx and ny. Positive and negative values of kx, ky, or kz have the same energy and represent the same
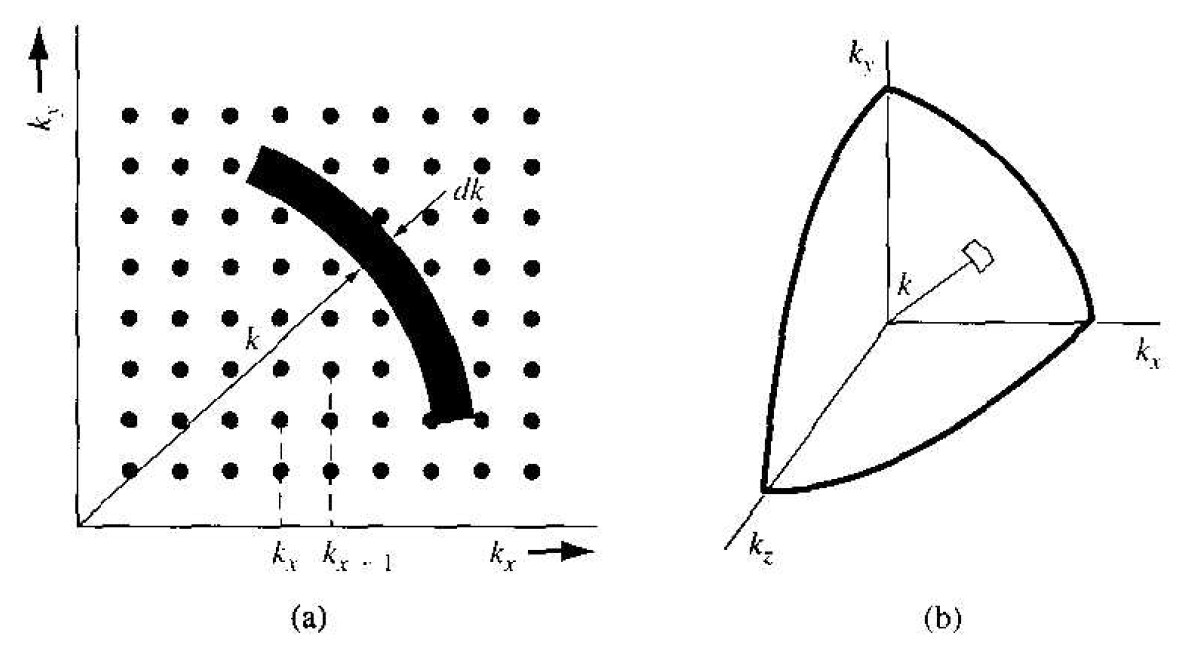
Figure 1.1 (a) A two-dimensional army of allowed quantum stales in k space. (b) The positive one-eighth of the spherical k space.
energy state. Since negative values of kx, ky, or kz do not represent additional quantum states, the density of quantum states will be determined by considering only the positive one-eighth of the spherical k space as shown in Figure 1.1b.
The distance between two quantum states in the kx direction, for example, is given by
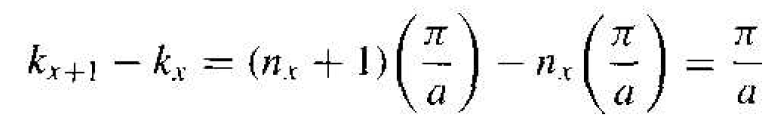 (3)
(3)
Generalizing this result to three dimensions, the volume Vk of a single quantum state is
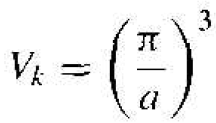 (4)
(4)
We can now determine the density of quantum states in k space. A differential volume in k space is shown in Figure 1.1b and is given by 4πk2 dk, so the differential density of quantum states in k space can he written as
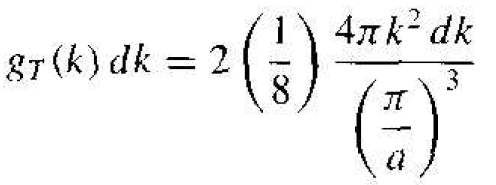 (5)
(5)
The first factor, 2, takes into account the two spin states allowed for each quantum stale; the next factor, 1/8, takes into account that we are considering only the quantum states for positive values of kx, ky, and kz. The factor 4πk2 dk, is again the differential volume and the factor (π/a)3 is the volume of one quantum state. Equation (5) may be simplified to
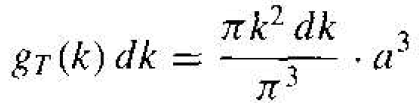 (6)
(6)
Equation (6) gives the density of quantum states as a function of momentum, through the parameter k. We can now determine the density of quantum states as a function of energy E. For a free electron, the parameters E and k are related by
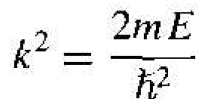 (7a)
(7a)
or
 (7b)
(7b)
The differential dk is
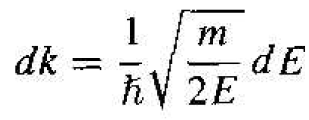 (8)
(8)
Then, substituting the expressions for k2 and dk into Equation (6). the number of energy states between E and E + dE is given by
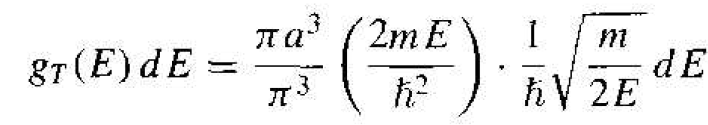 (9)
(9)
Since h = h/2π, Equation (9) becomes
 (10)
(10)
Equation (10) gives the total number of quantum states between the energy E and E + dE in the crystal space volume of a3. If we divide by the volume a3, then we will obtain the density of quantum states per unit volume of the crystal. Equation (10) then becomes
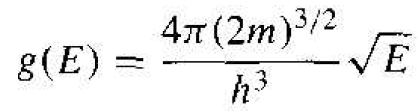 (11)
(11)
The density of quantum states is a function of energy E. As the energy of this free electron becomes small, the number of available quantum states decreases. This density function is really a double density, in that the units are given in terms of states per unit energy per unit volume.



|
|
|
|
الصين.. طريقة لمنع تطور قصر النظر لدى تلاميذ المدارس
|
|
|
|
|
|
|
ماذا سيحدث خلال كسوف الشمس يوم السبت؟
|
|
|
|
|
|
|
قسم الشؤون الدينية يختتم محاضراته الرمضانية في صحن مرقد أبي الفضل العباس (عليه السلام)
|
|
|