

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Differentiation and Integration in RN-The Darboux Integral in RN
المؤلف:
Murray H. Protter
المصدر:
Basic Elements of Real Analysis
الجزء والصفحة:
140-145
24-11-2016
966
The development of the theory of integration in RN for N ≥ 2 parallels the one-dimensional case given in Section of (The Darboux Integral for Functions on R1). For functions defined on an interval I in R1, we formed upper and lower sums by dividing I into a number of subintervals. In RN we begin with a domain F, i.e., a bounded region that has volume, and in order to form upper and lower sums, we divide F into a number of subdomains. These subdomains are the generalizations of the subinterval in R1, and the limits of the upper and lower sums as the number of subdomainstends to infinity yield upper and lower integrals. The subdomains can be thought of ashypercubesin RN with the volume of each hypercube just N times the length of a side.
Definition
Let F be a bounded set in RN that is a domain. A subdivision of F is a finite collection of domains {F1,F2,...,Fn} no two of which have common interior points and the union of which is F. That is,

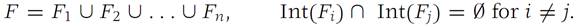
We denote such a subdivision by the single letter Δ.
Let D be a set in RN containing F and suppose that f : D → R1 is a bounded function on F. Let Δ be a subdivision of F and set


Definitions
The upper sum of f with respect to the subdivision Δ is defined by the formula


where V(Fi) is the volume in RN of the domain Fi . Similarly, the lower sum of f is define by

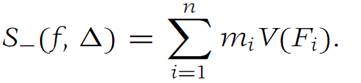
Let Δ be a subdivision of a domain F. Then Δ/, another subdivision of F, is called a refinement of Δ if every domain of Δ/ is entirely contained in one of the domains of Δ. Suppose that Δ1 ={F1,F2,...,Fn} and Δ2 ={G1,G2,...,Gm} are two subdivisions of F. We say that Δ/, the subdivision consisting of all nonempty domains of the form Fi ∩ Gj , i = 1, 2,...,n, j = 1, 2,...,m, is the common refinement of Δ1 and Δ2. Note that Δ/ is a refinement of both Δ1 and Δ2.
Theorem 1.1
Let F be a domain in RN and suppose that f :F → R1 is bounded on F.
(a) If m ≤ f(x) ≤ M for all x ∈ F and if Δ is any subdivision of F, then

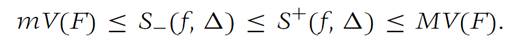
(b) If Δ/is a refinement of Δ, then

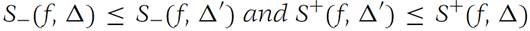
(c) If Δ1 and Δ2 are any two subdivisions of F, then

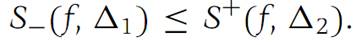
Proof
(a) The proof of part (a) is identical to the proof of part (a) in Theorem1.1 in the (The Darboux Integral for Functions on RN), the same theorem for functions from R1 into R1.
(b) Let Δ/={F/1,...,F/m} be a refinement of Δ ={F1,F2,...,Fn}.We denote by F/1,F/2,...,F/k the domains of Δ/ contained in F1. Then, using the symbols

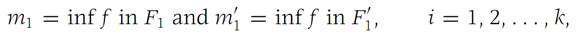
we have immediately m1 ≤ m/i, i =1, 2,...,k, since each F/I is a subset of F1.


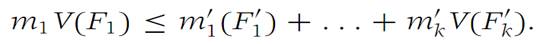
(1.1)

The same type of inequality as (1.1) holds for F2,F3,...,Fn. Summing these inequalities, we get S−(F, Δ) ≤ S−(f, Δ/). The proof that S+(f, Δ/) ≤ S+(f, Δ) is similar.
(c) If Δ1 and Δ2 are two subdivisions, let Δ/denote the common refinement. Then, from parts(a) and (b), it follows that


Definitions
Let F be a domain in RN and suppose that f : F → R1 is bounded on F. The upper integral of f is defined by


where the greatest lower bound is taken over all subdivisions Δ of F. The lower integral of f is

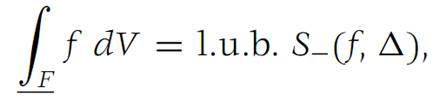
where the least upper bound is taken over all possible subdivisions Δ of F.If

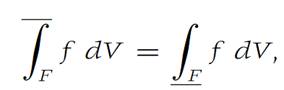
then we say that f is Darboux integrable, or just integrable, on F, and we designate the common value by

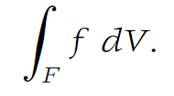
When we wish to emphasize that the integral is N-dimensional, we write

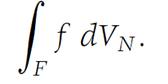
The following elementary results for integrals in RN are the direct analogues of the corresponding theorems given in Chapter of(Elementary Theory of Integration)for func- tionsfrom R1 into R1. The proofs are the same except for the necessary alterationsfrom intervals in R1 to domains in Rn.
Theorem 1.2
Let F be a domain in RN. Let f,f1,f2 be functions from F into R1 that are bounded.



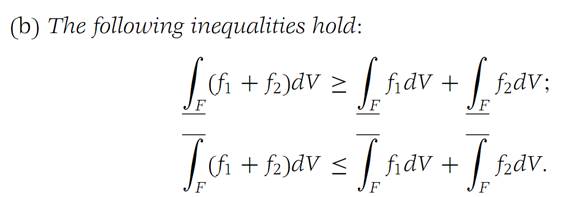

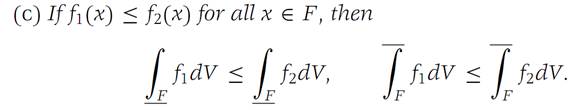
Theorem 1.3
Let F be a domain in RN and suppose that f :F → R1 is bounded.
(a) f is Darboux integrable on F if and only if for each ε> 0 there is a subdivision Δ of F such that


(b) If f is uniformly continuous on F, then F is Darboux integrable on F.
(c) If f is Darboux integrable on F, then |f | is also.
(d) If f1,f2 are each Darboux integrable on F, then f1 · f2 is also.
(e) If f is Darboux integrable on F, and H is a domain contained in F, then f is Darboux integrable on H.
The proof of Theorem 1.2 follows the lines of the analogous theorem in R1.
For positive functions from an interval I in R1 to R1, the Darboux integral gives a formula for finding the area under a curve. If F is a domain in RN, N ≥ 2, and if f : F → R1 is a nonnegative function, then the
Darboux integral of f gives the (N + 1)-dimensional volume “under the hyper surface f .”
Problems
- Let F be a domain in RN. Give an example of a function f : F → RN
such that f is bounded on F and

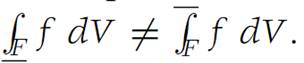
2. Suppose that F is a domain in RN and that f is integrable over F. Show that

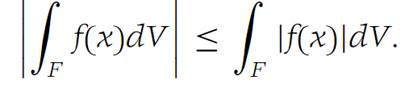
Basic Elements of Real Analysis, Murray H. Protter, Springer, 1998 .Page(140-145)
 الاكثر قراءة في التحليل الحقيقي
الاكثر قراءة في التحليل الحقيقي
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام) قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)
قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)


















