Wavelet

Wavelets are a class of a functions used to localize a given function in both space and scaling. A family of wavelets can be constructed from a function 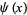, sometimes known as a "mother wavelet," which is confined in a finite interval. "Daughter wavelets"  are then formed by translation (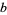) and contraction (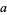). Wavelets are especially useful for compressing image data, since a wavelet transform has properties which are in some ways superior to a conventional Fourier transform.

An individual wavelet can be defined by

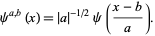(1)

Then

(2)

and Calderón's formula gives

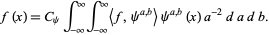(3)

A common type of wavelet is defined using Haar functions.

REFERENCES:

Benedetto, J. J. and Frazier, M. (Eds.). Wavelets: Mathematics and Applications. Boca Raton, FL: CRC Press, 1994.

Chui, C. K. An Introduction to Wavelets. San Diego, CA: Academic Press, 1992.

Chui, C. K. (Ed.). Wavelets: A Tutorial in Theory and Applications. San Diego, CA: Academic Press, 1992.

Chui, C. K.; Montefusco, L.; and Puccio, L. (Eds.). Wavelets: Theory, Algorithms, and Applications. San Diego, CA: Academic Press, 1994.

Daubechies, I. Ten Lectures on Wavelets. Philadelphia, PA: Society for Industrial and Applied Mathematics, 1992.

Erlebacher, G. H.; Hussaini, M. Y.; and Jameson, L. M. (Eds.). Wavelets: Theory and Applications. New York: Oxford University Press, 1996.

Foufoula-Georgiou, E. and Kumar, P. (Eds.). Wavelets in Geophysics. San Diego, CA: Academic Press, 1994.

Hernández, E. and Weiss, G. A First Course on Wavelets. Boca Raton, FL: CRC Press, 1996.

Hubbard, B. B. The World According to Wavelets: The Story of a Mathematical Technique in the Making, 2nd rev. upd. ed. New York: A K Peters, 1998.

Jawerth, B. and Sweldens, W. "An Overview of Wavelet Based Multiresolution Analysis." SIAM Rev. 36, 377-412, 1994.

Kaiser, G. A Friendly Guide to Wavelets. Cambridge, MA: Birkhäuser, 1994.

Massopust, P. R. Fractal Functions, Fractal Surfaces, and Wavelets. San Diego, CA: Academic Press, 1994.

Meyer, Y. Wavelets: Algorithms and Applications. Philadelphia, PA: SIAM Press, 1993.

Press, W. H.; Flannery, B. P.; Teukolsky, S. A.; and Vetterling, W. T. "Wavelet Transforms." §13.10 in Numerical Recipes in FORTRAN: The Art of Scientific Computing, 2nd ed. Cambridge, England: Cambridge University Press, pp. 584-599, 1992.

Resnikoff, H. L. and Wells, R. O. J. Wavelet Analysis: The Scalable Structure of Information. New York: Springer-Verlag, 1998.

Schumaker, L. L. and Webb, G. (Eds.). Recent Advances in Wavelet Analysis. San Diego, CA: Academic Press, 1993.

Stollnitz, E. J.; DeRose, T. D.; and Salesin, D. H. "Wavelets for Computer Graphics: A Primer, Part 1." IEEE Computer Graphics and Appl. 15, No. 3, 76-84, 1995.

Stollnitz, E. J.; DeRose, T. D.; and Salesin, D. H. "Wavelets for Computer Graphics: A Primer, Part 2." IEEE Computer Graphics and Appl. 15, No. 4, 75-85, 1995.

Strang, G. "Wavelets and Dilation Equations: A Brief Introduction." SIAM Rev. 31, 614-627, 1989.

Strang, G. "Wavelets." Amer. Sci. 82, 250-255, 1994.

Taswell, C. Handbook of Wavelet Transform Algorithms. Boston, MA: Birkhäuser, 1996.

Teolis, A. Computational Signal Processing with Wavelets. Boston, MA: Birkhäuser, 1997.

Vidakovic, B. Statistical Modeling by Wavelets. New York: Wiley, 1999.

Walker, J. S. A Primer on Wavelets and their Scientific Applications. Boca Raton, FL: CRC Press, 1999.

Walter, G. G. Wavelets and Other Orthogonal Systems with Applications. Boca Raton, FL: CRC Press, 1994.

Weisstein, E. W. "Books about Wavelets." http://www.ericweisstein.com/encyclopedias/books/Wavelets.html.

Wickerhauser, M. V. Adapted Wavelet Analysis from Theory to Software. Wellesley, MA: Peters, 1994.