


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات The Alexander polynomial is a knot invariant discovered in 1923 by J. W. Alexander (Alexander 1928). The Alexander polynomial remained the only known knot polynomial until the Jones polynomial was discovered in 1984. Unlike the Alexander polynomial, the more powerful Jones polynomial does, in most cases, distinguish handedness.
In technical language, the Alexander polynomial arises from the homology of the infinitely cyclic cover of a knot complement. Any generator of a principal Alexander ideal is called an Alexander polynomial (Rolfsen 1976). Because the Alexander invariant of a tame knot in 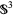 has a square presentation matrix, its Alexander ideal is principal and it has an Alexander polynomial denoted
has a square presentation matrix, its Alexander ideal is principal and it has an Alexander polynomial denoted 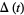 .
.
Let  be the matrix product of braid words of a knot, then
be the matrix product of braid words of a knot, then
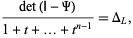 |
(1) |
where 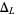 is the Alexander polynomial and det is the determinant. The Alexander polynomial of a tame knot in
is the Alexander polynomial and det is the determinant. The Alexander polynomial of a tame knot in  satisfies
satisfies
 |
(2) |
where  is a Seifert matrix, det is the determinant, and
is a Seifert matrix, det is the determinant, and 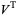 denotes the transpose.
denotes the transpose.
The Alexander polynomial is symmetric in  and
and 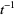 and satisfies
and satisfies
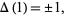 |
(3) |
where convention determines the sign. In this work, the convention 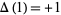 is used. The quantity
is used. The quantity  is known at the knot determinant.
is known at the knot determinant.
The notation 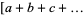 is an abbreviation for the Alexander polynomial of a knot
is an abbreviation for the Alexander polynomial of a knot
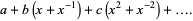 |
(4) |
The notation can also be extended for links, in which case one or more matrices is used to generate the corresponding multivariate Alexander polynomial (Rolfsen 1976, p. 389).

Let the Alexander polynomial of a link  in the variable
in the variable  be denoted
be denoted  . Then there exists a skein relationship discovered by J. H. Conway,
. Then there exists a skein relationship discovered by J. H. Conway,
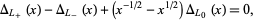 |
(5) |
corresponding to the above link diagrams (Adams 1994). This relation allows Alexander polynomials to be constructed for arbitrary knots by building them up as a sequence of over- and undercrossings.
The Alexander polynomial of a splittable link is always 0.
Surprisingly, there are known examples of nontrivial knots with Alexander polynomial 1, although no such examples occur among the knots of 10 or fewer crossings. An example is the 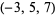 -pretzel knot (Adams 1994, p. 167). Rolfsen (1976, p. 167) gives four other such examples.
-pretzel knot (Adams 1994, p. 167). Rolfsen (1976, p. 167) gives four other such examples.
A modified version of the Alexander polynomial was formulated by J. H. Conway. It is variously known as the Conway polynomial (Livingston 1993, pp. 207-215) or Conway-Alexander polynomial, and is denoted 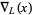 . It is a reparametrization of the Alexander polynomial given by
. It is a reparametrization of the Alexander polynomial given by
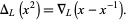 |
(6) |
The skein relationship convention used by for the Conway polynomial is
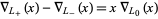 |
(7) |
(Doll and Hoste 1991).
Examples of Alexander  and Conway
and Conway 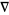 polynomials for common knots are given in the following table
polynomials for common knots are given in the following table
knot  |
 |
 |
| trefoil knot | 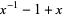 |
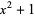 |
| figure eight knot | 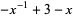 |
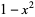 |
| Solomon's seal knot | 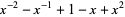 |
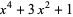 |
| stevedore's knot | 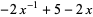 |
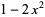 |
| Miller Institute knot |  |
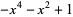 |
For a knot,
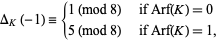 |
(8) |
where Arf is the Arf invariant (Jones 1985).
The HOMFLY polynomial 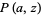 generalizes the Alexander polynomial (as well at the Jones polynomial) with
generalizes the Alexander polynomial (as well at the Jones polynomial) with
 |
(9) |
(Doll and Hoste 1991).
Rolfsen (1976) gives a tabulation of Alexander polynomials  (in abbreviated notation) for knots up to 10 crossings and links up to 9 crossings. Livingston (1993) gives an explicit table of Alexander polynomials (with negative powers cleared and initial minus sign) for knots up to 9 crossings.
(in abbreviated notation) for knots up to 10 crossings and links up to 9 crossings. Livingston (1993) gives an explicit table of Alexander polynomials (with negative powers cleared and initial minus sign) for knots up to 9 crossings.
REFERENCES:
Adams, C. C. The Knot Book: An Elementary Introduction to the Mathematical Theory of Knots. New York: W. H. Freeman, pp. 165-169, 1994.
Alexander, J. W. "Topological Invariants of Knots and Links." Trans. Amer. Math. Soc. 30, 275-306, 1928.
Alexander, J. W. "A Lemma on a System of Knotted Curves." Proc. Nat. Acad. Sci. USA 9, 93-95, 1923.
Bar-Natan, D. "The Rolfsen Knot Table." https://www.math.toronto.edu/~drorbn/KAtlas/Knots/.
Casti, J. L. "The Alexander Polynomial." Ch. 1 in Five More Golden Rules: Knots, Codes, Chaos, and Other Great Theories of 20th-Century Mathematics. New York: Wiley, pp. 1-34, 2000.
Doll, H. and Hoste, J. "A Tabulation of Oriented Links." Math. Comput. 57, 747-761, 1991.
Jones, V. "A Polynomial Invariant for Knots via von Neumann Algebras." Bull. Amer. Math. Soc. 12, 103-111, 1985.
Livingston, C. "Alexander Polynomials." Appendix 2 in Knot Theory. Washington, DC: Math. Assoc. Amer., pp. 229-232, 1993.
Murasugi, K. and Kurpita, B. I. A Study of Braids. Dordrecht, Netherlands: Kluwer, 1999.
Rolfsen, D. "Table of Knots and Links." Appendix C in Knots and Links. Wilmington, DE: Publish or Perish Press, pp. 280-287, 1976.
Stoimenow, A. "Alexander Polynomials." https://www.ms.u-tokyo.ac.jp/~stoimeno/ptab/a10.html.
Stoimenow, A. "Conway Polynomials." https://www.ms.u-tokyo.ac.jp/~stoimeno/ptab/c10.html.



|
|
|
|
التوتر والسرطان.. علماء يحذرون من "صلة خطيرة"
|
|
|
|
|
|
|
مرآة السيارة: مدى دقة عكسها للصورة الصحيحة
|
|
|
|
|
|
|
نحو شراكة وطنية متكاملة.. الأمين العام للعتبة الحسينية يبحث مع وكيل وزارة الخارجية آفاق التعاون المؤسسي
|
|
|