Polynomial Discriminant
المؤلف:
Akritas, A. G
المصدر:
Elements of Computer Algebra with Applications. New York: Wiley, 1989.
الجزء والصفحة:
...
13-2-2019
1113
Polynomial Discriminant
A polynomial discriminant is the product of the squares of the differences of the polynomial roots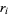 
. The discriminant of a polynomial is defined only up to constant factor, and several slightly different normalizations can be used. For a polynomial
 |
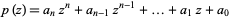(1)
|
of degree 
, the most common definition of the discriminant is
 |
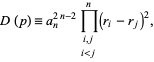(2)
|
which gives a homogenous polynomial of degree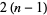 
in the coefficients of 
.
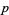The discriminant of a polynomial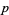 
is given in terms of a resultant as
 |
(3)
|
where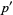 
is the derivative of 
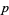and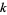 
is the degree of 
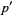. For fields of infinite characteristic, 
so the formula reduces to
 |
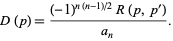(4)
|
The discriminant of a univariate polynomial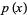 
is implemented in the Wolfram Language as Discriminant[p, x].
The discriminant of the quadratic equation
 |
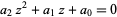(5)
|
is given by
 |
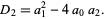(6)
|
The discriminant of the cubic equation
 |
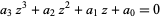(7)
|
is given by
 |
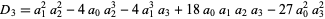(8)
|
The discriminant of a quartic equation
 |
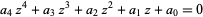(9)
|
is
 |
(10)
|
(Schroeppel 1972).
REFERENCES:
Akritas, A. G. Elements of Computer Algebra with Applications. New York: Wiley, 1989.
Basu, S.; Pollack, R.; and Roy, M.-F. Algorithms in Real Algebraic Geometry. Berlin: Springer-Verlag, 2003.
Caviness, B. F. and Johnson, J. R. (Eds.). Quantifier Elimination and Cylindrical Algebraic Decomposition. New York: Springer-Verlag, 1998.
Cohen, H. "Resultants and Discriminants." §3.3.2 in A Course in Computational Algebraic Number Theory. New York: Springer-Verlag, pp. 119-123, 1993.
Cox, D.; Little, J.; and O'Shea, D. Ideals, Varieties, and Algorithms: An Introduction to Algebraic Geometry and Commutative Algebra, 2nd ed. New York: Springer-Verlag, 1996.
Mignotte, M. and Stefănescu, D. Polynomials: An Algorithmic Approach. Singapore: Springer-Verlag, 1999.
Schroeppel, R. Item 4 in Beeler, M.; Gosper, R. W.; and Schroeppel, R. HAKMEM. Cambridge, MA: MIT Artificial Intelligence Laboratory, Memo AIM-239, p. 4, Feb. 1972. http://www.inwap.com/pdp10/hbaker/hakmem/geometry.html#item4.
Zippel, R. Effective Polynomial Computation. Boston, MA: Kluwer, 1993.
الاكثر قراءة في مواضيع عامة في الجبر
اخر الاخبار
اخبار العتبة العباسية المقدسة
