The roots (sometimes also called "zeros") of an equation

are the values of  for which the equation is satisfied.

Roots  which belong to certain sets are usually preceded by a modifier to indicate such, e.g., 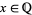 is called a rational root,  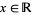is called a real root, and  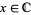is called a complex root.

The fundamental theorem of algebra states that every polynomial equation of degree 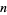 has exactly  complex roots, where some roots may have a multiplicity greater than 1 (in which case they are said to be degenerate). In the Wolfram Language, the expression Root[p(x), k] represents the 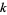th root of the polynomial 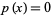, where 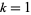, ..., 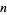is an index indicating the root number in the Wolfram Language's ordering.

The similar concept of the "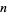th root"  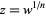of a complex number  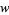is known as an nth root.

The roots of a complex function can be obtained by separating it into its real and imaginary plots and plotting these curves (which are related by the Cauchy-Riemann equations) separately. Their intersections give the complex roots of the original function. For example, the plot above shows the curves representing the real and imaginary parts of 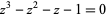, with the three roots indicated as black points.

Householder (1970) gives an algorithm for constructing root-finding algorithms with an arbitrary order of convergence. Special root-finding techniques can often be applied when the function in question is a polynomial.

REFERENCES:

Arfken, G. "Appendix 1: Real Zeros of a Function." Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 963-967, 1985.

Boyer, C. B. A History of Mathematics. New York: Wiley, 1968.

Householder, A. S. The Numerical Treatment of a Single Nonlinear Equation. New York: McGraw-Hill, 1970.

Kravanja, P. and van Barel, M. Computing the Zeros of Analytic Functions. Berlin: Springer-Verlag, 2000.

McNamee, J. M. "A Bibliography on Roots of Polynomials." J. Comput. Appl. Math. 47, 391-392, 1993.

McNamee, J. M. "A Bibliography on Roots of Polynomials." http://www.elsevier.com/homepage/sac/cam/mcnamee/.

Press, W. H.; Flannery, B. P.; Teukolsky, S. A.; and Vetterling, W. T. "Roots of Polynomials." §9.5 in Numerical Recipes in FORTRAN: The Art of Scientific Computing, 2nd ed. Cambridge, England: Cambridge University Press, pp. 362-372, 1992.

Whittaker, E. T. and Robinson, G. "The Numerical Solution of Algebraic and Transcendental Equations." Ch. 6 in The Calculus of Observations: A Treatise on Numerical Mathematics, 4th ed. New York: Dover, pp. 78-131, 1967.