Let K be the simplicial complex consisting of the triangular faces, edges and vertices of an octohedron in R³ with vertices P₁, P₂, P₃, P₄, P₅ and P₆, where

                                P₁ = (0, 0, 1), P₂ = (1, 0, 0), P₃ = (0, 1, 0),

                              P₄ = (−1, 0, 0), P₅ = (0, −1, 0), P₆ = (0, 0, −1)

This octohedron consists of the four triangular faces P₁P₂P₃, P₁P₃P₄, P₁P₄P₅ and P₁P₅P₂ of the pyramid whose base is the square P₂P₃P₄P₅ and whose apex is P₁, together with the four triangular faces P₆P₂P₃, P₆P₃P₄, P₆P₄P₅ and P₆P₅P₂ of the pyramid whose base is P₂P₃P₄P₅ and whose apex is P₆.

A typical 2-chain c₂ of K is a linear combination, with integer coefficients, of eight oriented 2-simplices that represent the triangular faces of the octohedron. Thus we can write

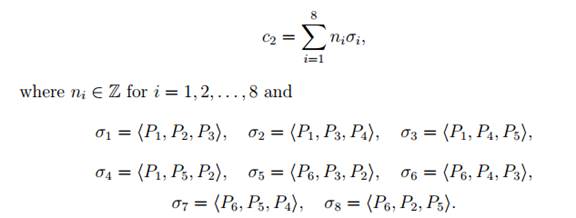
(The orientation on each of these triangles has been chosen such that the vertices of the triangle are listed in anticlockwise order when viewed from a point close to the centre of triangle that lies outside the octohedron.)  Similarly a typical 1-chain c1 of K is a linear combination, with integer coefficients, of twelve 1-simplices that represent the edges of the octohedron.

Thus we can write

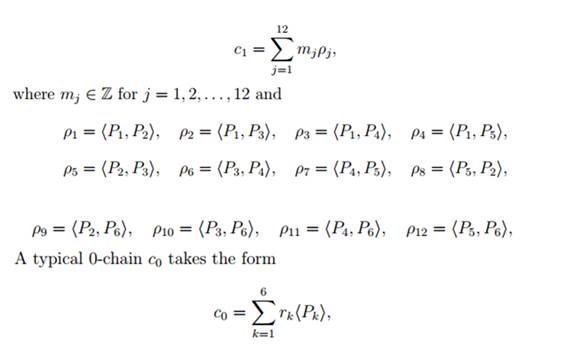
where r_k ∈ Z for k = 1, 2, . . . , 6.

We now calculate the boundary of a 2-chain. It follows from the definition of the boundary homomorphism ∂₂ that

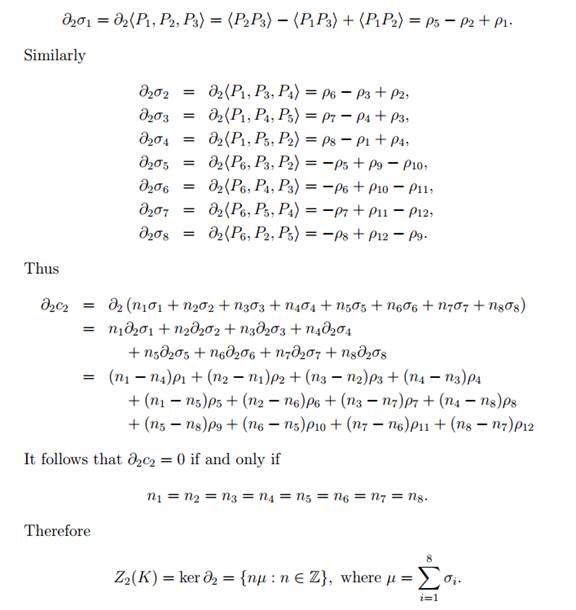
Now C₃(K) = 0, and thus B₂(K) = 0 (where 0 here denotes the zero group),  since the complex K has no 3-simplices. Therefore H₂(K) ∼= Z₂(K) ∼= Z.

Next we calculate the boundary of a 1-chain. It follows from the definition of the boundary homomorphism ∂₁ that

On examining the structure of these equations, we see that, when c₁ is a 1- cycle, it is possible to eliminate five of the integer quantities m_j , expressing

them in terms of the remaining quantities. For example, we can eliminate m₄, m₆, m₇, m₈ and m₁₂, expressing these quantities in terms of m₁, m₂, m₃,  m₅, m₉ m₁₀ and m₁₁ by means of the equations

                    m₄ = −m₁ − m₂ − m₃,

                   m₆ = m₂ − m₁₀ + m₅,

                  m₇ = m₂ + m₃ − m₁₀ − m₁₁ + m₅,

                  m₈ = −m₁ + m₉ + m₅,

                m₁₂ = −m₉ − m₁₀ − m₁₁

It follows that

Z₂(K) = {m₁z₁ + m₂z₂ + m₃z₃ + m₅z₅ + m₉z₉ + m₁₀z₁₀ + m₁₁z₁₁},

where

            z₁ = ρ₁ − ρ₄ − ρ₈ = −∂₂σ₄,

            z₂ = ρ₂ − ρ₄ + ρ₆ + ρ₇ = ∂₂(σ₂ + σ₃),

            z₃ = ρ₃ − ρ₄ + ρ₇ = ∂₂σ₃,

           z₅ = ρ₅ + ρ₆ + ρ₇ + ρ₈ = ∂₂(σ₁ + σ₂ + σ₃ + σ₄),

            z₉ = ρ₈ + ρ₉ − ρ₁₂ = −∂₂σ₈,

            z₁₀ = −ρ₆ − ρ₇ + ρ₁₀ − ρ₁₂ = ∂₂(σ₆ + σ₇),

            z₁₁ = ρ₁₁ − ρ₇ − ρ₁₂ = ∂₂σ₇.

From these equations, we see that the generators z₁, z₂, z₃, z₅, z₉, z₁₀ and z₁₁ of the group Z₁(K) of 1-cycles all belong to the group B₁(K) of 1-boundaries.

It follows that Z₁(K) = B₁(K), and therefore H₁(K) = 0.

In order to determine H₀(K) it suffices to note that the 0-chains

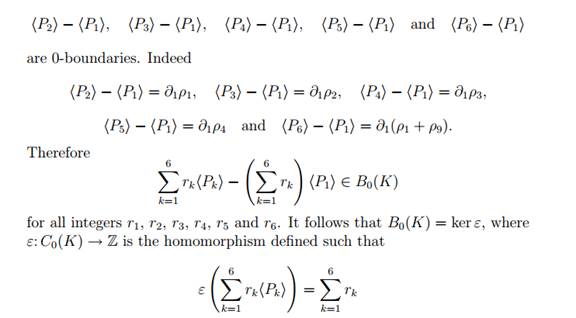
for all integers r_k (k = 1, 2, . . . , 6). Now Z₀(K) = C₀(K) since the homomorphism ∂₀: C₀(K) → C₋₁(K) is the zero homomorphism mapping C₀(K)  to the zero group. It follows that

                                       H₀(K) = C₀(K)/B₀(K) = C₀(K)/ ker ε ≅Z.

(Here we are using the result that the image of a homomorphism is isomorphic to the quotient of the domain of the homomorphism by the kernel of the homomorphism.)

We have thus shown that

                      H₂(K)≅Z, H₁(K) = 0, H₀(K) ≅ Z.

One can show that Z₁(K) = B₁(K) by employing an alternative approach to that used above. An element z of Z1(K) is of the form z =∑¹²_j=1 m_jρ_j, where

                      m₁ + m₂ + m₃ + m₄ = 0, m₁ − m₅ + m₈ − m₉ = 0,

                     m₂ + m₅ − m₆ − m₁₀ = 0, m₃ + m₆ − m₇ − m₁₁ = 0,

                   m₄ + m₇ − m₈ − m₁₂ = 0 and m₉ + m₁₀ + m₁₁ + m₁₂ = 0.

The 1-cycle z belongs to the group B₁(K) if and only if there exists some 2-chain c₂ such that z = ∂₂c₂. It follows that z ∈ B₁(K) if and only if there exist integers n₁, n₂, . . . , n₈ such that

                   m₁ = n₁ − n₄, m₂ = n₂ − n₁, m₃ = n₃ − n₂, m₄ = n₄ − n₃,

                        m₅ = n₁ − n₅, m₆ = n₂ − n₆, m₇ = n₃ − n₇, m₈ = n₄ − n₈,

                    m₉ = n₅ − n₈, m₁₀ = n₆ − n₅, m₁₁ = n₇ − n₆, m₁₂ = n₈ − n₇.

The integers n₁, n₂, . . . , n₈ solving the above equations are not uniquely determined,  since, given one collection of integers n₁, n₂, . . . , n₈ satisfying these equations, another solution can be obtained by adding some fixed integer to each of n₁, n₂, . . . , n₈. It follows from this that if there exists some collection n₁, n₂, . . . , n₈ of integers that solves the above equations, then there exists a solution which satisfies the extra condition n1 = 0. We then find that

                   n₁ = 0, n₂ = m₂, n₃ = m₂ + m₃, n₄ = −m₁,

             n₅ = −m₅, n₆ = m₂ − m₆, n₇ = m₂ + m₃ − m₇, n₈ = −m₁ − m₈.

On substituting n₁, n₂, . . . , n₈ into the relevant equations, and making use of the constraints on the values of m₁, m₂, . . . , m₁₂, we find that we do indeed have a solution to the equations that express the integers m_j in terms of the integers n_i. It follows that every 1-cycle of K is a 1-boundary. Thus Z₁(K) = B₁(K), and therefore H₁(K) = 0.