Grassmannian
المؤلف:
Fulton, W.
المصدر:
Schubert Varieties and Degeneracy Loci. New York: Springer-Verlag, 1998.
الجزء والصفحة:
...
7-7-2021
1936
Grassmannian
The Grassmannian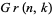 
is the set of 
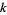-dimensional subspaces in an 
-dimensional vector space. For example, the set of lines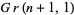 
is projective space. The real Grassmannian (as well as the complex Grassmannian) are examples of manifolds. For example, the subspace 
has a neighborhood 
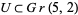. A subspace 
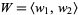is in 
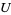if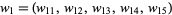 
and 
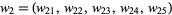and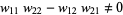 
. Then for any 
, the vectors 
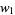and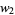 
are uniquely determined by requiring 
and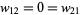 
. The other six entries provide coordinates for 
.
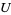In general, the Grassmannian can be given coordinates in a similar way at a point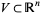 
. Let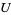 
be the open set of 
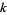-dimensional subspaces which project onto 
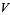. First one picks an orthonormal basis 
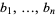for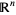 
such that 
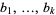span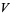 
. Using this basis, it is possible to take any 
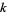vectors and make a 
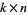matrix. Doing this for the basis of 
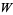, another 
-dimensional subspace in 
, gives a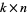 
-matrix, which is well-defined up to linear combinations of the rows. The final step is to row-reduce so that the first 
block is the identity matrix. Then the last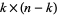 
block is uniquely determined by 
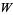. The entries in this block give coordinates for the open set 
.
If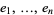 
is the standard basis of 
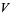, a basis of 
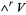is given by the 
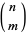vectors 
, 
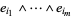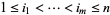. If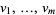 
is a basis of a subspace 
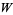of dimension 
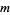of 
, 
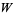corresponds to a point 
of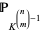 
, whose coordinates are the components of 
with respect to the basis of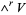 
given above. These coordinates are the so-called Grassmann coordinates of 
.
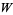A different choice of the basis of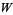 
yields a different 
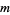-tuple of coordinates, which differs from the original 
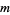-tuple by a nonzero multiplicative constant, hence it corresponds to the same point.
The Grassmannian is also a homogeneous space. A subspace is determined by its basis vectors. The group that permutes basis vectors is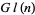 
. The matrix that fixes 
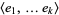is a diagonal block matrix, with a 
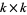nonsingular matrix in the top left, and a 
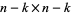invertible matrix in the lower right. 
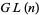acts transitively on the Grassmannian 
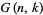. Let 
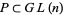be the stabilizer (or isotropy) of 
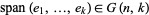. Then 
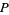is the subgroup of 
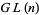consisting of matrices ![A=[a_(i,j)]](https://mathworld.wolfram.com/images/equations/Grassmannian/Inline65.gif)
such that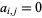 
for all 
, 
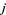such that 
and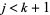 
. 
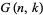is isomorphic to 
.
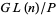The tangent space to the Grassmannian is given by 
matrices, i.e., linear maps from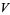 
to the quotient vector space 
.
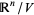The elements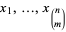 
are the 
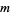-minors of the 
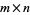matrix whose 
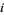th row contains the components of 
with respect to the basis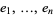 
. It corresponds to a linear transformation 
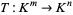whose range is 
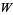. In general, the range of such a linear transformation has dimension 
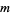iff the corresponding 
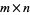matrix has rank 
.
Let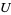 
be the subset of 
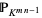defined by the condition that all the 
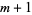-minors of the matrix 
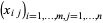(which can be viewed as a sequence of 
coordinates) be equal to zero, and one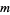 
-minor be nonzero. The Grassmannian 
can be viewed as the image of the map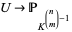 
which maps each matrix of 
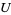to the sequence of its 
-minors.
It as an algebraic projective algebraic variety defined by equations called Plücker's equations. It is a nonsingular variety of dimension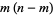 
.
REFERENCES:
Fulton, W. Schubert Varieties and Degeneracy Loci. New York: Springer-Verlag, 1998.
Harris, J. "Grassmannians and Related Varieties." Lecture 6 in Algebraic Geometry: A First Course. New York: Springer-Verlag, pp. 63-71, 1992.
Kleiman, S. and Laksov, D. "Schubert Calculus." Amer. Math. Monthly 79, 1061-1082, 1972.
Shafarevich, I. R. Basic Algebraic Geometry, Vol. 1, 2nd ed. Berlin: Springer-Verlag, pp. 42-44, 1994.
الاكثر قراءة في التبلوجيا
اخر الاخبار
اخبار العتبة العباسية المقدسة
