A 2-variable oriented knot polynomial 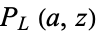 motivated by the Jones polynomial (Freyd et al. 1985). Its name is an acronym for the last names of its co-discoverers: Hoste, Ocneanu, Millett, Freyd, Lickorish, and Yetter (Freyd et al. 1985). Independent work related to the HOMFLY polynomial was also carried out by Prztycki and Traczyk (1987). HOMFLY polynomial is defined by the skein relationship

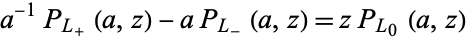(1)

(Doll and Hoste 1991), where 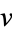 is sometimes written instead of  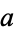(Kanenobu and Sumi 1993) or, with a slightly different relationship, as

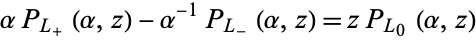(2)

(Kauffman 1991). It is also defined as 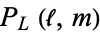 in terms of skein relationship

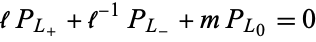(3)

(Lickorish and Millett 1988). It can be regarded as a nonhomogeneous polynomial in two variables or a homogeneous polynomial in three variables. In three variables the skein relationship is written

(4)

It is normalized so that 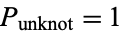. Also, for  unlinked unknotted components,

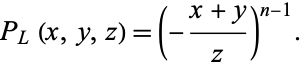(5)

This polynomial usually detects chirality but does not detect the distinct enantiomers of the knots 09-042, 10-048, 10-071, 10-091, 10-104, and 10-125 (Jones 1987). The HOMFLY polynomial of an oriented knot is the same if the orientation is reversed. It is a generalization of the Jones polynomial 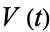, satisfying

			(6)
			(7)

It is also a generalization of the Alexander polynomial 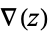, satisfying

(8)

The HOMFLY polynomial of the mirror image 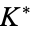 of a knot  is given by

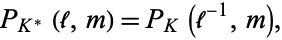(9)

so 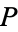 usually but not always detects chirality.

A split union of two links (i.e., bringing two links together without intertwining them) has HOMFLY polynomial

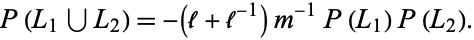(10)

Also, the composition of two links

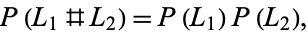(11)

so the polynomial of a composite knot factors into polynomials of its constituent knots (Adams 1994).

Mutants have the same HOMFLY polynomials. In fact, there are infinitely many distinct knots with the same HOMFLY polynomial (Kanenobu 1986). Examples include (05-001, 10-132), (08-008, 10-129) (08-016, 10-156), and (10-025, 10-056) (Jones 1987). Incidentally, these also have the same Jones polynomial.

M. B. Thistlethwaite has tabulated the HOMFLY polynomial for knots up to 13 crossings.

REFERENCES:

Adams, C. C. The Knot Book: An Elementary Introduction to the Mathematical Theory of Knots. New York: W. H. Freeman, pp. 171-172, 1994.

Doll, H. and Hoste, J. "A Tabulation of Oriented Links." Math. Comput. 57, 747-761, 1991.

Freyd, P.; Yetter, D.; Hoste, J.; Lickorish, W. B. R.; Millett, K.; and Oceanu, A. "A New Polynomial Invariant of Knots and Links." Bull. Amer. Math. Soc. 12, 239-246, 1985.

Jones, V. "Hecke Algebra Representations of Braid Groups and Link Polynomials." Ann. Math. 126, 335-388, 1987.

Kanenobu, T. "Infinitely Many Knots with the Same Polynomial." Proc. Amer. Math. Soc. 97, 158-161, 1986.

Kanenobu, T. and Sumi, T. "Polynomial Invariants of 2-Bridge Knots through 22 Crossings." Math. Comput. 60, 771-778 and S17-S28, 1993.

Kauffman, L. H. Knots and Physics. Singapore: World Scientific, p. 52, 1991.

Lickorish, W. B. R. and Millett, B. R. "The New Polynomial Invariants of Knots and Links." Math. Mag. 61, 1-23, 1988.

Livingston, C. Knot Theory. Washington, DC: Math. Assoc. Amer., pp. 213-217, 1993.

Morton, H. R. and Short, H. B. "Calculating the 2-Variable Polynomial for Knots Presented as Closed Braids." J. Algorithms 11, 117-131, 1990.

Przytycki, J. and Traczyk, P. "Conway Algebras and Skein Equivalence of Links." Proc. Amer. Math. Soc. 100, 744-748, 1987.

Stoimenow, A. "Jones Polynomials." https://www.ms.u-tokyo.ac.jp/~stoimeno/ptab/j10.html.

اخر الاخبار

اخبار العتبة العباسية المقدسة

المجمَع العلمي يقيم مسابقة الكوثر الخاصة بترتيل القرآن الكريم في كربلاء

قسم الشؤون الفكرية والثقافية يُصدر العدد الثالث والعشرين من مجلّة (أوراق معرفية)

قسم التربية والتعليم يُطلق الموسم التدريبيّ الصيفيّ لملاكات مجموعة العميد التربوية

المجمَع العلمي يُطلق المرحلة الثالثة من مشروع أمير القرّاء الوطني

المزيد

الآخبار الصحية

اختراق علمي.. دواء يؤخر ظهور السكري من النوع الأول لسنوات

هذا ما يحدث لجسمك إن أهملت نظافة فراشك

لماذا تعتبر النساء أكثر عرضة للإصابة بألزهايمر من الرجال؟

لماذا يحذر الخبراء من الإفراط في تناول الفراولة؟

المزيد

الآخبار التكنلوجية

السيارات الكهربائية أنظف بنسبة 73% من سيارات الوقود

سيارة لوسِد الكهربائية تدخل "غينيس" بأطول مدى في العالم

نظرية جديدة تنسف كل ما نعرفه عن تشكل أول قارة في الأرض

منذ 37 ألف عام.. دراسة تكشف "أقدم أمراض" أصابت البشر

المزيد