Highly Composite Number

Highly composite numbers are numbers such that divisor function 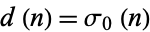 (i.e., the number of divisors of ) is greater than for any smaller 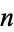. Superabundant numbers are closely related to highly composite numbers, and the first 19 superabundant and highly composite numbers are the same.

There are an infinite number of highly composite numbers, and the first few are 1, 2, 4, 6, 12, 24, 36, 48, 60, 120, 180, 240, 360, 720, 840, 1260, 1680, 2520, 5040, ... (OEIS A002182). The corresponding numbers of divisors are 1, 2, 3, 4, 6, 8, 9, 10, 12, 16, 18, 20, 24, 30, 32, ... (OEIS A002183). Ramanujan (1915) listed 102 highly composite numbers up to 6746328388800, but omitted 293318625600. Robin (1983) gives the first 5000 highly composite numbers, and a comprehensive survey is given by Nicholas (1988). Flammenkamp gives a list of the first 779674 highly composite numbers.

If

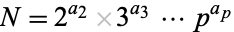(1)

is the prime factorization of a highly composite number, then

1. The primes 2, 3, ..., 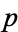 form a string of consecutive primes,

2. The exponents are nonincreasing, so 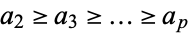, and

3. The final exponent 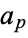 is always 1, except for the two cases  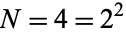and 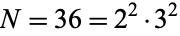, where it is 2.

Let 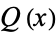 be the number of highly composite numbers 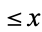. Ramanujan (1915) showed that

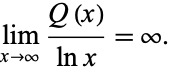(2)

Alaoglu and Erdős (1944) showed that there exists a constant  such that

(3)

Nicholas proved that there exists a constant 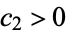 such that

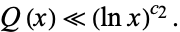(4)

REFERENCES:

Alaoglu, L. and Erdős, P. "On Highly Composite and Similar Numbers." Trans. Amer. Math. Soc. 56, 448-469, 1944.

Andree, R. V. "Ramanujan's Highly Composite Numbers." Abacus 3, 61-62, 1986.

Berndt, B. C. Ramanujan's Notebooks, Part IV. New York: Springer-Verlag, p. 53, 1994.

Dickson, L. E. History of the Theory of Numbers, Vol. 1: Divisibility and Primality. New York: Dover, p. 323, 2005.

Flammenkamp, A. "Highly Composite Numbers." https://wwwhomes.uni-bielefeld.de/achim/highly.html.

Hoffman, P. The Man Who Loved Only Numbers: The Story of Paul Erdős and the Search for Mathematical Truth. New York: Hyperion, pp. 88-91, 1998.

Honsberger, R. Mathematical Gems I. Washington, DC: Math. Assoc. Amer., p. 112, 1973.

Honsberger, R. "An Introduction to Ramanujan's Highly Composite Numbers." Ch. 14 in Mathematical Gems III. Washington, DC: Math. Assoc. Amer., pp. 193-207, 1985.

Kanigel, R. The Man Who Knew Infinity: A Life of the Genius Ramanujan. New York: Washington Square Press, p. 232, 1991.

Nicholas, J.-L. "On Highly Composite Numbers." In Ramanujan Revisited: Proceedings of the Centenary Conference, University of Illinois at Urbana-Champaign, June 1-5, 1987 (Ed. G. E. Andrews, B. C. Berndt, and R. A. Rankin). Boston, MA: Academic Press, pp. 215-244, 1988.

Ramanujan, S. "Highly Composite Numbers." Proc. London Math. Soc. 14, 347-409, 1915.

Ramanujan, S. Collected Papers of Srinivasa Ramanujan (Ed. G. H. Hardy, P. V. S. Aiyar, and B. M. Wilson). Providence, RI: Amer. Math. Soc., 2000.

Robin, G. "Méthodes d'optimalisation pour un problème de théories des nombres." RAIRO Inform. Théor. 17, 239-247, 1983.

Séroul, R. "Highly Composite Numbers." §8.14 in Programming for Mathematicians. Berlin: Springer-Verlag, pp. 208-213, 2000.

Siano, D. "Highly Composite Numbers: How Can We Calculate Them?" https://www.eclipse.net/~dimona/juliannum.html.

Siano, D. B. and Siano, J. D. "An Algorithm for Generating Highly Composite Numbers." https://wwwhomes.uni-bielefeld.de/achim/julianmanuscript3.pdf. October 7, 1994.

Sloane, N. J. A. Sequences A002182/M1025 and A002183/M0546 in "The On-Line Encyclopedia of Integer Sequences."

Wells, D. The Penguin Dictionary of Curious and Interesting Numbers. New York: Penguin Books, p. 128, 1986.