

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Thue-Morse Constant
المؤلف:
Allouche, J. P.; Arnold, A.; Berstel, J.; Brlek, S.; Jockusch, W.; Plouffe, S.; and Sagan, B.
المصدر:
"A Relative of the Thue-Morse Sequence." Discr. Math. 139
الجزء والصفحة:
...
3-2-2021
2339
Thue-Morse Constant
The Thue-Morse constant, also called the parity constant, is given by the concatenated digits of the Thue-Morse sequence
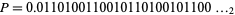 |
(1) |
(OEIS A010060) interpreted as a binary number. In, decimal, it can be written as
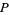 |
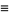 |
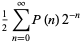 |
(2) |
 |
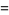 |
 |
(3) |
(OEIS A014571), where 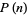 is the parity of
is the parity of 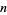 (i.e., the numbers of 1s in the binary representation of
(i.e., the numbers of 1s in the binary representation of 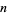 , computed modulo 2).
, computed modulo 2).
Dekking (1977) proved that the Thue-Morse constant is transcendental, and Allouche and Shallit give a complete proof correcting a minor error of Dekking.
The Thue-Morse constant can be written in base 2 by stages by taking the previous iteration 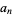 , taking the complement
, taking the complement 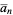 obtained by reversing the digits of
obtained by reversing the digits of 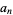 , and appending, producing
, and appending, producing
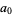 |
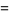 |
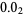 |
(4) |
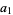 |
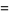 |
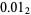 |
(5) |
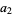 |
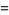 |
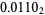 |
(6) |
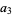 |
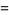 |
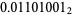 |
(7) |
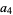 |
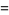 |
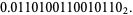 |
(8) |
This can be written symbolically as
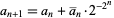 |
(9) |
with  . Here, the complement is the number
. Here, the complement is the number 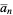 such that
such that 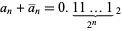 , which can be found from
, which can be found from
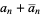 |
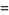 |
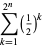 |
(10) |
 |
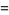 |
 |
(11) |
 |
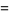 |
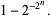 |
(12) |
Therefore,
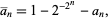 |
(13) |
and
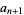 |
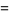 |
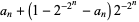 |
(14) |
 |
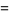 |
 |
(15) |
The first few iterations give 0, 1/4, 3/8, 105/256, 13515/32768, ... (OEIS A074072 and A074073).
The regular continued fraction for the Thue-Morse constant is [0 2 2 2 1 4 3 5 2 1 4 2 1 5 44 1 4 1 2 4 1 1 1 5 14 1 50 15 5 1 1 1 4 2 1 4 1 43 1 4 1 2 1 3 16 1 2 1 2 1 50 1 2 424 1 2 5 2 1 1 1 5 5 2 22 5 1 1 1 1274 3 5 2 1 1 1 4 1 1 15 154 7 2 1 2 2 1 2 1 1 50 1 4 1 2 867374 1 1 1 5 5 1 1 6 1 2 7 2 1650 23 3 1 1 1 2 5 3 84 1 1 1 1284 ...] (OEIS A014572), and seems to continue with sporadic large terms in suspicious-looking patterns. A nonregular continued fraction is
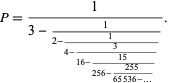 |
(16) |
A related infinite product is
 |
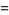 |
![1/4[2-product_(n=0)^(infty)(2^(2^n)-1)/(2^(2^n))]](https://mathworld.wolfram.com/images/equations/Thue-MorseConstant/Inline48.gif) |
(17) |
 |
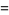 |
![1/4[2-product_(n=0)^(infty)2^(-2^n)(2^(2^n)-1)]](https://mathworld.wolfram.com/images/equations/Thue-MorseConstant/Inline51.gif) |
(18) |
 |
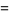 |
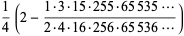 |
(19) |
(Finch 2003, p. 437).
REFERENCES:
Allouche, J. P.; Arnold, A.; Berstel, J.; Brlek, S.; Jockusch, W.; Plouffe, S.; and Sagan, B. "A Relative of the Thue-Morse Sequence." Discr. Math. 139, 455-461, 1995.
Allouche, J. P. and Shallit, J. "The Ubiquitous Prouhet-Thue-Morse Sequence." https://www.math.uwaterloo.ca/~shallit/Papers/ubiq.ps.
Dekking, F. M. "Transcendence du nombre de Thue-Morse." Comptes Rendus de l'Academie des Sciences de Paris 285, 157-160, 1977.
Finch, S. R. "Prouhet-Thue-Morse Constant." §6.8 in Mathematical Constants. Cambridge, England: Cambridge University Press, pp. 436-441, 2003.
Goldstein, S.; Kelly, K. A.; and Speer, E. R. "The Fractal Structure of Rarefied Sums of the Thue-Morse Sequence." J. Number Th. 42, 1-19, 1992.
Schroeppel, R. and Gosper, R. W. Item 122 in Beeler, M.; Gosper, R. W.; and Schroeppel, R. HAKMEM. Cambridge, MA: MIT Artificial Intelligence Laboratory, Memo AIM-239, pp. 56-57, Feb. 1972. https://www.inwap.com/pdp10/hbaker/hakmem/series.html#item122.
Sloane, N. J. A. Sequences A010060, A014571, A014572, A074072, and A074073 in "The On-Line Encyclopedia of Integer Sequences."
 الاكثر قراءة في نظرية الاعداد
الاكثر قراءة في نظرية الاعداد
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة
قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)


















