

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Kaprekar Routine
المؤلف:
Eldridge, K. E. and Sagong, S.
المصدر:
"The Determination of Kaprekar Convergence and Loop Convergence of All 3-Digit Numbers." Amer. Math. Monthly 95
الجزء والصفحة:
...
14-11-2020
1077
Kaprekar Routine
The Kaprekar routine is an algorithm discovered in 1949 by D. R. Kaprekar for 4-digit numbers, but which can be generalized to 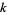 -digit numbers. To apply the Kaprekar routine to a number
-digit numbers. To apply the Kaprekar routine to a number 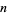 , arrange the digits in descending (
, arrange the digits in descending ( ) and ascending (
) and ascending (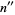 ) order. Now compute
) order. Now compute 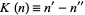 (discarding any initial 0s) and iterate, where
(discarding any initial 0s) and iterate, where  is sometimes called the Kaprekar function. The algorithm reaches 0 (a degenerate case), a constant, or a cycle, depending on the number of digits in
is sometimes called the Kaprekar function. The algorithm reaches 0 (a degenerate case), a constant, or a cycle, depending on the number of digits in 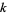 and the value of
and the value of 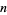 . The list of values is sometimes called a Kaprekar sequence, and the result
. The list of values is sometimes called a Kaprekar sequence, and the result 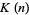 is sometimes called a Kaprekar number (Deutsch and Goldman 2004), though this nomenclature should be deprecated because of confusing with the distinct sort of Kaprekar number.
is sometimes called a Kaprekar number (Deutsch and Goldman 2004), though this nomenclature should be deprecated because of confusing with the distinct sort of Kaprekar number.
In base-10, the numbers  for which
for which 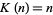 are given by 495, 6174, 549945, 631764, ... (OEIS A099009). Similarly, the numbers
are given by 495, 6174, 549945, 631764, ... (OEIS A099009). Similarly, the numbers  for which iterating
for which iterating 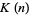 gives a cycle of length
gives a cycle of length 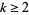 are given by 53955, 59994, 61974, 62964, 63954, 71973, ... (OEIS A099010).
are given by 53955, 59994, 61974, 62964, 63954, 71973, ... (OEIS A099010).
Iterating the Kaprekar map in base-10, all 1- and 2-digit numbers give 0. Exactly 60 3-digit numbers, namely 100, 101, 110, 111, 112, 121, 122, 211, 212, 221, ... (OEIS A090429), reach 0, while the rest give 495 in at most 6 iterations. Exactly 77 4-digit numbers, namely 1000, 1011, 1101, 1110, 1111, 1112, 1121, 1211, ... (OEIS A069746), reach 0, while the remainder give 6174 in at most 8 iterations. The value 6174 is sometimes known as Kaprekar's constant (Deutsch and Goldman 2004). This pattern breaks down for 5-digit numbers, which may converge to 0 or one of the 10 constants 53955, 59994, 61974, 62964, 63954, 71973, 74943, 75933, 82962, 83952.
The following table summarizes the possible cycles in various bases 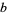 and the first few numbers of digits.
and the first few numbers of digits.
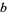 |
possible cycles for 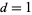 , 2, ... base- , 2, ... base-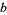 digits digits |
| 2 | 0, 0, 9, 21, |
| 3 | 0, 0, (32, 52), 184, (320, 580, 484), ... |
| 4 | 0, 30, |
| 5 | 8, (48, 72), 392, (1992, 2616, 2856, 2232), (7488, 10712, 9992, 13736, 11432), ... |
| 6 | 0, 105, (430, 890, 920, 675, 860, 705), |
| 7 | 0, (144, 192), (1068, 1752, 1836), (9936, 15072, 13680, 13008, 10608), (55500, 89112, 91800, 72012, 91212, 77388), ... |
| 8 | 21, 252, |
| 9 | (16, 48), (320, 400), |
| 10 | 0, 495, 6174, |

The figure above (similar to that appearing on the cover of the above issue of The Mathematics Teacher) shows the number of steps required for the Kaprekar routine to reach a fixed point for values of 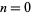 to 9999, partitioned into rows of length 100 (Deutsch and Goldman 2004). In this plot, numbers having fewer than 4 digits are padded with leading 0s, thus resulting in all values converging to 6174.
to 9999, partitioned into rows of length 100 (Deutsch and Goldman 2004). In this plot, numbers having fewer than 4 digits are padded with leading 0s, thus resulting in all values converging to 6174.
REFERENCES:
Deutsch, D. and Goldman, B. "Kaprekar's Constant." Math. Teacher 98, 234-242, 2004.
Eldridge, K. E. and Sagong, S. "The Determination of Kaprekar Convergence and Loop Convergence of All 3-Digit Numbers." Amer. Math. Monthly 95, 105-112, 1988.
Kaprekar, D. R. "An Interesting Property of the Number 6174." Scripta Math. 15, 244-245, 1955.
Sloane, N. J. A. Sequences A069746,A090429, A099009, and A099010 in "The On-Line Encyclopedia of Integer Sequences."
Trigg, C. W. "All Three-Digit Integers Lead to ...." The Math. Teacher, 67, 41-45, 1974.
Young, A. L. "A Variation on the 2-digit Kaprekar Routine." Fibonacci Quart. 31, 138-145, 1993.
 الاكثر قراءة في نظرية الاعداد
الاكثر قراءة في نظرية الاعداد
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام) قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)
قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)


















