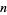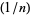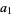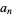A Diophantine problem (i.e., one whose solution must be given in terms of integers) which seeks a solution to the following problem. Given  men and a pile of coconuts, each man in sequence takes th of the coconuts left after the previous man removed his (i.e.,  for the first man, , for the second, ...,  for the last) and gives  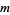coconuts (specified in the problem to be the same number for each man) which do not divide equally to a monkey. When all  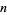men have so divided, they divide the remaining coconuts  ways (i.e., taking an additional  coconuts each), and give the  coconuts which are left over to the monkey. If 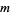 is the same at each division, then how many coconuts  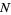were there originally? The solution is equivalent to solving the  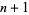Diophantine equations

			(1)
			(2)
			(3)
			(4)
			(5)

which can be rewritten as

			(6)
			(7)
			(8)
			(9)
			(10)
			(11)

Since there are 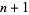 equations in the  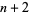unknowns 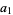, , 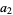..., 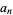, , 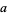and , the solutions span a one-dimensional space (i.e., there is an infinite family of solution parameterized by a single value). The solution to these equations can be given by

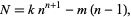(12)

where 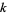 is an arbitrary integer (Gardner 1961).

For the particular case of 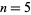 men and  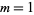left over coconuts, the 6 equations can be combined into the single Diophantine equation

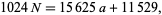(13)

where 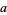 is the number given to each man in the last division. The smallest positive solution in this case is  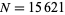coconuts, corresponding to  and 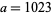; Gardner 1961). The following table shows how this rather large number of coconuts is divided under the scheme described above.

removed	given to monkey	left
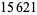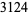	1
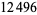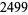	1
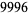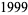	1
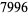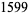	1
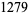	1
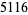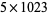	1	0

If no coconuts are left for the monkey after the final -way division (Williams 1926), then the original number of coconuts is

{(1+nk)n^n-(n-1) n odd; (n-1+nk)n^n-(n-1) n even. " src="https://mathworld.wolfram.com/images/equations/MonkeyandCoconutProblem/NumberedEquation3.gif" style="height:41px; width:205px" />

(14)

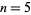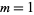The smallest positive solution for case  and  is 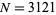 coconuts, corresponding to  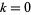and 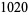 coconuts in the final division (Gardner 1961). The following table shows how these coconuts are divided.

removed	given to monkey	left
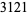624	1
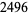499	1
399	1
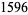319	1
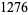255	1
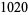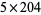	0	0

A different version of the problem having a solution of 79 coconuts is considered by Pappas (1989).

REFERENCES:

Anning, N. "Monkeys and Coconuts." Math. Teacher 54, 560-562, 1951.

Bowden, J. "The Problem of the Dishonest Men, the Monkeys, and the Coconuts." In Special Topics in Theoretical Arithmetic. Lancaster, PA: Lancaster Press, pp. 203-212, 1936.

Gardner, M. "The Monkey and the Coconuts." Ch. 9 in The Second Scientific American Book of Puzzles & Diversions: A New Selection. New York: Simon and Schuster, pp. 104-111, 1961.

Kirchner, R. B. "The Generalized Coconut Problem." Amer. Math. Monthly 67, 516-519, 1960.

Moritz, R. E. "Solution to Problem 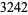." Amer. Math. Monthly 35, 47-48, 1928.

Ogilvy, C. S. and Anderson, J. T. Excursions in Number Theory. New York: Dover, pp. 52-54, 1988.

Olds, C. D. Continued Fractions. New York: Random House, pp. 48-50, 1963.

Pappas, T. "The Monkey and the Coconuts." The Joy of Mathematics. San Carlos, CA: Wide World Publ./Tetra, pp. 226-227 and 234, 1989.

Williams, B. A. "Coconuts." The Saturday Evening Post, Oct. 9, 1926.

اخر الاخبار

اخبار العتبة العباسية المقدسة

قسم الشؤون الفكرية يصدر العدد 55 من مجلة العميد المحكمة

المجمع العلمي يجري اختبار القبول للمتقدمين إلى دورة ربيع القرآن في كربلاء

وفد الهيأة العُليا لإحياء التراث يزور السيد موسى الزنجاني بمدينة قم المقدّسة

لتعزيز الشراكات المعرفية.. وفد قسم شؤون المعارف يلتقي عددًا من أعلام مدينة النجف الأشرف

المزيد

الآخبار الصحية

كنز غذائي على طبقك.. ماذا تفعل السبانخ بجسمك؟

ما لا يتوقعه أحد.. "سر غذائي" في الجبن الدسم

لتحسين الهضم والنوم.. هذا أفضل وقت لتناول العشاء

10 طرق بسيطة وغير مألوفة لحرق المزيد من السعرات يوميا

المزيد

الآخبار التكنلوجية

سابقة بعالم الطيران.. طائرة تقرر الهبوط بنفسها بعد ظرف طارئ

"الإنفلونزا الخارقة" تكتسح دول العالم.. وتثير قلقا كبيرا

مرحلة ما قبل الأعراض.. دواء جديد يستهدف "شرارة" الزهايمر

إزالة ثاني أكسيد الكربون من الغلاف الجوي.. ما دور المحيطات والبحار؟

المزيد