Circular Segment
المؤلف:
Beyer, W. H.
المصدر:
CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press
الجزء والصفحة:
...
10-4-2020
1490
Circular Segment
A portion of a disk whose upper boundary is a (circular) arc and whose lower boundary is a chord making a central angle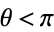 
radians (
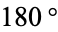), illustrated above as the shaded region. The entire wedge-shaped area is known as a circular sector.
Circular segments are implemented in the Wolfram Language as DiskSegment[![<span style=]()
{" src="https://mathworld.wolfram.com/images/equations/CircularSegment/Inline3.gif" style="height:15px; width:5px" />x, y![<span style=]()
}" src="https://mathworld.wolfram.com/images/equations/CircularSegment/Inline4.gif" style="height:15px; width:5px" />, r, ![<span style=]()
{" src="https://mathworld.wolfram.com/images/equations/CircularSegment/Inline5.gif" style="height:15px; width:5px" />q1, q2![<span style=]()
}" src="https://mathworld.wolfram.com/images/equations/CircularSegment/Inline6.gif" style="height:15px; width:5px" />]. Elliptical segments are similarly implemented as DiskSegment[![<span style=]()
{" src="https://mathworld.wolfram.com/images/equations/CircularSegment/Inline7.gif" style="height:15px; width:5px" />x, y![<span style=]()
}" src="https://mathworld.wolfram.com/images/equations/CircularSegment/Inline8.gif" style="height:15px; width:5px" />, ![<span style=]()
{" src="https://mathworld.wolfram.com/images/equations/CircularSegment/Inline9.gif" style="height:15px; width:5px" />r1, r2![<span style=]()
}" src="https://mathworld.wolfram.com/images/equations/CircularSegment/Inline10.gif" style="height:15px; width:5px" />, ![<span style=]()
{" src="https://mathworld.wolfram.com/images/equations/CircularSegment/Inline11.gif" style="height:15px; width:5px" />q1, q2![<span style=]()
}" src="https://mathworld.wolfram.com/images/equations/CircularSegment/Inline12.gif" style="height:15px; width:5px" />].
Let 
be the radius of the circle,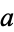 
the chord length, 
the arc length,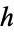 
the height of the arced portion, and 
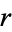the height of the triangular portion. Then the radius is
 |
(1)
|
the arc length is
 |
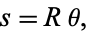(2)
|
the height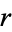 
is
and the length of the chord is
From elementary trigonometry, the angle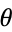 
obeys the relationships
The area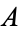 
of the (shaded) segment is then simply given by the area of the circular sector (the entire wedge-shaped portion) minus the area of the bottom triangular portion,
 |
(14)
|
Plugging in gives
where the formula for the isosceles triangle in terms of the polygon vertex angle has been used (Beyer 1987). These formula find application in the common case of determining the volume of fluid in a cylindrical segment (i.e., horizontal cylindrical tank) based on the height of the fluid in the tank.
The area can also be found directly by integration as
 |
(19)
|
It follows that the weighted mean of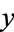 
is
so the geometric centroid of the circular segment is
 |
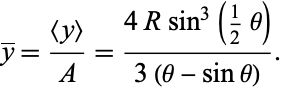(22)
|
Checking shows that this obeys the proper limits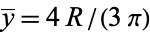 
for a semicircle (
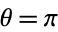) and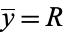 
for a point mass at the top of the segment (
).
Finding the value of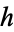 
such that the circular segment (left figure) has area equal to 1/4 of the circle (right figure) is sometimes known as the quarter-tank problem.
Approximate formulas for the arc length and area are
 |
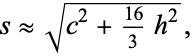(23)
|
accurate to within 0.3% for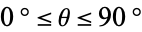 
, and
 |
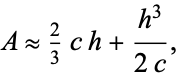(24)
|
accurate to within 0.1% for 
and 0.8% for 
(Harris and Stocker 1998).
REFERENCES:
Beyer, W. H. (Ed.). CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, p. 125, 1987.
Fukagawa, H. and Pedoe, D. "Segments of a Circle." §1.6 in Japanese Temple Geometry Problems. Winnipeg, Manitoba, Canada: Charles Babbage Research Foundation, pp. 14-15 and 88-92, 1989.
Harris, J. W. and Stocker, H. "Segment of a Circle." §3.8.6 in Handbook of Mathematics and Computational Science. New York: Springer-Verlag, pp. 92-93, 1998.
Kern, W. F. and Bland, J. R. Solid Mensuration with Proofs, 2nd ed. New York: Wiley, p. 4, 1948.
Sloane, N. J. A. Sequence A133742 in "The On-Line Encyclopedia of Integer Sequences."
الاكثر قراءة في نظرية الاعداد
اخر الاخبار
اخبار العتبة العباسية المقدسة
