

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Reverse Polish Notation
المؤلف:
Stone, A
المصدر:
"Reverse Polish Notation." http://www-stone.ch.cam.ac.uk/documentation/rrf/rpn.html.
الجزء والصفحة:
...
12-11-2019
1141
Reverse Polish Notation
Reverse Polish notation (RPN) is a method for representing expressions in which the operator symbol is placed after the arguments being operated on. Polish notation, in which the operator comes before the operands, was invented in the 1920s by the Polish mathematician Jan Lucasiewicz. In the late 1950s, Australian philosopher and computer scientist Charles L. Hamblin suggested placing the operator after the operands and hence created reverse polish notation.
For example, the following RPN expression will produce the sum of 2 and 3, namely 5: 2 3 +.
Reverse Polish notation, also known as postfix notation, contrasts with the "infix notation" of standard arithmetic expressions in which the operator symbol appears between the operands.
RPN has the property that brackets are not required to represent the order of evaluation or grouping of the terms. RPN expressions are simply evaluated from left to right and this greatly simplifies the computation of the expression within computer programs. As an example, the arithmetic expression 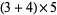 can be expressed in RPN as
can be expressed in RPN as 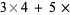 .
.
In practice RPN can be conveniently evaluated using a stack structure. Reading the expression from left to right, the following operations are performed:
1. If a value appears next in the expression, push this value on to the stack.
2. If an operator appears next, pop two items from the top of the stack and push the result of the operation on to the stack.
A standard infix arithmetic expression can be converted to an RPN expression using a parsing algorithm as a recursive descent parse.
RPN is used in Hewlett Packard and some Texas Instruments calculators and internally in some computer languages.
REFERENCES:
The Calculator Home Page. "Reverse Polish Notation." http://www.calculator.org/rpn.html.
Stone, A. "Reverse Polish Notation." http://www-stone.ch.cam.ac.uk/documentation/rrf/rpn.html.
 الاكثر قراءة في نظرية الاعداد
الاكثر قراءة في نظرية الاعداد
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة
قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)


















