

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Rogers L-Function
المؤلف:
Abel, N. H.
المصدر:
Oeuvres Completes, Vol. 2 (Ed. L. Sylow and S. Lie). New York: Johnson Reprint Corp.,
الجزء والصفحة:
...
13-8-2019
2556
Rogers L-Function

If 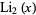 denotes the usual dilogarithm, then there are two variants that are normalized slightly differently, both called the Rogers
denotes the usual dilogarithm, then there are two variants that are normalized slightly differently, both called the Rogers 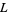 -function (Rogers 1907). Bytsko (1999) defines
-function (Rogers 1907). Bytsko (1999) defines
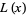 |
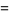 |
![6/(pi^2)[Li_2(x)+1/2lnxln(1-x)]](http://mathworld.wolfram.com/images/equations/RogersL-Function/Inline5.gif) |
(1) |
 |
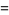 |
![6/(pi^2)[sum_(n=1)^(infty)(x^n)/(n^2)+1/2lnxln(1-x)],](http://mathworld.wolfram.com/images/equations/RogersL-Function/Inline8.gif) |
(2) |
(which he calls "the" dilogarithm), while Gordon and McIntosh (1997) and Loxton (1991, p. 287) define the Rogers 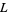 -function as
-function as
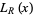 |
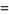 |
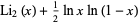 |
(3) |
 |
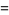 |
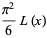 |
(4) |
 |
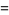 |
![[sum_(n=1)^(infty)(x^n)/(n^2)+1/2lnxln(1-x)].](http://mathworld.wolfram.com/images/equations/RogersL-Function/Inline18.gif) |
(5) |
The function 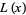 satisfies the concise reflection relation
satisfies the concise reflection relation
 |
(6) |
(Euler 1768), as well as Abel's functional equation
 |
(7) |
(Abel 1988, Bytsko 1999). Abel's duplication formula for  follows from Abel's functional equation and is given by
follows from Abel's functional equation and is given by
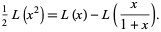 |
(8) |
The function has the nice series
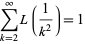 |
(9) |
(Lewin 1982; Loxton 1991, p. 298).
In terms of 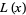 , the well-known dilogarithm identities become
, the well-known dilogarithm identities become
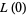 |
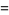 |
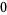 |
(10) |
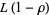 |
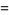 |
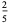 |
(11) |
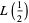 |
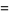 |
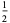 |
(12) |
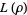 |
 |
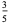 |
(13) |
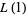 |
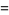 |
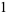 |
(14) |
(Loxton 1991, pp. 287 and 289; Bytsko 1999), where 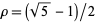 .
.
Numbers 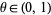 which satisfy
which satisfy
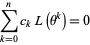 |
(15) |
for some value of 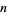 are called L-algebraic numbers. Loxton (1991, p. 289) gives a slew of identities having rational coefficients
are called L-algebraic numbers. Loxton (1991, p. 289) gives a slew of identities having rational coefficients
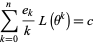 |
(16) |
instead of integers, where 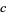 is a rational number, a corrected and expanded version of which is summarized in the following table. In this table, polynomials
is a rational number, a corrected and expanded version of which is summarized in the following table. In this table, polynomials 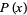 denote the real root of
denote the real root of  . Many more similar identities can be found using integer relationalgorithms.
. Many more similar identities can be found using integer relationalgorithms.
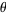 |
 |
 |
| 1 | 1 | 1 |
 |
1 |  |
 |
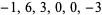 |
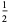 |
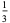 |
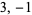 |
1 |
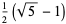 |
1 | 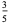 |
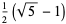 |
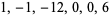 |
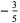 |
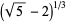 |
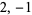 |
1 |
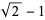 |
 |
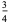 |
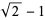 |
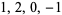 |
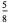 |
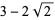 |
 |
1 |
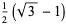 |
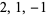 |
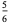 |
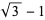 |
 |
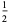 |
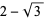 |
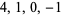 |
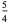 |
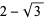 |
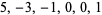 |
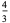 |
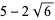 |
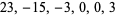 |
3 |
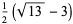 |
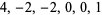 |
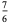 |
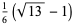 |
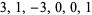 |
 |
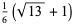 |
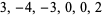 |
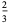 |
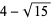 |
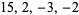 |
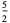 |
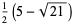 |
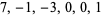 |
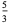 |
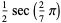 , , |
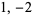 |
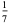 |
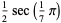 |
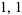 |
 |
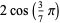 |
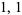 |
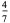 |
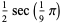 |
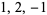 |
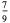 |
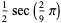 |
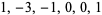 |
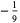 |
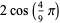 |
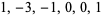 |
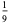 |
 |
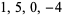 |
1 |
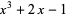 |
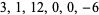 |
2 |
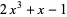 |
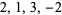 |
 |
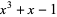 |
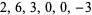 |
3 |
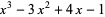 |
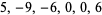 |
1 |
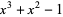 |
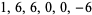 |
2 |
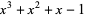 |
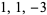 |
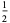 |
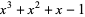 |
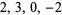 |
 |
Bytsko (1999) gives the additional identities
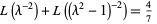 |
(17) |
 |
(18) |
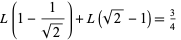 |
(19) |
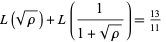 |
(20) |
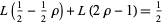 |
(21) |
 |
(22) |
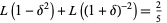 |
(23) |
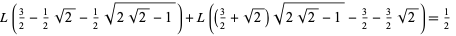 |
(24) |
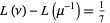 |
(25) |
where
 |
 |
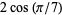 |
(26) |
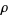 |
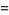 |
 |
(27) |
 |
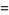 |
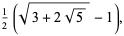 |
(28) |
with  the positive root of
the positive root of
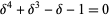 |
(29) |
and 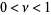 and
and 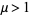 the real roots of
the real roots of
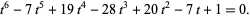 |
(30) |
Here, (◇) and (◇) are special cases of Watson's identities and (◇) is a special case of Abel's duplication formula with 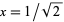 (Gordon and McIntosh 1997, Bytsko 1999).
(Gordon and McIntosh 1997, Bytsko 1999).
Rogers (1907) obtained a dilogarithm identity in 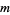 variables with
variables with 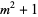 terms which simplifies to Euler's identity for
terms which simplifies to Euler's identity for 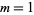 and Abel's functional equation for
and Abel's functional equation for 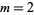 (Gordon and McIntosh 1997). For
(Gordon and McIntosh 1997). For 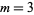 , it is equivalent to
, it is equivalent to
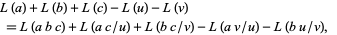 |
(31) |
with
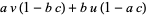 |
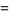 |
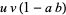 |
(32) |
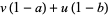 |
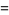 |
 |
(33) |
(Gordon and McIntosh 1997).
REFERENCES:
Abel, N. H. Oeuvres Completes, Vol. 2 (Ed. L. Sylow and S. Lie). New York: Johnson Reprint Corp., pp. 189-192, 1988.
Bytsko, A. G. "Fermionic Representations for Characters of 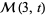 ,
,  ,
, 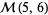 and
and 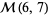 Minimal Models and Related Dilogarithm and Rogers-Ramanujan-Type Identities." J. Phys. A: Math. Gen. 32, 8045-8058, 1999.
Minimal Models and Related Dilogarithm and Rogers-Ramanujan-Type Identities." J. Phys. A: Math. Gen. 32, 8045-8058, 1999.
Bytsko, A. G. "Two-Term Dilogarithm Identities Related to Conformal Field Theory." 9 Nov 1999. http://arxiv.org/abs/math-ph/9911012.
Euler, L. Institutiones calculi integralis, Vol. 1. Basel, Switzerland: Birkhäuser, pp. 110-113, 1768.
Gordon, B. and McIntosh, R. J. "Algebraic Dilogarithm Identities." Ramanujan J. 1, 431-448, 1997.
Lewin, L. "The Dilogarithm in Algebraic Fields." J. Austral. Math. Soc. (Ser. A) 33, 302-330, 1982.
Lewin, L. (Ed.). Structural Properties of Polylogarithms. Providence, RI: Amer. Math. Soc., 1991.
Loxton, J. H. "Partition Identities and the Dilogarithm." Ch. 13 in Structural Properties of Polylogarithms (Ed. L. Lewin). Providence, RI: Amer. Math. Soc., pp. 287-299, 1991.
Rogers, L. J. "On Function Sum Theorems Connected with the Series 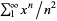 ." Proc. London Math. Soc. 4, 169-189, 1907.
." Proc. London Math. Soc. 4, 169-189, 1907.
Watson, G. N. "A Note on Spence's Logarithmic Transcendent." Quart. J. Math. Oxford Ser. 8, 39-42, 1937.
 الاكثر قراءة في التفاضل و التكامل
الاكثر قراءة في التفاضل و التكامل
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة
قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)


















