Dawson's Integral
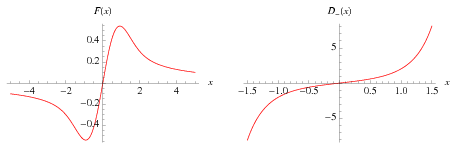
Dawson's integral (Abramowitz and Stegun 1972, pp. 295 and 319), also sometimes called Dawson's function, is the entire function given by the integral
where 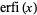 is erfi, that arises in computation of the Voigt lineshape (Harris 1948, Hummer 1963, Sajo 1993, Lether 1997), in heat conduction, and in the theory of electrical oscillations in certain special vacuum tubes (McCabe 1974). It is commonly denoted
is erfi, that arises in computation of the Voigt lineshape (Harris 1948, Hummer 1963, Sajo 1993, Lether 1997), in heat conduction, and in the theory of electrical oscillations in certain special vacuum tubes (McCabe 1974). It is commonly denoted 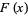 (McCabe 1974; Coleman 1987; Milone and Milone 1988; Sajo 1993; Lether 1997; Press et al. 2007, p. 302), although Spanier and Oldham (1987) denote it by
(McCabe 1974; Coleman 1987; Milone and Milone 1988; Sajo 1993; Lether 1997; Press et al. 2007, p. 302), although Spanier and Oldham (1987) denote it by 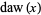 .
.
Dawson's integral is implemented in the Wolfram Language as DawsonF[z].
It is an odd function, so
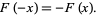 |
(3)
|
Its derivative is
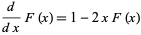 |
(4)
|
and its indefinite integral is
 |
(5)
|
where 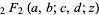 is a generalized hypergeometric function.
is a generalized hypergeometric function.
It is the particular solution to the differential equation
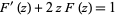 |
(6)
|
(McCabe 1974).
Its Maclaurin series is given by
(OEIS A122803 and A001147). If has the asymptotic series
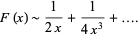 |
(9)
|
It also arises in the semi-integral of 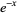 via
via
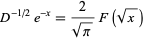 |
(10)
|
(Spanier and Oldham 1987, p. 406).
It is given by the sums
(Spanier and Oldham 1987, p. 407), where 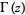 is the gamma function and
is the gamma function and 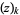 is a Pochhammer symbol.
is a Pochhammer symbol.
Dawson's integral has continued fractions
(McCabe 1974).

The plots above show the behavior of 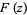 in the complex plane.
in the complex plane.
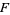 has a maximum at
has a maximum at 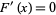 , or
, or
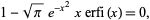 |
(15)
|
giving
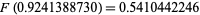 |
(16)
|
(OEIS A133841 and A133842), and an inflection at 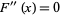 , or
, or
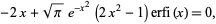 |
(17)
|
giving
 |
(18)
|
(OEIS A133843).
The function is sometimes generalized such that
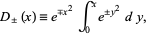 |
(19)
|
giving
where  is the erf function and
is the erf function and 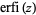 is the imaginary error function erfi.
is the imaginary error function erfi.
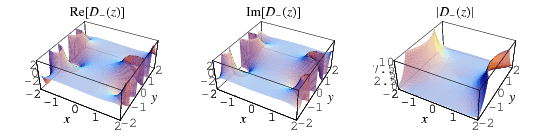
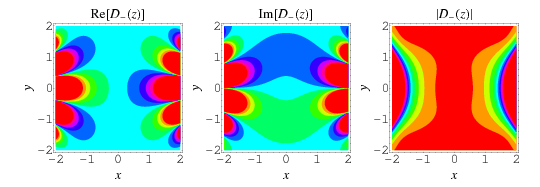
The plots above show the behavior of 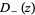 in the complex plane.
in the complex plane.
REFERENCES:
Abramowitz, M. and Stegun, I. A. (Eds.). Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 295 and 319, 1972.
Cody, W. J.; Pociorek, K. A.; and Thatcher, H. C. "Chebyshev Approximations for Dawson's Integral." Math. Comput. 24, 171-178, 1970.
Coleman, J. P. "Complex Polynomial Approximation by the Lanczos  -Method: Dawson's Integral." J. Comput. Appl. Math. 20, 137-151, 1987.
-Method: Dawson's Integral." J. Comput. Appl. Math. 20, 137-151, 1987.
Dawson, F. "On the Numerical Value of 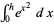 ." London Math. Soc. Proc. 29, 519-522, 1898.
." London Math. Soc. Proc. 29, 519-522, 1898.
Dijkstra, D. A. "A Continued Fraction Expansion for a Generalization of Dawson's Integral." Math. Comp. 31, 503-510, 1977.
Faddeyeva, V. N. and Terent'ev, N. M. Tables of Values of the Function  for Complex Argument. New York: Pergamon Press, 1961.
for Complex Argument. New York: Pergamon Press, 1961.
Harris, D. III. "On the Line Absorption Coefficients Due to Doppler Effect and Damping." Astrophys. J. 108, 1120-115, 1948.
Hummer, D. G. "Noncoherent Scattering I. The Redistribution Functions with Doppler Broadening." Monthly Not. Roy. Astron. Soc. 125, 21-37, 1963.
Hummer, D. G. "Expansion of Dawson's Function in a Series of Chebyshev Polynomials." Math. Comput. 18, 317-319, 1964.
Lether, F. G. "Elementary Approximations for Dawson's Integral." J. Quant. Spectros. Radiat. Transfer 4, 343-345, 1991.
Lether, F. G. "Constrained Near-Minimax Rational Approximations to Dawson's Integral." Appl. Math. Comput. 88, 267-274, 1997.
Lohmander, B. and Rittsten, S. "Table of the Function 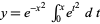 ." Kungl. Fysiogr. Sällsk. i Lund Föhr. 28, 45-52, 1958.
." Kungl. Fysiogr. Sällsk. i Lund Föhr. 28, 45-52, 1958.
Luke, Y. L. The Special Functions and their Approximations, Vol. 2. New York: Academic Press, 1969.
McCabe, J. H. "A Continued Fraction Expansion with a Truncation Error Estimate for Dawson's Integral." Math. Comput. 28, 811-816, 1974.
Milone, L. A. and Milone, A. A. E. "Evaluation of Dawson's Function." Astrophys. Space Sci. 147, 189-191, 1988.
Moshier, S. L. Methods and Programs for Mathematical Functions. Chichester, England: Ellis Horwood, 1989.
Press, W. H.; Flannery, B. P.; Teukolsky, S. A.; and Vetterling, W. T. "Dawson's Integral." §6.10 in Numerical Recipes: The Art of Scientific Computing, 3rd ed. Cambridge, England: Cambridge University Press, pp. 302-304, 2007.
Rosser, J. B. "Theory and Application of 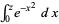 and
and  ." Brooklyn, NY: Mapleton House, 1948.
." Brooklyn, NY: Mapleton House, 1948.
Rybicki, G. B. "Dawson's Integral and the Sampling Theorem." Computers in Physics 3, 85-87, 1989.
Sajo, E. "On the Recursive Properties of Dawson's Integral." J. Phys. A 26, 2977-2987, 1993.
Sloane, N. J. A. Sequences A001147/M3002, A122803, A133841, A133842, and A133843 in "The On-Line Encyclopedia of Integer Sequences."
Spanier, J. and Oldham, K. B. "Dawson's Integral." Ch. 42 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 405-410, 1987.
 الاكثر قراءة في التفاضل و التكامل
الاكثر قراءة في التفاضل و التكامل
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة


