
Hyperbolic Functions
 المؤلف:
Abramowitz, M. and Stegun, I. A.
المؤلف:
Abramowitz, M. and Stegun, I. A.
 المصدر:
"Hyperbolic Functions." §4.5 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover
المصدر:
"Hyperbolic Functions." §4.5 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover
 الجزء والصفحة:
...
الجزء والصفحة:
...
 3-6-2019
3-6-2019
 4222
4222
Hyperbolic Functions
The hyperbolic functions 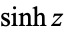 ,
, 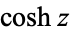 ,
, 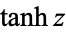 ,
, 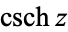 ,
, 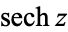 ,
, 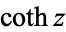 (hyperbolic sine, hyperbolic cosine, hyperbolic tangent, hyperbolic cosecant, hyperbolic secant, and hyperbolic cotangent) are analogs of the circular functions, defined by removing
(hyperbolic sine, hyperbolic cosine, hyperbolic tangent, hyperbolic cosecant, hyperbolic secant, and hyperbolic cotangent) are analogs of the circular functions, defined by removing 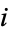 s appearing in the complex exponentials. For example,
s appearing in the complex exponentials. For example,
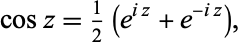 |
(1)
|
so
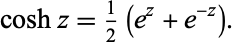 |
(2)
|
Note that alternate notations are sometimes used, as summarized in the following table.
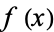 |
alternate notations |
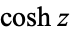 |
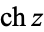 (Gradshteyn and Ryzhik 2000, p. xxvii) (Gradshteyn and Ryzhik 2000, p. xxvii) |
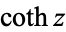 |
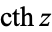 (Gradshteyn and Ryzhik 2000, p. xxvii) (Gradshteyn and Ryzhik 2000, p. xxvii) |
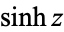 |
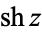 (Gradshteyn and Ryzhik 2000, p. xxvii) (Gradshteyn and Ryzhik 2000, p. xxvii) |
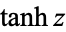 |
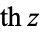 (Gradshteyn and Ryzhik 2000, p. xxvii) (Gradshteyn and Ryzhik 2000, p. xxvii) |
The hyperbolic functions share many properties with the corresponding circular functions. In fact, just as the circle can be represented parametrically by
a rectangular hyperbola (or, more specifically, its right branch) can be analogously represented by
where 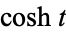 is the hyperbolic cosine and
is the hyperbolic cosine and  is the hyperbolic sine.
is the hyperbolic sine.
The hyperbolic functions arise in many problems of mathematics and mathematical physics in which integrals involving 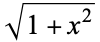 arise (whereas the circular functions involve
arise (whereas the circular functions involve 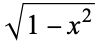 ). For instance, the hyperbolic sine arises in the gravitational potential of a cylinder and the calculation of the Roche limit. The hyperbolic cosine function is the shape of a hanging cable (the so-called catenary). The hyperbolic tangent arises in the calculation of and rapidity of special relativity. All three appear in the Schwarzschild metric using external isotropic Kruskal coordinates in general relativity. The hyperbolic secant arises in the profile of a laminar jet. The hyperbolic cotangent arises in the Langevin function for magnetic polarization.
). For instance, the hyperbolic sine arises in the gravitational potential of a cylinder and the calculation of the Roche limit. The hyperbolic cosine function is the shape of a hanging cable (the so-called catenary). The hyperbolic tangent arises in the calculation of and rapidity of special relativity. All three appear in the Schwarzschild metric using external isotropic Kruskal coordinates in general relativity. The hyperbolic secant arises in the profile of a laminar jet. The hyperbolic cotangent arises in the Langevin function for magnetic polarization.
The hyperbolic functions are defined by
For arguments multiplied by 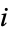 ,
,
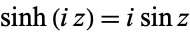 |
(17)
|
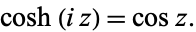 |
(18)
|
The hyperbolic functions satisfy many identities analogous to the trigonometric identities (which can be inferred using Osborn's rule) such as
See also Beyer (1987, p. 168).
Some half-angle formulas are
where 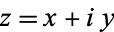 .
.
Some double-angle formulas are
Identities for complex arguments include
The absolute squares for complex arguments are
REFERENCES:
Abramowitz, M. and Stegun, I. A. (Eds.). "Hyperbolic Functions." §4.5 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 83-86, 1972.
Anderson, J. W. "Trigonometry in the Hyperbolic Plane." §5.7 in Hyperbolic Geometry. New York: Springer-Verlag, pp. 146-151, 1999.
Beyer, W. H. "Hyperbolic Function." CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, pp. 168-186 and 219, 1987.
Coxeter, H. S. M. and Greitzer, S. L. Geometry Revisited. Washington, DC: Math. Assoc. Amer., pp. 126-131, 1967.
Harris, J. W. and Stocker, H. "Hyperbolic Functions." Handbook of Mathematics and Computational Science. New York: Springer-Verlag, pp. 245-262, 1998.
Jeffrey, A. "Hyperbolic Identities." §2.5 in Handbook of Mathematical Formulas and Integrals, 2nd ed. Orlando, FL: Academic Press, pp. 117-122, 2000.
Yates, R. C. "Hyperbolic Functions." A Handbook on Curves and Their Properties. Ann Arbor, MI: J. W. Edwards, pp. 113-118, 1952.
Zwillinger, D. (Ed.). "Hyperbolic Functions." §6.7 in CRC Standard Mathematical Tables and Formulae. Boca Raton, FL: CRC Press, pp. 476-481 1995.
 الاكثر قراءة في التفاضل و التكامل
الاكثر قراءة في التفاضل و التكامل
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة


