Double Factorial
المؤلف:
Arfken, G
المصدر:
Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press
الجزء والصفحة:
...
15-5-2019
4650
Double Factorial
The double factorial of a positive integer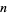 
is a generalization of the usual factorial 
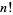defined by
![n!!=<span style=]() {n·(n-2)...5·3·1 n>0 odd; n·(n-2)...6·4·2 n>0 even; 1 n=-1,0. " src="http://mathworld.wolfram.com/images/equations/DoubleFactorial/NumberedEquation1.gif" style="height:62px; width:225px" /> |
(1)
|
Note that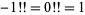 
, by definition (Arfken 1985, p. 547).
The origin of the notation 
appears not to not be widely known and is not mentioned in Cajori (1993).
For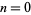 
, 1, 2, ..., the first few values are 1, 1, 2, 3, 8, 15, 48, 105, 384, ... (OEIS A006882). The numbers of decimal digits in 
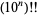for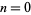 
, 1, ... are 1, 4, 80, 1285, 17831, 228289, 2782857, 32828532, ... (OEIS A114488).
The double factorial is implemented in the Wolfram Language as n!! or Factorial2[n].
The double factorial is a special case of the multifactorial.
The double factorial can be expressed in terms of the gamma function by
 |
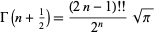(2)
|
(Arfken 1985, p. 548).
The double factorial can also be extended to negative odd integers using the definition
for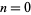 
, 1, ... (Arfken 1985, p. 547).
Similarly, the double factorial can be extended to complex arguments as
![z!!=2^([1+2z-cos(piz)]/4)pi^([cos(piz)-1]/4)Gamma(1+1/2z).](http://mathworld.wolfram.com/images/equations/DoubleFactorial/NumberedEquation3.gif) |
(5)
|
There are many identities relating double factorials to factorials. Since
![(2n+1)!!2^nn!
=[(2n+1)(2n-1)...1][2n][2(n-1)][2(n-2)]...2·1
=[(2n+1)(2n-1)...1][2n(2n-2)(2n-4)...2]
=(2n+1)(2n)(2n-1)(2n-2)(2n-3)(2n-4)...2·1
=(2n+1)!,](http://mathworld.wolfram.com/images/equations/DoubleFactorial/NumberedEquation4.gif) |
(6)
|
it follows that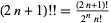 
. For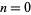 
, 1, ..., the first few values are 1, 3, 15, 105, 945, 10395, ... (OEIS A001147).
Also, since
it follows that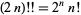 
. For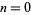 
, 1, ..., the first few values are 1, 2, 8, 48, 384, 3840, 46080, ... (OEIS A000165).
Finally, since
![(2n-1)!!2^nn!
=[(2n-1)(2n-3)...1][2n][2(n-1)][2(n-2)]...2(1)
=(2n-1)(2n-3)...1][2n(2n-2)(2n-4)...2]
=2n(2n-1)(2n-2)(2n-3)(2n-4)...2(1)
=(2n)!,](http://mathworld.wolfram.com/images/equations/DoubleFactorial/NumberedEquation5.gif) |
(10)
|
it follows that
 |
(11)
|
For 
odd,
For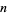 
even,
Therefore, for any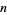 
,
 |
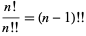(18)
|
 |
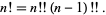(19)
|
The double factorial satisfies the beautiful series
The latter gives rhe sum of reciprocal double factorials in closed form as
(OEIS A143280), where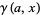 
is a lower incomplete gamma function. This sum is a special case of the reciprocal multifactorial constant.
A closed-form sum due to Ramanujan is given by
![sum_(n=0)^infty(-1)^n[((2n-1)!!)/((2n)!!)]^3=[(Gamma(9/8))/(Gamma(5/4)Gamma(7/8))]^2](http://mathworld.wolfram.com/images/equations/DoubleFactorial/NumberedEquation9.gif) |
(26)
|
(Hardy 1999, p. 106). Whipple (1926) gives a generalization of this sum (Hardy 1999, pp. 111-112).
REFERENCES:
Arfken, G. Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 544-545 and 547-548, 1985.
Cajori, F. A History of Mathematical Notations, Vol. 2. New York: Dover, 1993.
Hardy, G. H. Ramanujan: Twelve Lectures on Subjects Suggested by His Life and Work, 3rd ed. New York: Chelsea, 1999.
Meserve, B. E. "Double Factorials." Amer. Math. Monthly 55, 425-426, 1948.
Sloane, N. J. A. Sequences A000165/M1878, A001147/M3002, A006882/M0876, A114488, and A143280 in "The On-Line Encyclopedia of Integer Sequences."
Whipple, F. J. W. "On Well-Poised Series, Generalised Hypergeometric Series Having Parameters in Pairs, Each Pair with the Same Sum." Proc. London Math. Soc. 24, 247-263, 1926.
الاكثر قراءة في التفاضل و التكامل
اخر الاخبار
اخبار العتبة العباسية المقدسة
