Exponential Function
المؤلف:
Abramowitz, M. and Stegun, I. A.
المصدر:
"Exponential Function." §4.2 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover
الجزء والصفحة:
...
2-5-2019
3012
Exponential Function
The exponential function is the entire function defined by
 |
(1)
|
where e is the solution of the equation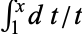 
so that 
. 
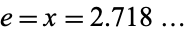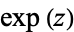is also the unique solution of the equation 
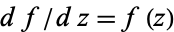with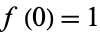 
.
The exponential function is implemented in the Wolfram Language as Exp[z].
It satisfies the identity
 |
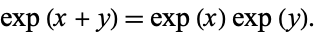(2)
|
If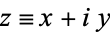 
,
 |
(3)
|
The exponential function satisfies the identities
where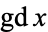 
is the Gudermannian (Beyer 1987, p. 164; Zwillinger 1995, p. 485).
The exponential function has Maclaurin series
 |
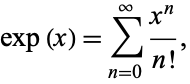(8)
|
and satisfies the limit
 |
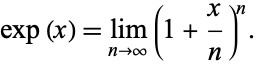(9)
|
If
 |
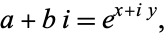(10)
|
then
The exponential function has continued fraction
 |
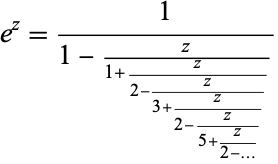(14)
|
(Wall 1948, p. 348).
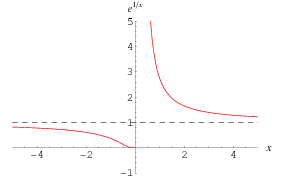
The above plot shows the function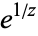 
(Trott 2004, pp. 165-166).
Integrals involving the exponential function include
(Borwein et al. 2004, p. 55).
REFERENCES:
Abramowitz, M. and Stegun, I. A. (Eds.). "Exponential Function." §4.2 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 69-71, 1972.
Beyer, W. H. CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, p. 217, 1987.
Borwein, J.; Bailey, D.; and Girgensohn, R. Experimentation in Mathematics: Computational Paths to Discovery. Wellesley, MA: A K Peters, 2004.
Finch, S. "Linear Independence of Exponential Functions." http://algo.inria.fr/csolve/sstein.html.
Fischer, G. (Ed.). Plates 127-128 in Mathematische Modelle aus den Sammlungen von Universitäten und Museen, Bildband.Braunschweig, Germany: Vieweg, pp. 124-125, 1986.
Krantz, S. G. "The Exponential and Applications." §1.2 in Handbook of Complex Variables. Boston, MA: Birkhäuser, pp. 7-12, 1999.
Spanier, J. and Oldham, K. B. "The Exponential Function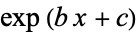 
" and "Exponentials of Powers 
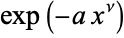." Chs. 26-27 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 233-261, 1987.
Trott, M. "Elementary Transcendental Functions." §2.2.3 in The Mathematica GuideBook for Programming. New York: Springer-Verlag, 2004. http://www.mathematicaguidebooks.org/.
Wall, H. S. Analytic Theory of Continued Fractions. New York: Chelsea, 1948.
Yates, R. C. "Exponential Curves." A Handbook on Curves and Their Properties. Ann Arbor, MI: J. W. Edwards, pp. 86-97, 1952.
Zwillinger, D. (Ed.). CRC Standard Mathematical Tables and Formulae. Boca Raton, FL: CRC Press, 1995.
الاكثر قراءة في التفاضل و التكامل
اخر الاخبار
اخبار العتبة العباسية المقدسة
