

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Klein,s Absolute Invariant
المؤلف:
Cohn, H
المصدر:
Introduction to the Construction of Class Fields. New York: Dover
الجزء والصفحة:
...
23-4-2019
2796
Klein's Absolute Invariant
 |
 |
Let  and
and  be periods of a doubly periodic function, with
be periods of a doubly periodic function, with 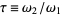 the half-period ratio a number with
the half-period ratio a number with ![I[tau]!=0](http://mathworld.wolfram.com/images/equations/KleinsAbsoluteInvariant/Inline4.gif) . Then Klein's absolute invariant (also called Klein's modular function) is defined as
. Then Klein's absolute invariant (also called Klein's modular function) is defined as
 |
(1) |
where  and
and 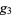 are the invariants of the Weierstrass elliptic function with modular discriminant
are the invariants of the Weierstrass elliptic function with modular discriminant
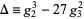 |
(2) |
(Klein 1877). If 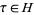 , where
, where 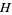 is the upper half-plane, then
is the upper half-plane, then
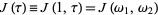 |
(3) |
is a function of the ratio  only, as are
only, as are  ,
, 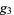 , and
, and 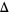 . Furthermore,
. Furthermore,  ,
,  ,
, 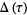 , and
, and 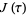 are analytic in
are analytic in 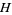 (Apostol 1997, p. 15).
(Apostol 1997, p. 15).
Klein's absolute invariant is implemented in the Wolfram Language as KleinInvariantJ[tau].
The function 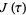 is the same as the j-function, modulo a constant multiplicative factor.
is the same as the j-function, modulo a constant multiplicative factor.
Every rational function of 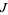 is a modular function, and every modular function can be expressed as a rational functionof
is a modular function, and every modular function can be expressed as a rational functionof 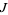 (Apostol 1997, p. 40).
(Apostol 1997, p. 40).
Klein's invariant can be given explicitly by
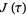 |
 |
![4/(27)([1-lambda(tau)+lambda^2(tau)]^3)/(lambda^2(tau)[1-lambda(tau)]^2)](http://mathworld.wolfram.com/images/equations/KleinsAbsoluteInvariant/Inline23.gif) |
(4) |
 |
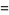 |
![([E_4(tau)]^3)/([E_4(tau)]^3-[E_6(tau)^2])](http://mathworld.wolfram.com/images/equations/KleinsAbsoluteInvariant/Inline26.gif) |
(5) |
(Klein 1878-1879, Cohn 1994), where 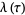 is the elliptic lambda function
is the elliptic lambda function
![lambda(tau)=[(theta_2(0,q))/(theta_3(0,q))]^4,](http://mathworld.wolfram.com/images/equations/KleinsAbsoluteInvariant/NumberedEquation4.gif) |
(6) |
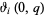 is a Jacobi theta function, the
is a Jacobi theta function, the 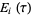 are Eisenstein series, and
are Eisenstein series, and 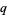 is the nome. Klein's invariant can also be simply expressed in terms of the five Weber functions
is the nome. Klein's invariant can also be simply expressed in terms of the five Weber functions 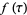 ,
, 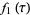 ,
, 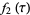 ,
, 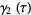 , and
, and  .
.
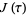 is invariant under a unimodular transformation, so
is invariant under a unimodular transformation, so
 |
(7) |
and 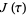 is a modular function.
is a modular function. 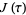 takes on the special values
takes on the special values
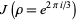 |
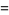 |
 |
(8) |
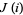 |
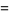 |
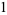 |
(9) |
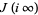 |
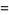 |
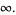 |
(10) |
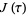 satisfies the functional equations
satisfies the functional equations
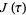 |
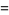 |
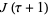 |
(11) |
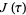 |
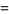 |
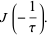 |
(12) |
It satisfies a number of beautiful multiple-argument identities, including the duplication formula
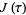 |
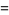 |
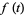 |
(13) |
 |
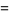 |
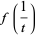 |
(14) |
with
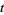 |
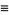 |
![1/(64)[(eta(tau))/(eta(2tau))]^(24)](http://mathworld.wolfram.com/images/equations/KleinsAbsoluteInvariant/Inline63.gif) |
(15) |
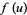 |
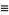 |
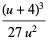 |
(16) |
and 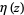 the Dedekind eta function, the triplication formula
the Dedekind eta function, the triplication formula
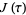 |
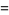 |
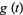 |
(17) |
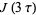 |
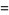 |
 |
(18) |
with
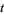 |
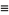 |
![1/(27)[(eta(tau))/(eta(3tau))]^(12)](http://mathworld.wolfram.com/images/equations/KleinsAbsoluteInvariant/Inline76.gif) |
(19) |
 |
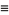 |
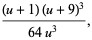 |
(20) |
and the quintuplication formula
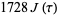 |
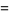 |
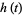 |
(21) |
 |
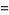 |
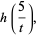 |
(22) |
with
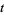 |
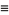 |
![1/5[(eta(tau))/(eta(5tau))]^6](http://mathworld.wolfram.com/images/equations/KleinsAbsoluteInvariant/Inline88.gif) |
(23) |
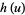 |
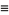 |
 |
(24) |
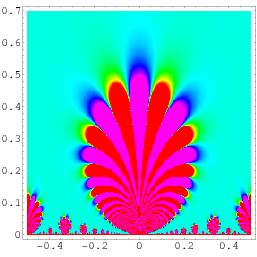
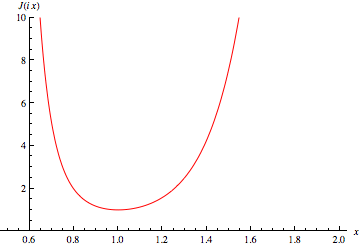
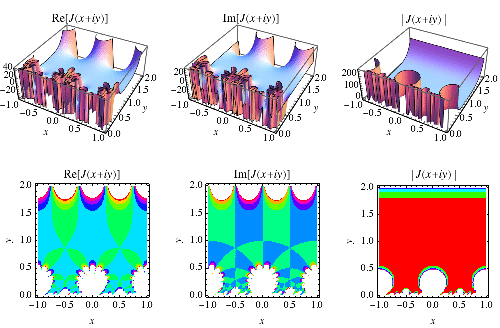
Plotting the real or imaginary part of 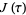 in the complex plane produces a beautiful fractal-like structure, illustrated above.
in the complex plane produces a beautiful fractal-like structure, illustrated above.
REFERENCES:
Apostol, T. M. "Klein's Modular Function  ," "Invariance of
," "Invariance of 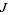 Under Unimodular Transformation," "The Fourier Expansions of
Under Unimodular Transformation," "The Fourier Expansions of  and
and 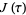 ," "Special Values of
," "Special Values of 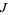 ," and "Modular Functions as Rational Functions of
," and "Modular Functions as Rational Functions of  ." §1.12-1.13, 1.15, and 2.5-2.6 in Modular Functions and Dirichlet Series in Number Theory, 2nd ed. New York: Springer-Verlag, pp. 15-18, 20-22, and 39-40, 1997.
." §1.12-1.13, 1.15, and 2.5-2.6 in Modular Functions and Dirichlet Series in Number Theory, 2nd ed. New York: Springer-Verlag, pp. 15-18, 20-22, and 39-40, 1997.
Brezhnev, Y. V. "Uniformisation: On the Burnside Curve 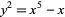 ." 9 Dec 2001. http://arxiv.org/abs/math.CA/0111150.
." 9 Dec 2001. http://arxiv.org/abs/math.CA/0111150.
Borwein, J. M. and Borwein, P. B. Pi & the AGM: A Study in Analytic Number Theory and Computational Complexity. New York: Wiley, pp. 115 and 179, 1987.
Cohn, H. Introduction to the Construction of Class Fields. New York: Dover, p. 73, 1994.
Klein, F. "Sull' equazioni dell' Icosaedro nella risoluzione delle equazioni del quinto grado [per funzioni ellittiche]." Reale Istituto Lombardo, Rendiconto, Ser. 2 10, 1877.
Klein, F. "Über die Transformation der elliptischen Funktionen und die Auflösung der Gleichungen fünften Grades." Math. Ann.14, 111-172, 1878-1879.
Nesterenko, Yu. V. A Course on Algebraic Independence: Lectures at IHP 1999. Unpublished manuscript. 1999.
 الاكثر قراءة في التفاضل و التكامل
الاكثر قراءة في التفاضل و التكامل
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة
قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)


















