ole

 The word "pole" is used prominently in a number of very different branches of mathematics. Perhaps the most important and widespread usage is to denote a singularity of a complex function. In inversive geometry, the inversion pole is related to inverse points with respect to an inversion circle. The term "pole" is also used to denote the degenerate points 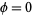 and 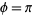 in spherical coordinates, corresponding to the north pole and south polerespectively. "All-poles method" is an alternate term for the maximum entropy method used in deconvolution. In triangle geometry, an orthopole is the point of concurrence certain perpendiculars with respect to a triangle of a given line, and a Simson line pole is similarly defined based on the Simson line of a point with respect to a triangle. In projective geometry, the perspector is sometimes known as the perspective pole.

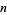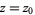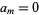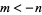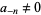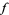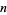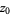In complex analysis, an analytic function  is said to have a pole of order  at a point  if, in the Laurent series,  for  and . Equivalently,  has a pole of order  at  if 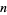 is the smallest positive integer for which  is holomorphic at . A analytic function  has a pole at infinity if

A nonconstant polynomial 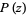 has a pole at infinity of order 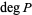, i.e., the polynomial degree of .

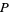The basic example of a pole is 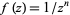, which has a single pole of order  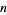at . Plots of  and 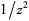 are shown above in the complex plane.

For a rational function, the poles are simply given by the roots of the denominator, where a root of multiplicity 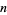corresponds to a pole of order .

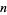A holomorphic function whose only singularities are poles is called a meromorphic function.

Renteln and Dundes (2005) give the following (bad) mathematical jokes about poles:

Q: What's the value of a contour integral around Western Europe? A: Zero, because all the Poles are in Eastern Europe.

Q: Why did the mathematician name his dog "Cauchy?" A: Because he left a residue at every pole.

REFERENCES:

Renteln, P. and Dundes, A. "Foolproof: A Sampling of Mathematical Folk Humor." Notices Amer. Math. Soc. 52, 24-34, 2005.