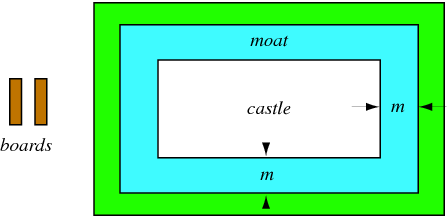
There are two versions of the moat-crossing problem, one geometric and one algebraic. The geometric moat problems asks for the widest moat Rapunzel can cross to escape if she has only two unit-length boards (and no means to nail or otherwise attach them together). More generally, what is the widest moat which can be crossed using 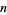 boards? Matthew Cook has conjectured that the asymptotic solution to this problem is  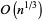(Cook 1997).

The algebraic moat-crossing problem asks if it is possible to walk to infinity on the real line using only steps of bounded lengths and steps on the prime numbers. The answer is negative (Gethner et al. 1998). However, the Gaussian moat problem that asks whether it is possible to walk to infinity in the Gaussian integers using the Gaussian primes as stepping stones and taking steps of bounded length is unresolved. Gethner et al. (1998) show that a moat of width 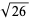 exists.

REFERENCES:

Cook, M. "Update on the Moat Crossing Problem." E-mail communication, 1997. http://www.mathcad.com/library/LibraryContent/puzzles/soln26/8b/update.html.

Finch, S. "Moat Crossing Optimization Problem." http://www.mathcad.com/library/LibraryContent/puzzles/soln26/soln26.html.

Gethner, E. and Stark, H. M. "Periodic Gaussian Moats." Experiment. Math. 6, 251-254, 1997.

Gethner, E.; Wagon, S.; and Wick, B. "A Stroll Through the Gaussian Primes." Amer. Math. Monthly 105, 327-337, 1998.

Guy, R. K. Unsolved Problems in Number Theory, 2nd ed. New York: Springer-Verlag, 1994.

Haugland, J. K. "A Walk on Complex Primes." [Norwegian.] Normat 43, 168-170, 1995.

Jordan, J. H. and Rabung, J. R. "A Conjecture of Paul Erdős Concerning Gaussian Primes." Math. Comput. 24, 221-223, 1970.

Montgomery, H. Ten Lectures on the Interface Between Analytic Number Theory and Harmonic Analysis. Providence, RI: Amer. Math. Soc., 1994.

Vardi, I. "Prime Percolation." Experiment. Math. 7, 275-289, 1998.

Wagon, S. Mathematica in Action, 2nd ed. New York: Springer-Verlag, 1999.

اخر الاخبار

اخبار العتبة العباسية المقدسة

قسم الشؤون الدينية يوزع أكثر من ألفي وجبة طعام للمعزين في ذكرى عاشوراء بنينوى

قسم الشؤون الطبية يواصل تقديم خدماته الصحية لزائري عاشوراء

جموع المعزين تحيي مراسم يوم العاشر من شهر المحرم عند المرقدين الطاهرين

قسم العلاقات يطلق محطات فتية الكفيل الثقافية في محافظة نينوى

المزيد

الآخبار الصحية

لهذا السبب.. إغلاق 25 مركزًا لعلاج الإدمان في مصر ؟

خلافا للاعتقاد الشائع.. علماء يكشفون أسرار بذور البطيخ ؟!

هذا ما يحدث لجسمك عند تناولك الطماطم بانتظام !

تعرف على أطعمة تسرّع "تعافي الجسم" من حروق الشمس

المزيد

الآخبار التكنلوجية

الثلوج تغطي الصحراء الأكثر جفافا في العالم!

بتلسكوب "ألما".. التقاط صورا غير مسبوقة لبدايات الكون !

هل هاتفك مراقب؟.. 5 علامات خطيرة تكشف التجسس عليك

دبي تطلق أول رحلة تجريبية للتاكسي الجوي

المزيد