At this stage we are not going to try to find the formula for the above increasing function of the temperature in terms of our familiar mercury temperature scale, but instead we shall define temperature by a new scale. At one time “the temperature” was defined arbitrarily by dividing the expansion of water into even degrees of a certain size. But when one then measures temperature with a mercury thermometer, one finds that the degrees are no longer even. But now we can make a definition of temperature which is independent of any particular substance. We can use that function f(T), which does not depend on what device we use, because the efficiency of these reversible engines is independent of their working substances. Since the function we found is rising with temperature, we will define the function itself as the temperature, measured in units of the standard one-degree temperature, as follows:

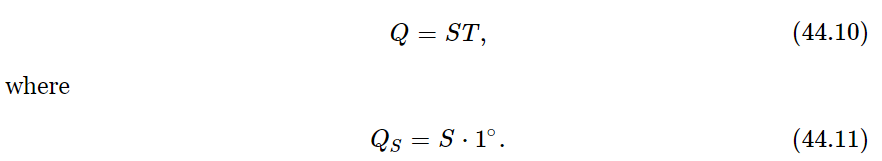
This means that we can tell how hot an object is by finding out how much heat is absorbed by a reversible engine working between the temperature of the object and the unit temperature (Fig. 44–9). If seven times more heat is taken out of a boiler than is delivered at a one-degree condenser, the temperature of the boiler will be called seven degrees, and so forth. So, by measuring how much heat is absorbed at different temperatures, we determine the temperature. The temperature defined in this way is called the absolute thermodynamic temperature, and it is independent of the substance. We shall use this definition exclusively from now on.¹

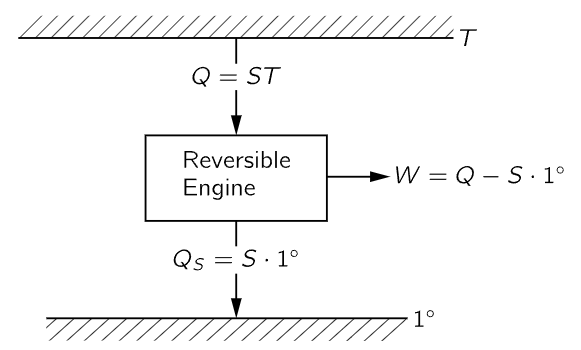
Fig. 44–9. Absolute thermodynamic temperature.

Now we see that when we have two engines, one working between T₁ and one degree, the other working between T₂ and one degree, delivering the same heat at unit temperature, then the heats absorbed must be related by

But that means that if we have a single engine running between T₁ and T₂, then the result of the whole analysis, the grand finale, is that Q₁ is to T₁ as Q₂ is to T₂, if the engine absorbs energy Q₁ at temperature T₁ and delivers heat Q₂ at temperature T₂. Whenever the engine is reversible, this relationship between the heats must follow. That is all there is to it: that is the center of the universe of thermodynamics.

If this is all there is to thermodynamics, why is it considered such a difficult subject? In doing a problem involving a given mass of some substance, the condition of the substance at any moment can be described by telling what its temperature is and what its volume is. If we know the temperature and volume of a substance, and that the pressure is some function of the temperature and volume, then we know the internal energy. One could say, “I do not want to do it that way. Tell me the temperature and the pressure, and I will tell you the volume. I can think of the volume as a function of temperature and pressure, and the internal energy as a function of temperature and pressure, and so on.” That is why thermodynamics is hard, because everyone uses a different approach. If we could only sit down once and decide on our variables, and stick to them, it would be fairly easy.

Now we start to make deductions. Just as F=ma is the center of the universe in mechanics, and it goes on and on and on after that, in the same way the principle just found is all there is to thermodynamics. But can one make conclusions out of it?

We begin. To obtain our first conclusion, we shall combine both laws, the law of conservation of energy and this law which relates the heats Q₂ and Q₁, and we can easily obtain the efficiency of a reversible engine. From the first law, we have W=Q₁−Q₂. According to our new principle,

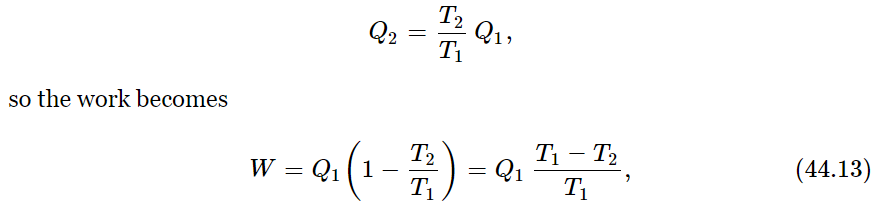
which tells us the efficiency of the engine—how much work we get out of so much heat. The efficiency of an engine is proportional to the difference in the temperatures between which the engine runs, divided by the higher temperature:

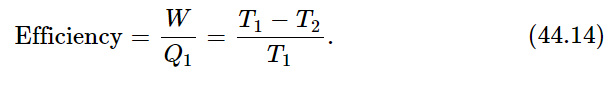
The efficiency cannot be greater than unity and the absolute temperature cannot be less than zero, absolute zero. So, since T₂ must be positive, the efficiency is always less than unity. That is our first conclusion.

________________________________________________________
Margin

1- We have previously defined our scale of temperature in a different way, namely by stating that the mean kinetic nergy of a molecule in a perfect gas is proportional to the temperature, or that the perfect gas law says pV is proportional to T. Is this new definition equivalent? Yes, since the final result (44.7) derived from the gas law is the same as that derived here.

مواضيع ذات صلة

الديناميكا الحرارية الجزيئية

توزيع بولتزمان

العلاقات بين الخواص

القانون الثالث للديناميكا الحرارية

الطاقة الحرة

القانون الثاني للديناميكا الحرارية

القانون الأول للديناميكا الحرارية

Order and entropy

Irreversibility

The Clausius-Clapeyron equation

Internal energy

Entropy