A generalized continued fraction is an expression of the form

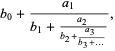(1)

where the partial numerators 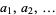 and partial denominators  may in general be integers, real numbers, complex numbers, or functions (Rockett and Szüsz, 1992, p. 1). Generalized continued fractions may also be written in the forms

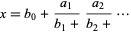(2)

or

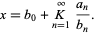(3)

Note that letters other than 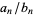 are sometimes also used; for example, the documentation for ContinuedFractionK[f, g, {" src="https://mathworld.wolfram.com/images/equations/GeneralizedContinuedFraction/Inline4.gif" style="height:15px; width:5px" />i, imin, imax}" src="https://mathworld.wolfram.com/images/equations/GeneralizedContinuedFraction/Inline5.gif" style="height:15px; width:5px" />] in the Wolfram Language uses .

Padé approximants provide another method of expanding functions, namely as a ratio of two power series. The quotient-difference algorithm allows interconversion of continued fraction, power series, and rational function approximations.

A small sample of closed-form continued fraction constants is given in the following table (cf. Euler 1775). The Ramanujan continued fractions provide another fascinating class of continued fraction constants, and the Rogers-Ramanujan continued fraction is an example of a convergent generalized continued fraction function where a simple definition leads to quite intricate structure.

continued fraction	value	approximate	OEIS
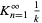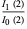		0.697774...	A052119
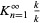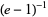		0.581976...	A073333
		1.525135...	A111129
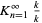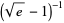		1.541494...	A113011

The value

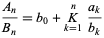(4)

is known as the 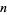th convergent of the continued fraction.

A regular continued fraction representation (which is usually what is meant when the term "continued fraction" is used without qualification) of a number  is one for which the partial quotients are all unity (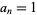),  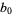is an integer, and 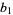, , 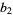... are positive integers (Rockett and Szüsz, 1992, p. 3).

Euler showed that if a convergent series can be written in the form

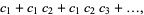(5)

then it is equal to the continued fraction

(6)

(Borwein et al. 2004, p. 30).

To "round" a regular continued fraction, truncate the last term unless it is 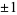, in which case it should be added to the previous term (Gosper 1972, Item 101A). To take one over a simple continued fraction, add (or possibly delete) an initial 0 term. To negate, take the negative of all terms, optionally using the identity

(7)

A particularly beautiful identity involving the terms of the continued fraction is

(8)

There are two possible representations for a finite simple fraction:

{[a_0,...,a_(n-1),a_n-1,1] for a_n>1; [a_0,...,a_(n-2),a_(n-1)+1] for a_n=1. " src="https://mathworld.wolfram.com/images/equations/GeneralizedContinuedFraction/NumberedEquation9.gif" style="height:41px; width:306px" />

(9)

REFERENCES:

Borwein, J.; Bailey, D.; and Girgensohn, R. Experimentation in Mathematics: Computational Paths to Discovery. Wellesley, MA: A K Peters, 2004.

Gosper, R. W. "Continued fractions query." math-fun@cs.arizona.edu posting, Dec. 27, 1996.

Gosper, R. W. Item 101a in Beeler, M.; Gosper, R. W.; and Schroeppel, R. HAKMEM. Cambridge, MA: MIT Artificial Intelligence Laboratory, Memo AIM-239, pp. 37-39, Feb. 1972. https://www.inwap.com/pdp10/hbaker/hakmem/cf.html#item101a.

Rockett, A. M. and Szüsz, P. Continued Fractions. New York: World Scientific, 1992.