

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Inverse Trigonometric Functions
المؤلف:
Abramowitz, M. and Stegun, I. A.
المصدر:
"Inverse Circular Functions." §4.4 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover
الجزء والصفحة:
...
12-10-2019
3727
The inverse trigonometric functions are the inverse functions of the trigonometric functions, written  ,
,  ,
, 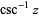 ,
,  ,
,  , and
, and 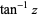 .
.
Alternate notations are sometimes used, as summarized in the following table.
 |
alternate notations |
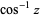 |
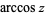 (Spanier and Oldham 1987, p. 333; Gradshteyn and Ryzhik 2000, p. 207) (Spanier and Oldham 1987, p. 333; Gradshteyn and Ryzhik 2000, p. 207) |
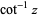 |
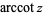 (Spanier and Oldham 1987, p. 333), (Spanier and Oldham 1987, p. 333),  (Spanier and Oldham 1987, p. 333; Gradshteyn and Ryzhik 2000, p. 208; Jeffrey 2000, p. 127) (Spanier and Oldham 1987, p. 333; Gradshteyn and Ryzhik 2000, p. 208; Jeffrey 2000, p. 127) |
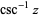 |
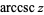 (Spanier and Oldham 1987, p. 333), (Spanier and Oldham 1987, p. 333), 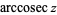 (Spanier and Oldham 1987, p. 333; Gradshteyn and Ryzhik 2000, p. 207) (Spanier and Oldham 1987, p. 333; Gradshteyn and Ryzhik 2000, p. 207) |
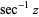 |
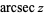 (Spanier and Oldham 1987, p. 333; Gradshteyn and Ryzhik 2000, p. 209) (Spanier and Oldham 1987, p. 333; Gradshteyn and Ryzhik 2000, p. 209) |
 |
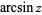 (Spanier and Oldham 1987, p. 333; Gradshteyn and Ryzhik 2000, p. 207) (Spanier and Oldham 1987, p. 333; Gradshteyn and Ryzhik 2000, p. 207) |
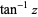 |
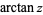 (Spanier and Oldham 1987, p. 333), (Spanier and Oldham 1987, p. 333), 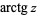 (Spanier and Oldham 1987, p. 333; Gradshteyn and Ryzhik 2000, p. 208; Jeffrey 2000, p. 127) (Spanier and Oldham 1987, p. 333; Gradshteyn and Ryzhik 2000, p. 208; Jeffrey 2000, p. 127) |
The inverse trigonometric functions are multivalued. For example, there are multiple values of 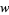 such that
such that 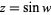 , so
, so  is not uniquely defined unless a principal value is defined. Such principal values are sometimes denoted with a capital letter so, for example, the principal value of the inverse sine
is not uniquely defined unless a principal value is defined. Such principal values are sometimes denoted with a capital letter so, for example, the principal value of the inverse sine 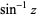 may be variously denoted
may be variously denoted 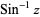 or
or  (Beyer 1987, p. 141). On the other hand, the notation
(Beyer 1987, p. 141). On the other hand, the notation 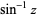 (etc.) is also commonly used denote either the principal value or any quantity whose sine is
(etc.) is also commonly used denote either the principal value or any quantity whose sine is  an (Zwillinger 1995, p. 466). Worse still, the principal value and multiply valued notations are sometimes reversed, with for example
an (Zwillinger 1995, p. 466). Worse still, the principal value and multiply valued notations are sometimes reversed, with for example 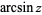 denoting the principal value and
denoting the principal value and 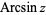 denoting the multivalued functions (Spanier and Oldham 1987, p. 333).
denoting the multivalued functions (Spanier and Oldham 1987, p. 333).
Since the inverse trigonometric functions are multivalued, they require branch cuts in the complex plane. Differing branch cut conventions are possible, but those adopted in this work follow those used by the Wolfram Language, summarized below.
| function name | function | Wolfram Language | branch cut(s) |
| inverse cosecant | 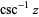 |
ArcCsc[z] | 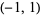 |
| inverse cosine | 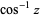 |
ArcCos[z] | 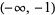 and and 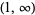 |
| inverse cotangent | 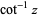 |
ArcCot[z] |  |
| inverse secant |  |
ArcSec[z] | 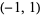 |
| inverse sine | 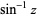 |
ArcSin[z] | 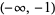 and and 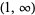 |
| inverse tangent | 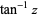 |
ArcTan[z] | ![(-iinfty,-i]](http://mathworld.wolfram.com/images/equations/InverseTrigonometricFunctions/Inline46.gif) and and 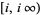 |
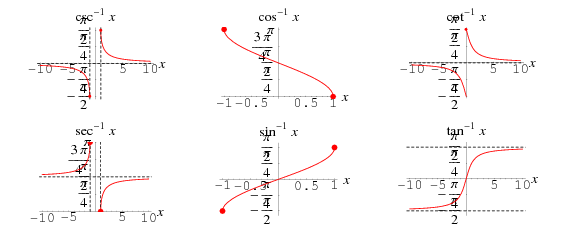
Different conventions are possible for the range of these functions for real arguments. Following the convention used by the Wolfram Language, the inverse trigonometric functions defined in this work have the following ranges for domains on the real line 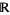 , illustrated above.
, illustrated above.
| function name | function | domain | range |
| inverse cosecant | 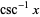 |
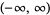 |
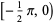 or or ![(0,1/2pi]](http://mathworld.wolfram.com/images/equations/InverseTrigonometricFunctions/Inline52.gif) |
| inverse cosine |  |
![[-1,1]](http://mathworld.wolfram.com/images/equations/InverseTrigonometricFunctions/Inline54.gif) |
![[0,pi]](http://mathworld.wolfram.com/images/equations/InverseTrigonometricFunctions/Inline55.gif) |
| inverse cotangent | 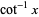 |
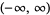 |
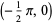 or or ![(0,1/2pi]](http://mathworld.wolfram.com/images/equations/InverseTrigonometricFunctions/Inline59.gif) |
| inverse secant | 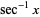 |
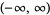 |
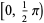 or or ![(1/2pi,pi]](http://mathworld.wolfram.com/images/equations/InverseTrigonometricFunctions/Inline63.gif) |
| inverse sine |  |
![[-1,1]](http://mathworld.wolfram.com/images/equations/InverseTrigonometricFunctions/Inline65.gif) |
![[-1/2pi,1/2pi]](http://mathworld.wolfram.com/images/equations/InverseTrigonometricFunctions/Inline66.gif) |
| inverse tangent | 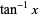 |
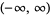 |
 |
Inverse-forward identities are
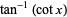 |
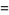 |
![1/2pi-x forx in [0,pi]](http://mathworld.wolfram.com/images/equations/InverseTrigonometricFunctions/Inline72.gif) |
(1) |
 |
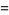 |
![1/2pi-x forx in [0,pi]](http://mathworld.wolfram.com/images/equations/InverseTrigonometricFunctions/Inline75.gif) |
(2) |
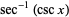 |
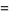 |
![1/2pi-x forx in [0,1/2pi].](http://mathworld.wolfram.com/images/equations/InverseTrigonometricFunctions/Inline78.gif) |
(3) |
Forward-inverse identities are
 |
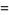 |
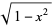 |
(4) |
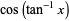 |
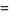 |
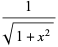 |
(5) |
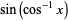 |
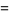 |
 |
(6) |
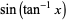 |
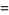 |
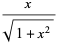 |
(7) |
 |
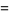 |
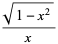 |
(8) |
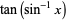 |
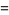 |
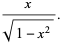 |
(9) |
Inverse sum identities include
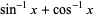 |
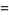 |
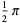 |
(10) |
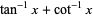 |
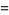 |
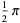 |
(11) |
 |
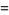 |
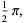 |
(12) |
where equation (11) is valid only for 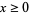 .
.
Complex inverse identities in terms of natural logarithms include
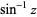 |
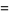 |
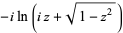 |
(13) |
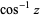 |
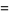 |
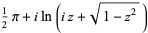 |
(14) |
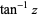 |
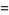 |
![1/2i[ln(1-iz)-ln(1+iz)].](http://mathworld.wolfram.com/images/equations/InverseTrigonometricFunctions/Inline115.gif) |
(15) |
REFERENCES:
Abramowitz, M. and Stegun, I. A.(Eds.). "Inverse Circular Functions." §4.4 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 79-83, 1972.
Apostol, T. M. "Inverses of the Trigonometric Functions." §6.21 in Calculus, 2nd ed., Vol. 1: One-Variable Calculus, with an Introduction to Linear Algebra. Waltham, MA: Blaisdell, pp. 253-256, 1967.
Beyer, W. H.(Ed.). CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, 1987.
Harris, J. W. and Stocker, H. "Inverse Trigonometric Functions." Handbook of Mathematics and Computational Science. New York: Springer-Verlag, pp. 306-318, 1998.
Jeffrey, A. "Inverse Trigonometric and Hyperbolic Functions." §2.7 in Handbook of Mathematical Formulas and Integrals, 2nd ed. Orlando, FL: Academic Press, pp. 124-128, 2000.
Spanier, J. and Oldham, K. B. "Inverse Trigonometric Functions." Ch. 35 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 331-341, 1987.
Trott, M. "Inverse Trigonometric and Hyperbolic Functions." §2.2.5 in The Mathematica GuideBook for Programming. New York: Springer-Verlag, pp. 180-191, 2004. http://www.mathematicaguidebooks.org/.
Zwillinger, D.(Ed.). CRC Standard Mathematical Tables and Formulae. Boca Raton, FL: CRC Press, 1995.