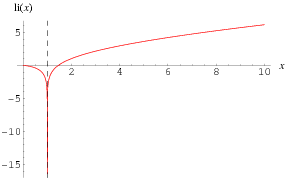
The logarithmic integral (in the "American" convention; Abramowitz and Stegun 1972; Edwards 2001, p. 26), is defined for real  as

		{int_0^x(dt)/(lnt) for 0<x<1; PVint_0^x(dt)/(lnt) for x>1" src="http://mathworld.wolfram.com/images/equations/LogarithmicIntegral/Inline4.gif" style="height:84px; width:158px" />	(1)
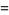		{int_0^x(dt)/(lnt) for 0<x<1; lim_(epsilon->0^+)[int_0^(1-epsilon)(dt)/(lnt)+int_(1+epsilon)^x(dt)/(lnt)] for x>1" src="http://mathworld.wolfram.com/images/equations/LogarithmicIntegral/Inline7.gif" style="height:84px; width:240px" />	(2)

Here, PV denotes Cauchy principal value of the integral, and the function has a singularity at .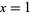

The logarithmic integral defined in this way is implemented in the Wolfram Language as LogIntegral[x].

There is a unique positive number

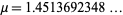(3)

(OEIS A070769; Derbyshire 2004, p. 114) known as Soldner's constant for which , so the logarithmic integral can also be written as

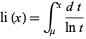(4)

for .

Special values include

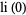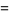			(5)
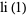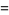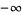			(6)
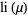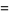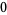			(7)
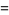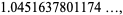			(8)

(OEIS A069284), where  is Soldner's constant (Edwards 2001, p. 34).

Min   Max       Re       Im

The definition can also be extended to the complex plane, as illustrated above.

Its derivative is

(9)

and its indefinite integral is

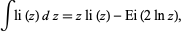(10)

where 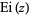 is the exponential integral. It also has the definite integral

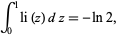(11)

where  (OEIS A002162) is the natural logarithm of 2.

The logarithmic integral obeys

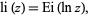(12)

where 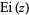 is the exponential integral, as well as the identity

(13)

(Bromwich and MacRobert 1991, p. 334; Hardy 1999, p. 25).

Nielsen showed and Ramanujan independently discovered that

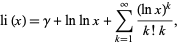(14)

where 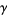 is the Euler-Mascheroni constant (Nielsen 1965, pp. 3 and 11; Berndt 1994; Finch 2003; Havil 2003, p. 106). Another formula due to Ramanujan which converges more rapidly is

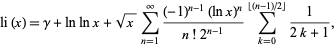(15)

where  is the floor function (Berndt 1994).

The form of this function appearing in the prime number theorem (used for example by Landau as well as Havil 2003, pp. 105 and 175) and sometimes referred to as the "European" definition (Derbyshire 2004, p. 373) is defined so that :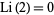

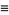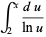			(16)
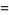			(17)

Note that the notation 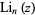 is (confusingly) also used for the polylogarithm and also for the "American" definition of  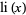(Edwards 2001, p. 26).

REFERENCES:

Abramowitz, M. and Stegun, I. A. (Eds.). Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, p. 879, 1972.

Berndt, B. C. Ramanujan's Notebooks, Part IV. New York: Springer-Verlag, pp. 126-131, 1994.

Bromwich, T. J. I'A. and MacRobert, T. M. An Introduction to the Theory of Infinite Series, 3rd ed. New York: Chelsea, p. 334, 1991.

de Morgan, A. The Differential and Integral Calculus, Containing Differentiation, Integration, Development, Series, Differential Equations, Differences, Summation, Equations of Differences, Calculus of Variations, Definite Integrals--With Applications to Algebra, Plane Geometry, Solid Geometry, and Mechanics. London: Robert Baldwin, p. 662, 1839.

Derbyshire, J. Prime Obsession: Bernhard Riemann and the Greatest Unsolved Problem in Mathematics. New York: Penguin, pp. 114-117 and 373, 2004.

Edwards, H. M. Riemann's Zeta Function. New York: Dover, 2001.

Finch, S. R. "Euler-Gompertz Constant." §6.2 in Mathematical Constants. Cambridge, England: Cambridge University Press, pp. 423-428, 2003.

Hardy, G. H. Ramanujan: Twelve Lectures on Subjects Suggested by His Life and Work, 3rd ed. New York: Chelsea, 1999.

Havil, J. Gamma: Exploring Euler's Constant. Princeton, NJ: Princeton University Press, pp. 105-106 and 175-176, 2003.

Koosis, P. The Logarithmic Integral I. Cambridge, England: Cambridge University Press, 1998.

Nielsen, N. "Theorie des Integrallograrithmus und Verwandter Transzendenten." Part II in Die Gammafunktion. New York: Chelsea, 1965.

Vardi, I. Computational Recreations in Mathematica. Reading, MA: Addison-Wesley, p. 151, 1991.

Hardy, G. H. Ramanujan: Twelve Lectures on Subjects Suggested by His Life and Work, 3rd ed. New York: Chelsea, p. 45, 1999.

Le Lionnais, F. Les nombres remarquables. Paris: Hermann, p. 39, 1983.

Sloane, N. J. A. Sequences A0021624074, A069284 and A070769 in "The On-Line Encyclopedia of Integer Sequences."

Soldner. Abhandlungen 2, 333, 1812.