1.1 Maxwell’s equations in (3 + 1)-form

we define a set of observers in the Kerr spacetime which have the four-velocity u^μ such that

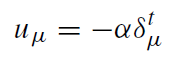 (1.1)

where α := (ΣΔ/A)^1/2 is the lapse function. Since u_μξ^μ_(φ)= 0, this family of observers has zero angular momentum. By means of the projector h_μν = g_μν + u_μuν we can obtain spatial vectors and tensors in the reference frame of the observer.

We introduce the following notation for the so defined electrodynamical quantities measured by locally non-rotating observers: E is the electric field strength, B is the magnetic field strength, ρ_e is the electric charge density, and j is the electric current density. Denote by ϖ the norm of a Killing vector ξ^μ_(φ) reflecting the axial symmetry of spacetime,

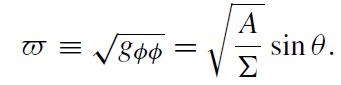 (1.2)

We denote by e_ǿ a three dimensional unit vector in the direction of the Killing vector ξ^μ_(φ). By using this notation, Maxwell's equations can be written in the following form:

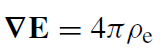 (1.3)

∇B = 0 (1.4)

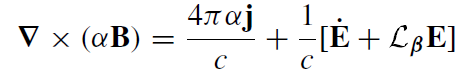 (1.5)

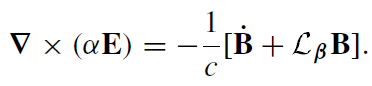 (1.6)

Here

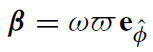 (1.7)

and

 (1.8)

is the angular velocity of rotation (with respect to t) of locally non-rotating observers. The notation ʆ_βE is used for the Lie derivative of a vector E along β

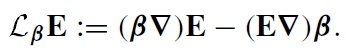 (1.9)

This Lie derivative describes how the vector E varies with respect to the field β. ʆβE vanishes when the origin and the end of the vector E are ‘glued’ under a displacement by β dφ. A dot denotes differentiation with respect to t and ∇ is the three dimensional (covariant) gradient operator in the curved ‘absolute’ space with metric h_μν .

The equations (1.3), (1.4) have a familiar form, whereas (1.5), (1.6) are slightly unusual. The following differences are evident. The function α appears because the physical time flows differently at different points of space while the equations are written in terms of the global ‘time’ t (recall that the acceleration of free fall, a, is related to α in the reference frame of locally nonrotating observers by the formula a = −c²∇ ln α). Furthermore, the expressions in brackets are ‘Lie-type’ derivatives (with respect to time) for the set of locally non-rotating observers who move in absolute space and for whom dx/dt = β.

Thus, these expressions correspond to total derivatives with respect to the times of E and B, respectively, with the motion of locally non-rotating observers taken into account.

1.2 Boundary conditions at the event horizon

The Rindler spacetime is a very good approximation in the narrow strip region near the event horizon of a black hole. For this reason, the previous results can be easily generalized to the case of a stationary black hole.

The event horizon is generated by null geodesics which are bicharacteristics of Maxwell's equations. The corresponding boundary conditions at the horizon can be written in a very clear form by introducing a fictitious surface electric charge density σ H which compensates for the flux of the electric field across the surface and a fictitious surface electric current i^H which closes tangent components of the magnetic fields at the horizon. This interpretation is used in the membrane formalism.

The horizon of a stationary black hole has topology T × S², and the surface with the topology S² is a two dimensional surface of infinite gravitational redshift, α = 0. The redshifted gravitational acceleration αa ≡ −c²αn ln α remains finite at the horizon:

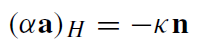 (1.10)

where n is a unit vector pointing orthogonally out of the horizon, and κ is the surface gravity. In the context of calculations near the horizon, it is convenient to introduce a coordinate system (α, λ, φ), where λ is a proper distance along the horizon from the north pole toward the equator. In these coordinates, the metric of the absolute three-space near the horizon takes the form

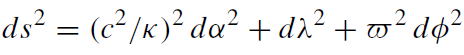 (1.11)

and the unit vectors along the ‘toroidal’ (φ), ‘poloidal’ (λ), and ‘normal’  (α) directions are

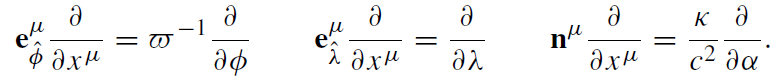 (1.12)

Macdonald and Thorne formulated the conditions at the horizon as follows:

In these relations the symbol→ indicates approach to the black hole horizon along the trajectory of a freely falling observer; (2)∇ is the two-dimensional divergence at the horizon, and B_|| and E_|| are the magnetic and electric field components tangent to the horizon. RH ≡ 4π/c is the effective surface resistance of the event horizon (RH = 377Ω). The lapse function α in the conditions reflects the slowdown in the flow of physical time for locally non-rotating observers in the neighborhood of the black hole.