

علم الكيمياء

تاريخ الكيمياء والعلماء المشاهير

التحاضير والتجارب الكيميائية

المخاطر والوقاية في الكيمياء

اخرى

مقالات متنوعة في علم الكيمياء

كيمياء عامة


الكيمياء التحليلية

مواضيع عامة في الكيمياء التحليلية

التحليل النوعي والكمي

التحليل الآلي (الطيفي)

طرق الفصل والتنقية


الكيمياء الحياتية

مواضيع عامة في الكيمياء الحياتية

الكاربوهيدرات

الاحماض الامينية والبروتينات

الانزيمات

الدهون

الاحماض النووية

الفيتامينات والمرافقات الانزيمية

الهرمونات


الكيمياء العضوية

مواضيع عامة في الكيمياء العضوية

الهايدروكاربونات

المركبات الوسطية وميكانيكيات التفاعلات العضوية

التشخيص العضوي

تجارب وتفاعلات في الكيمياء العضوية


الكيمياء الفيزيائية

مواضيع عامة في الكيمياء الفيزيائية

الكيمياء الحرارية

حركية التفاعلات الكيميائية

الكيمياء الكهربائية


الكيمياء اللاعضوية

مواضيع عامة في الكيمياء اللاعضوية

الجدول الدوري وخواص العناصر

نظريات التآصر الكيميائي

كيمياء العناصر الانتقالية ومركباتها المعقدة


مواضيع اخرى في الكيمياء

كيمياء النانو

الكيمياء السريرية

الكيمياء الطبية والدوائية

كيمياء الاغذية والنواتج الطبيعية

الكيمياء الجنائية


الكيمياء الصناعية

البترو كيمياويات

الكيمياء الخضراء

كيمياء البيئة

كيمياء البوليمرات

مواضيع عامة في الكيمياء الصناعية

الكيمياء الاشعاعية والنووية
Term symbols
المؤلف:
CATHERINE E. HOUSECROFT AND ALAN G. SHARPE
المصدر:
INORGANIC CHEMISTRY
الجزء والصفحة:
2th ed p 572
23-8-2016
7711
Term symbols
If we know (2S + 1), L and J for an energy state, we can write the full term symbol. This is done by writing the symbol of the value of L (i.e. S, P, D . . .) with the value of (2S + 1) as a left-superscript and the value of J as a rightsubscript. Thus, the electronic ground state of carbon is 3P0 (‘triplet P zero’) denoting L = 1,(2S+1) = 3 (i.e. S = 1) and J = 0. Different values of J denote different Term symbols. If we know (2S + 1), L and J for an energy state, we can write the full term symbol. This is done by writing the symbol of the value of L (i.e. S, P, D . . .) with the value of (2S + 1) as a left-superscript and the value of J as a rightsubscript. Thus, the electronic ground state of carbon is 3P0 (‘triplet P zero’) denoting L = 1, (2S + 1) = 3 (i.e. S = 1) and J = 0. Different values of J denote different levels within the term, i.e. (2S+1)LJ1 ,(2S+1)LJ2 . . . , the levels having different energies. Inorganic chemists often omit the value of J and refer to a (2S+1)L term; we shall usually follow this practice in this book. Now we look in detail at the electronic ground states of atoms with Z = 1 to 10.
Hydrogen (Z = 1)
A hydrogen atom has an electronic configuration of 1s1; for the electron, l = 0 so L must be 0 and, therefore, we have an S term. The total spin quantum number S = 1/2 so) 2S + 1) = 2 (a doublet term). The only possible value of J is 1/2, and so the term symbol for the hydrogen atom is 2S1/2.
Helium (Z = 2)
For helium (1s2), both electrons have l = 0, so L ¼ 0. Two electrons both with n = 1 and l = 0 must have ms = 1/2 and_1/2, so S = 0 and(2S + 1) = 1 (a singlet term). The only value of J is 0, and so the term symbol is 1S0. Thus, the ns2 configuration, having L = 0, S = 0 and J = 0, will contribute nothing to the term symbol in lithium and lateratoms. The same conclusion can be drawn for any np6 configuration and the reader is left to confirm this statement.
Lithium (Z = 3) and beryllium (Z = 4)
Atomic lithium has the electronic configuration 1s2 2s1, and its term symbol is the same as that for hydrogen, 2S1/2.
Similarly, the term symbol for beryllium (1s22s2) is the same as that for helium, 1S0.
Boron (Z = 5)
For boron (1s22s22p1) we need only consider the p electron for reasons outlined above. For this, l = 1 so L = 1 (a P term); S = 1/2 and so (2S +1( = 2 (a doublet term). J can take values) L S); (L + S _ 1) . . . |L _ S|, and so J = 3/2 or 1/2. The term symbol for boron may be 2P3/2 or 2P1/2.
Carbon (Z = 6)
For carbon (1s22s22p2), only the p electrons need be considered, and each has l = 1. Values of ml may be +1, 0 or _1, and the algebraic sum of ml for the individual electrons gives values of L = 2, 1 or 0 (D, P or S terms respectively). The two electrons may be spin-paired or have parallel spins and so S = 0 or 1, giving (2S + 1) = 1 (singlet term) or 3 (triplet term). It might seem that J could be 3, 2, 1 or 0, but this is not so. If, for example, the two electrons each have n = 2, l = 1 and ml = 1 (giving L = 2), they cannot both have ms = 1/2 as this would violate Pauli’s principle. The only allowed combinations of ml and ms (and corresponding values of ML and MS) for two p electrons with the same value of n are shown in the table; such combinations are called microstates. Table: Microstates for two electrons in an np level: values of ml and ms (represented as paired or unpaired electrons) and resultant values of ML , MS and MJ .

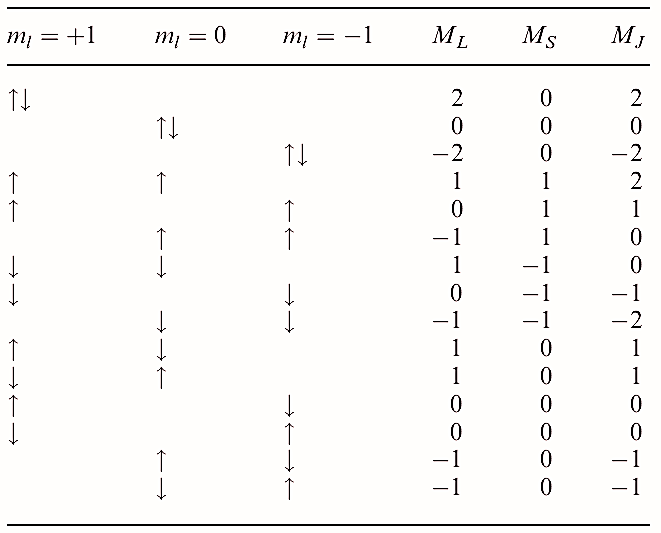
Inspection of the table reveals the following:
- the 15 microstates can be grouped into three sets, with the proviso that no set can contain a repetition;
- there is a set of five microstates with ML = 2, 1, 0, _1, _2 and MS = 0 (and thus MJ = 2, 1, 0, _1, _2) corresponding to L = 1 and a D term; moreover, since S = 0 (singlet) and J = 2, the term symbol is 1D2;
- there is a set of nine microstates with ML = 1, 0, _1 and MS = 1, 0, _1 which can be assigned the term 3P (because L = 1 and S = 1);
- further examination of this last set of microstates reveals that it can be subdivided into a set of five with
J = 2 (term symbol 3P2), a set of three with J = 1 (3P1), and a single entry with J = 0 (3P0);
- one entry in the table remains unaccounted for and has ML = 0, MS = 0 and MJ = 0, corresponding to the term 1S0.
We have, of course, no means of telling which entry with ML = 0 and MS = 0 should be assigned to which term (or similarly, how entries with ML = 1 and MS = 0, or ML = _1 and MS = 0 should be assigned). Indeed, it is not meaningful to do so. Of the five terms that we have denoted for carbon (1D2, 3P2, 3P1, 3P0 and 1S0), the one with the lowest energy is 3P0 and this is the electronic ground state. The others are excited states; notice that Hund’s rules do not always apply to excited states.
Nitrogen to neon (Z = 7_10)
A similar treatment for the nitrogen atom shows that the 2p3 configuration gives rise to 4S, 2P and 2D terms. For the 2p4 configuration (oxygen), we introduce a useful simplification by considering it as 2p6 plus two positrons which annihilate two of the electrons. Since positrons differ from electrons only in charge, the terms arising from the np4 and np2 configurations are the same. Similarly, np5 is equivalent to np1. This positron or positive hole concept is very useful and can be extended to nd configurations. Relative energies of terms and levels. In regard to relative energies of terms, we state all of Hund’s rules in a formal way. It is found from analysis of spectroscopic data that, provided that Russell–Saunders coupling holds:
- the term having the highest spin multiplicity (highest value of S) is the most stable (lowest energy);
- if two or more terms have the same value of S, the term having the higher value of L is the more stable;
- for all terms having the same values of S and L, the level with the lowest value of J is the most stable if the sub-shell is less than half-filled, and the level with the highest value of J is most stable if the sub-shell is more than half-filled (if the sub-shell is half-filled and S has the highest possible value, L = 0 and J =S).
Thus for the terms corresponding to the electronic configuration np2 (1D2, 3P2, 3P1, 3P0 and 1S0, see above), that of lowest energy is 3P and the level of lowest energy is 3P0.
 الاكثر قراءة في مواضيع عامة في الكيمياء اللاعضوية
الاكثر قراءة في مواضيع عامة في الكيمياء اللاعضوية
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام) قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)
قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)


















