

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Descriptive Statistics_Benford,s Law
المؤلف:
Benford, F.
المصدر:
"The Law of Anomalous Numbers." Proc. Amer. Phil. Soc. 78
الجزء والصفحة:
...
4-2-2021
1942
Descriptive Statistics_Benford's Law
A phenomenological law also called the first digit law, first digit phenomenon, or leading digit phenomenon. Benford's law states that in listings, tables of statistics, etc., the digit 1 tends to occur with probability 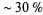 , much greater than the expected 11.1% (i.e., one digit out of 9). Benford's law can be observed, for instance, by examining tables of logarithms and noting that the first pages are much more worn and smudged than later pages (Newcomb 1881). While Benford's law unquestionably applies to many situations in the real world, a satisfactory explanation has been given only recently through the work of Hill (1998).
, much greater than the expected 11.1% (i.e., one digit out of 9). Benford's law can be observed, for instance, by examining tables of logarithms and noting that the first pages are much more worn and smudged than later pages (Newcomb 1881). While Benford's law unquestionably applies to many situations in the real world, a satisfactory explanation has been given only recently through the work of Hill (1998).
Benford's law was used by the character Charlie Eppes as an analogy to help solve a series of high burglaries in the Season 2 "The Running Man" episode (2006) of the television crime drama NUMB3RS.
Benford's law applies to data that are not dimensionless, so the numerical values of the data depend on the units. If there exists a universal probability distribution  over such numbers, then it must be invariant under a change of scale, so
over such numbers, then it must be invariant under a change of scale, so
 |
(1) |
If  , then
, then  , and normalization implies
, and normalization implies  . Differentiating with respect to
. Differentiating with respect to  and setting
and setting  gives
gives
 |
(2) |
having solution  . Although this is not a proper probability distribution (since it diverges), both the laws of physics and human convention impose cutoffs. For example, randomly selected street addresses obey something close to Benford's law.
. Although this is not a proper probability distribution (since it diverges), both the laws of physics and human convention impose cutoffs. For example, randomly selected street addresses obey something close to Benford's law.

If many powers of 10 lie between the cutoffs, then the probability that the first (decimal) digit is  is given by a logarithmic distribution
is given by a logarithmic distribution
 |
(3) |
for  , ..., 9, illustrated above and tabulated below.
, ..., 9, illustrated above and tabulated below.
 |
 |
 |
 |
| 1 | 0.30103 | 6 | 0.0669468 |
| 2 | 0.176091 | 7 | 0.0579919 |
| 3 | 0.124939 | 8 | 0.0511525 |
| 4 | 0.09691 | 9 | 0.0457575 |
| 5 | 0.0791812 |
However, Benford's law applies not only to scale-invariant data, but also to numbers chosen from a variety of different sources. Explaining this fact requires a more rigorous investigation of central limit-like theorems for the mantissas of random variables under multiplication. As the number of variables increases, the density function approaches that of the above logarithmic distribution. Hill (1998) rigorously demonstrated that the "distribution of distributions" given by random samples taken from a variety of different distributions is, in fact, Benford's law (Matthews).
One striking example of Benford's law is given by the 54 million real constants in Plouffe's "Inverse Symbolic Calculator" database, 30% of which begin with the digit 1. Taking data from several disparate sources, the table below shows the distribution of first digits as compiled by Benford (1938) in his original paper.
| col. | title | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | samples |
| A | Rivers, Area | 31.0 | 16.4 | 10.7 | 11.3 | 7.2 | 8.6 | 5.5 | 4.2 | 5.1 | 335 |
| B | Population | 33.9 | 20.4 | 14.2 | 8.1 | 7.2 | 6.2 | 4.1 | 3.7 | 2.2 | 3259 |
| C | Constants | 41.3 | 14.4 | 4.8 | 8.6 | 10.6 | 5.8 | 1.0 | 2.9 | 10.6 | 104 |
| D | Newspapers | 30.0 | 18.0 | 12.0 | 10.0 | 8.0 | 6.0 | 6.0 | 5.0 | 5.0 | 100 |
| E | Specific Heat | 24.0 | 18.4 | 16.2 | 14.6 | 10.6 | 4.1 | 3.2 | 4.8 | 4.1 | 1389 |
| F | Pressure | 29.6 | 18.3 | 12.8 | 9.8 | 8.3 | 6.4 | 5.7 | 4.4 | 4.7 | 703 |
| G | H.P. Lost | 30.0 | 18.4 | 11.9 | 10.8 | 8.1 | 7.0 | 5.1 | 5.1 | 3.6 | 690 |
| H | Mol. Wgt. | 26.7 | 25.2 | 15.4 | 10.8 | 6.7 | 5.1 | 4.1 | 2.8 | 3.2 | 1800 |
| I | Drainage | 27.1 | 23.9 | 13.8 | 12.6 | 8.2 | 5.0 | 5.0 | 2.5 | 1.9 | 159 |
| J | Atomic Wgt. | 47.2 | 18.7 | 5.5 | 4.4 | 6.6 | 4.4 | 3.3 | 4.4 | 5.5 | 91 |
| K |  , ,  |
25.7 | 20.3 | 9.7 | 6.8 | 6.6 | 6.8 | 7.2 | 8.0 | 8.9 | 5000 |
| L | Design | 26.8 | 14.8 | 14.3 | 7.5 | 8.3 | 8.4 | 7.0 | 7.3 | 5.6 | 560 |
| M | Reader's Digest | 33.4 | 18.5 | 12.4 | 7.5 | 7.1 | 6.5 | 5.5 | 4.9 | 4.2 | 308 |
| N | Cost Data | 32.4 | 18.8 | 10.1 | 10.1 | 9.8 | 5.5 | 4.7 | 5.5 | 3.1 | 741 |
| O | X-Ray Volts | 27.9 | 17.5 | 14.4 | 9.0 | 8.1 | 7.4 | 5.1 | 5.8 | 4.8 | 707 |
| P | Am. League | 32.7 | 17.6 | 12.6 | 9.8 | 7.4 | 6.4 | 4.9 | 5.6 | 3.0 | 1458 |
| Q | Blackbody | 31.0 | 17.3 | 14.1 | 8.7 | 6.6 | 7.0 | 5.2 | 4.7 | 5.4 | 1165 |
| R | Addresses | 28.9 | 19.2 | 12.6 | 8.8 | 8.5 | 6.4 | 5.6 | 5.0 | 5.0 | 342 |
| S |  , ,  |
25.3 | 16.0 | 12.0 | 10.0 | 8.5 | 8.8 | 6.8 | 7.1 | 5.5 | 900 |
| T | Death Rate | 27.0 | 18.6 | 15.7 | 9.4 | 6.7 | 6.5 | 7.2 | 4.8 | 4.1 | 418 |
| Average | 30.6 | 18.5 | 12.4 | 9.4 | 8.0 | 6.4 | 5.1 | 4.9 | 4.7 | 1011 | |
| Probable Error |  |
 |
 |
 |
 |
 |
 |
 |
The following table gives the distribution of the first digit of the mantissa following Benford's Law using a number of different methods.
| method | OEIS | sequence |
| Sainte-Lague | A055439 | 1, 2, 3, 1, 4, 5, 6, 1, 2, 7, 8, 9, ... |
| d'Hondt | A055440 | 1, 2, 1, 3, 1, 4, 2, 5, 1, 6, 3, 1, ... |
| largest remainder, Hare quotas | A055441 | 1, 2, 3, 4, 1, 5, 6, 7, 1, 2, 8, 1, ... |
| largest remainder, Droop quotas | A055442 | 1, 2, 3, 1, 4, 5, 6, 1, 2, 7, 8, 1, ... |
REFERENCES:
Barlow, J. L. and Bareiss, E. H. "On Roundoff Error Distributions in Floating Point and Logarithmic Arithmetic." Computing 34, 325-347, 1985.
Benford, F. "The Law of Anomalous Numbers." Proc. Amer. Phil. Soc. 78, 551-572, 1938.
Bogomolny, A. "Benford's Law and Zipf's Law." http://www.cut-the-knot.org/do_you_know/zipfLaw.shtml.
Boyle, J. "An Application of Fourier Series to the Most Significant Digit Problem." Amer. Math. Monthly 101, 879-886, 1994.
Flehinger, B. J. "On the Probability that a Random Integer Has Initial Digit 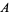 ." Amer. Math. Monthly 73, 1056-1061, 1966.
." Amer. Math. Monthly 73, 1056-1061, 1966.
Franel, J. Naturforschende Gesellschaft, Vierteljahrsschrift (Zürich) 62, 286-295, 1917.
Havil, J. "Benford's Law." §14.2 in Gamma: Exploring Euler's Constant. Princeton, NJ: Princeton University Press, pp. 145-155, 2003.
Hill, T. P. "Base-Invariance Implies Benford's Law." Proc. Amer. Math. Soc. 12, 887-895, 1995a.
Hill, T. P. "The Significant-Digit Phenomenon." Amer. Math. Monthly 102, 322-327, 1995b.
Hill, T. P. "A Statistical Derivation of the Significant-Digit Law." Stat. Sci. 10, 354-363, 1995c.
Hill, T. P. "The First Digit Phenomenon." Amer. Sci. 86, 358-363, 1998.
Knuth, D. E. "The Fraction Parts." §4.2.4B in The Art of Computer Programming, Vol. 2: Seminumerical Algorithms, 3rd ed. Reading, MA: Addison-Wesley, pp. 254-262, 1998.
Ley, E. "On the Peculiar Distribution of the U.S. Stock Indices Digits." Amer. Stat. 50, 311-313, 1996.
Livio, M. The Golden Ratio: The Story of Phi, the World's Most Astonishing Number. New York: Broadway Books, pp. 232-236, 2002.
Matthews, R. "The Power of One." http://www.fortunecity.com/emachines/e11/86/one.html.
Newcomb, S. "Note on the Frequency of the Use of Digits in Natural Numbers." Amer. J. Math. 4, 39-40, 1881.
Nigrini, M. J. The Detection of Income Tax Evasion Through an Analysis of Digital Frequencies. Ph.D. thesis. Cincinnati, OH: University of Cincinnati, 1992.
Nigrini, M. "A Taxpayer Compliance Application of Benford's Law." J. Amer. Tax. Assoc. 18, 72-91, 1996.
Nigrini, M. "I've Got Your Number." J. Accountancy 187, pp. 79-83, May 1999. http://www.aicpa.org/pubs/jofa/may1999/nigrini.htm.
Nigrini, M. Digital Analysis Using Benford's Law: Tests Statistics for Auditors. Vancouver, Canada: Global Audit Publications, 2000.
Plouffe, S. "Graph of the Number of Entries in Plouffe's Inverter." http://www.lacim.uqam.ca/~plouffe/statistics.html.
Raimi, R. A. "The Peculiar Distribution of First Digits." Sci. Amer. 221, 109-119, Dec. 1969.
Raimi, R. A. "On the Distribution of First Significant Digits." Amer. Math. Monthly 76, 342-348, 1969.
Raimi, R. A. "The First Digit Phenomenon." Amer. Math. Monthly 83, 521-538, 1976.
Schatte, P. "Zur Verteilung der Mantisse in der Gleitkommadarstellung einer Zufallsgröße." Z. Angew. Math. Mech. 53, 553-565, 1973.
Schatte, P. "On Mantissa Distributions in Computing and Benford's Law." J. Inform. Process. Cybernet. 24, 443-455, 1988.
Sloane, N. J. A. Sequences A055439, A055440, A055441, and A055442 in "The On-Line Encyclopedia of Integer Sequences."
 الاكثر قراءة في الاحتمالات و الاحصاء
الاكثر قراءة في الاحتمالات و الاحصاء
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام) قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)
قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)


















