Suppose that we have planar surface of scalar area S. We can define a vector area S whose magnitude is S and whose direction is perpendicular to the plane, in the sense determined by the right-hand grip rule on the rim. This quantity

clearly possesses both magnitude and direction. But is it a true vector? We know that if the normal to the surface makes an angle α_x with the x-axis then the area seen in the x-direction is S cos α_x. This is the x-component of S. Similarly, if the normal makes an angle α_y with the y-axis then the area seen in the y-direction is S cos α_y. This is the y-component of S. If we limit ourselves to a surface whose normal is perpendicular to the z-direction then α_x = π/2 - α_y = α. It follows that S = S(cos α, sin α, 0). If we rotate the basis about the z-axis by θ degrees, which is equivalent to rotating the normal to the surface about the z-axis by –θ degrees, then

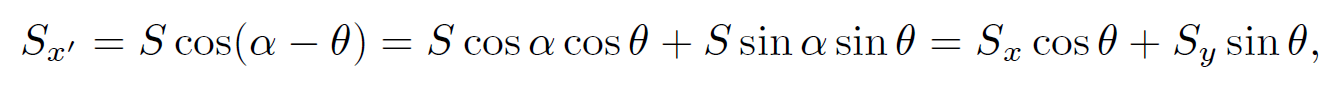  (1.1)

which is the correct transformation rule for the x-component of a vector. The other components transform correctly as well. This proves that a vector area is a true vector. According to the vector addition theorem the projected area of two plane surfaces, joined together at a line, in the x direction (say) is the x-component of the sum of the vector areas. Likewise, for many joined up plane areas the projected area in the x-direction, which is the same as the projected area of the rim in the x-direction, is the x-component of the resultant of all the vector areas:

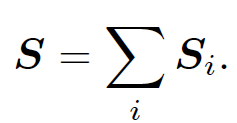 (1.2)

If we approach a limit, by letting the number of plane facets increase and their area reduce, then we obtain a continuous surface denoted by the resultant vector area:

 (1.3)

It is clear that the projected area of the rim in the x-direction is just S_x. Note that the rim of the surface determines the vector area rather than the nature of the surface. So, two different surfaces sharing the same rim both possess the same vector areas. In conclusion, a loop (not all in one plane) has a vector area S which is the resultant of the vector areas of any surface ending on the loop. The components of S are the projected areas of the loop in the directions of the basis vectors. As a corollary, a closed surface has S = 0 since it does not possess a rim.

مواضيع ذات صلة

القاعدة الأساسية لدفع الصاروخ

ثورة هايزنبرج: من يقين العلم إلى مسؤولية العلماء

الفزياء والكون .. تجربة ميكسلون ومورلي

الضوء

البلازما والفضاء

الكون المتحرك

الفيزياء والكون .. البلازما

الفيزياء والكون.. الذرة

قانون التوازن في كون الله الواسع

الفيزياء والكون جزيء الفا

وصف معجل «البيليترون »

دور الجدران المادية في حصر المادة البلازمية