Ohm's law

We all know the simplest version of Ohm's law:

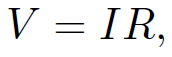 (1.1)

where V is the voltage drop across a resistor of resistance R when a current I flows through it. Let us generalize this law so that it is expressed in terms of E and j rather than V and I. Consider a length l of a conductor of uniform cross-sectional area A with a current I flowing down it. In general, we expect the electrical resistance of the conductor to be proportional to its length and inversely proportional to its area (i.e., it is harder to push an electrical current down a long rather than a short wire, and it is easier to push a current down a wide rather than a narrow conducting channel.) Thus, we can write

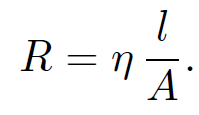 (1.2)

The constant ƞ is called the resistivity and is measured in units of ohm-meters. Ohm's law becomes

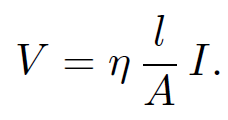 (1.3)

However, I/A = j_z (suppose that the conductor is aligned along the z-axis) and V/l = E_z, so the above equation reduces to

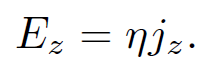 (1.4)

There is nothing special about the z-axis (in an isotropic conducting medium) so the previous formula immediately generalizes to

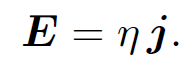 (1.5)

This is the vector form of Ohm's law. A charge q which moves through a voltage drop V acquires an energy qV from the electric field. In a resistor this energy is dissipated as heat. This type of heating is called ''ohmic heating". Suppose that N charges per unit time pass through a resistor. The current flowing is obviously I = Nq. The total energy gained by the charges, which appears as heat inside the resistor, is

 (1.6)

per unit time. Thus, the heating power is

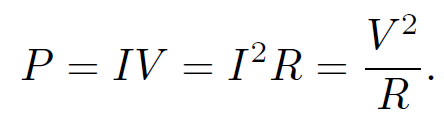 (1.7)

Equations (1.6) and (1.7) generalize to

 (1.8)

where P is now the power dissipated per unit volume in a resistive medium.

مواضيع ذات صلة

Thermal conductivity

Molecular diffusion

Ionic conductivity

The drift speed

Electrical transients

الشرر المقدس

الحقول الكهربية والشرر

الخلق غير المرئي

عجائب الكهرباء

مصادر الطاقة الكهربائية

التيارات الكهربائية التي تجري إلى الابد

النقل الكهربائي وقانون أوم