Probabilities can sometimes be surprising. We shall discuss two examples.

Birthday Probabilities

Suppose there are 30 people in a room. What is the probability that two of them share the same birthday (day and month)? (For simplicity, we shall ignore leap years.) Most people would say at first that the probability is small, but this is wrong.

First, list the 30 peoples’ birthdays in the order of their names (alphabetical order). If there is no restriction, each person has 365 possible birthdays. So there are 365³⁰ possibilities.

Suppose no two of them have the same birthday. There are 365 choices for the first person’s birthday, 364 for the second, 363 for the third, and so on. With no repeats the total number of possible lists of birthdays is

                                365×364×...×336.

Therefore the probability of the event “no two have the same birthday” is

                        365×364×...×336/365³⁰

which is approximately .294. So the probability of the “birthday coincidence” is about

                              1−.294 = .706,

or approximately 70%.

The Game Show

Our second example was made popular by columnist Marilyn Vos Savant.

Consider a TV game show: the contestant chooses between three doors marked doors 1, 2, and 3. Behind one door is a new car; behind each other is something almost worthless (a week’s supply of Kleenex tissues; two movie tickets; a pet goat)—call it a booby prize. The contestant chooses a door, and she gets the prize behind it.

But wait! After the choice is announced but before door is opened, the host opens one of the other two doors (not the one the contestant chose). Behind the door we see a goat. The host then asks, “Do you want to stay with your original choice? Or would you rather switch to the third door?”

Well, should the contestant stay or switch? Or doesn’t it matter? Most people would say it doesn’t matter.

To analyze the problem, we need to agree on a few things.

1. On any given night the chance that the car is behind any particular door is one in three.

2. The host always opens a door to show a goat, then offers the switch.

3. If the contestant’s first choice is the door with the car, there is an equal chance that the host will open either of the other two doors. He doesn’t open the lowernumbered one more often, or anything like that.

4. The game is always played the same way (the host never skips the “open another door” part).

For ease of analysis, suppose the doors are numbered 1, 2, and 3. The contestant chooses door 1. We shall write C1 to mean “the car is behind door 1,” C2 for “car behind door 2,” and C3 for “car behind door 3.” Similarly, H2 means “host opens door 2”, and H3 means “host opens door 3”. (He will not ever open door 1.)

Now suppose the game is played 600 times. We expect the car to behind each door in 200 cases. If the car is behind door 1, then we expect the host will open door 2 in 100 cases and open door 3 in 100 cases. In the 200 cases where the car is behind door 2, he always opens door 3; in the 200 cases where the car is behind door 3, he always opens door 2. So we can represent the data by

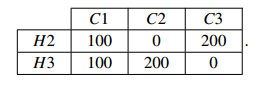
Now suppose the host opens door 2. This tells us that tonight is one of the 300 cases represented in the row H2. Of those 300 cases, the car is behind door 1 in 100 cases and behind door 3 in 200 cases. So the odds in favor of switching are 2 to 1.

The same reasoning applies when he opens door 3. So it is always best to switch.

مواضيع ذات صلة

Trial

Sampling

Sample Proportion

Sample

Poisson Trials

Experiment

Wiener Numbers

Time Series Analysis

Spencer,s Formula

Redundancy

Predictability

Moving Average